The Characteristic Time Scale of Cultural Evolution

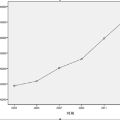
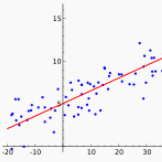
Numerous researchers from various disciplines have explored commonalities and divergences in the evolution of complex social formations. Here, we explore whether there is a characteristic time-course for the evolution of social complexity in a handful of different geographic areas. Time series data from the Seshat Global History Databank is shifted so that the overlapping time series can be fitted to a single logistic regression model for all 18 geographic areas under consideration. To analyse the endogenous growth of social complexity, each time series is restricted to a central time interval without discontinuous polity changes. The resulting regression shows convincing out-of-sample predictions and its period of rapidly growing social complexity can be identified via bootstrapping as a time interval of roughly 800 years.