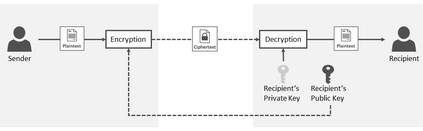
In this work, we present an efficient method for computing in the generalized Jacobian of special singular curves, nodal curves. The efficiency of the operation is due to the representation of an element in the Jacobian group by a single polynomial. In addition, we propose a probabilistic public key algorithm as an application of nodal curves.
翻译：在这项工作中,我们提出了一种高效的计算方法,在通用的雅各格特单曲线、节点曲线中进行计算,操作的效率是由于在雅各克组中用单一的多义表示一个元素。此外,我们建议采用概率公用钥匙算法作为节点曲线的应用。
相关内容
Arxiv
0+阅读 · 2022年7月31日
Arxiv
0+阅读 · 2022年7月29日
Arxiv
0+阅读 · 2022年7月28日
Arxiv
77+阅读 · 2019年11月10日
相关VIP内容
相关资讯
相关论文
Arxiv
0+阅读 · 2022年7月31日
Arxiv
0+阅读 · 2022年7月29日
Arxiv
0+阅读 · 2022年7月28日
Arxiv
77+阅读 · 2019年11月10日
微信扫码咨询专知VIP会员