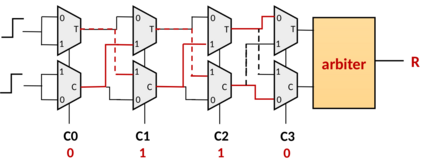
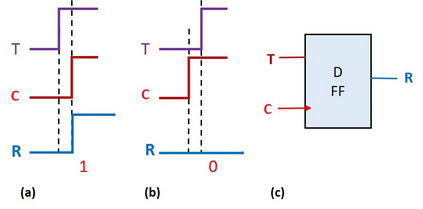
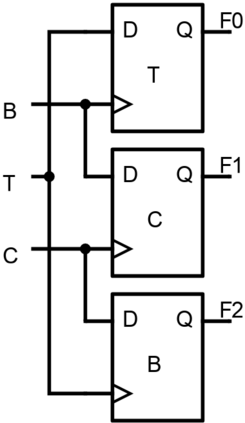
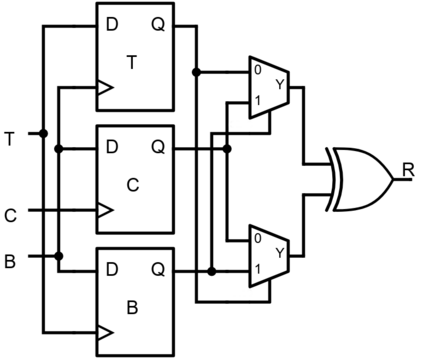
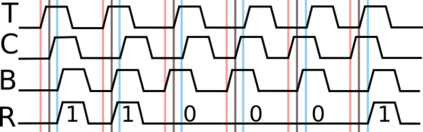
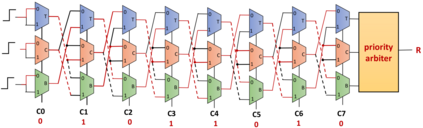
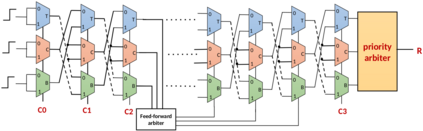
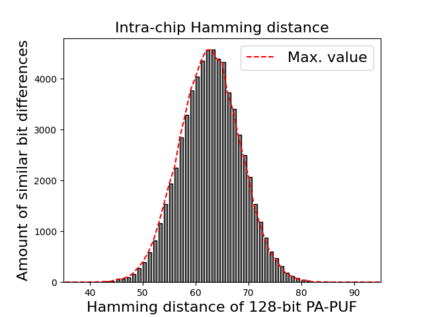
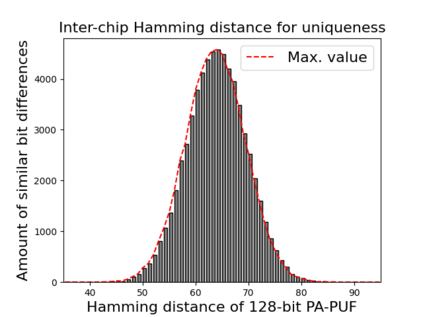
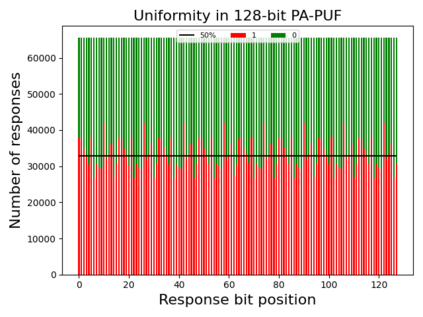
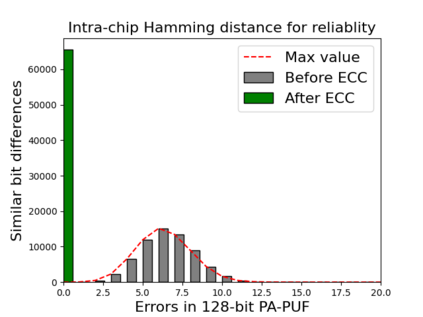
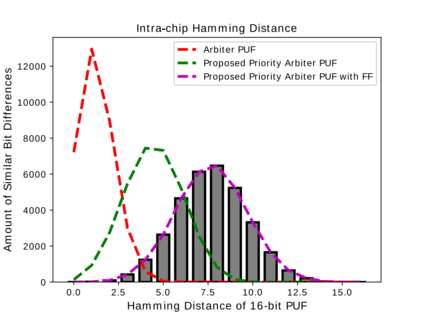
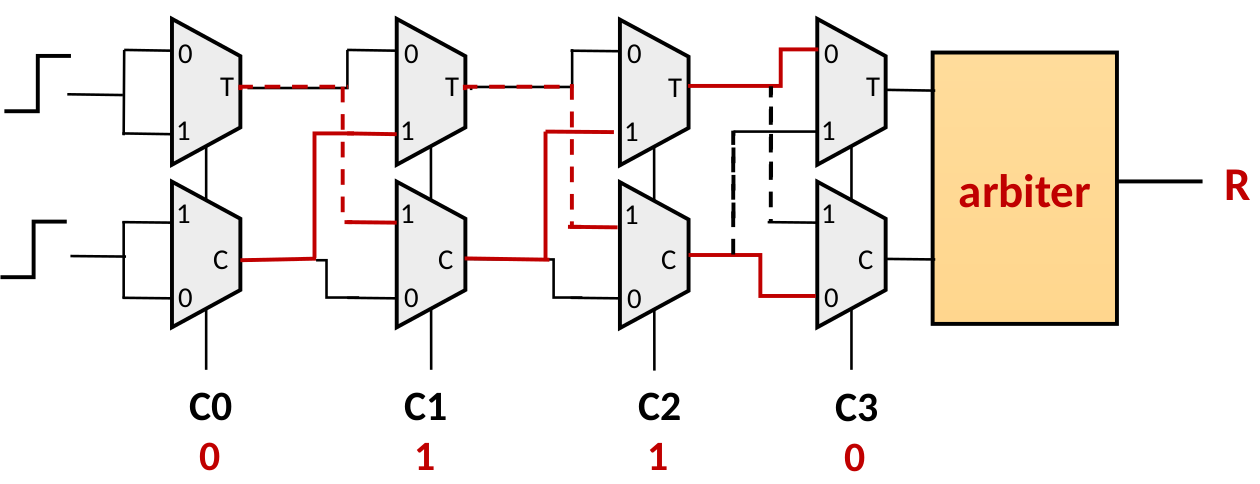
This paper proposes a 3-input arbiter-based novel physically unclonable function (PUF) design. Firstly, a 3-input priority arbiter is designed using a simple arbiter, two multiplexers (2:1), and an XOR logic gate. The priority arbiter has an equal probability of 0's and 1's at the output, which results in excellent uniformity (49.45%) while retrieving the PUF response. Secondly, a new PUF design based on priority arbiter PUF (PA-PUF) is presented. The PA-PUF design is evaluated for uniqueness, non-linearity, and uniformity against the standard tests. The proposed PA-PUF design is configurable in challenge-response pairs through an arbitrary number of feed-forward priority arbiters introduced to the design. We demonstrate, through extensive experiments, reliability of 100% after performing the error correction techniques and uniqueness of 49.63%. Finally, the design is compared with the literature to evaluate its implementation efficiency, where it is clearly found to be superior compared to the state-of-the-art.
翻译:本文提出了一个基于3个投入的仲裁人、基于3个投入的新的物理无法调和功能(PUF)设计。首先,一个基于3个投入的优先仲裁人的设计是使用简单的仲裁人、两个多路转换人(2:1)和一个 XOR 逻辑门。优先仲裁人在输出时的概率为0和1,这在输出时的概率是相等的,在检索PUF的响应时,结果非常统一(49.45 % ) 。第二,介绍了基于优先仲裁人PUF(PA-PUF)的新的PUF设计。对PA-PUF设计进行了与标准测试的独一性、非线性和统一性评价。拟议的PA-PUF设计通过向设计引入的任意数量的供餐前优先仲裁人可以配置在挑战对应配对中。我们通过广泛的实验,在进行错误纠正技术和49.63%的独特性之后证明100%的可靠性。最后,设计与评价其实施效率的文献进行了比较,在其中发现它明显优于状态。