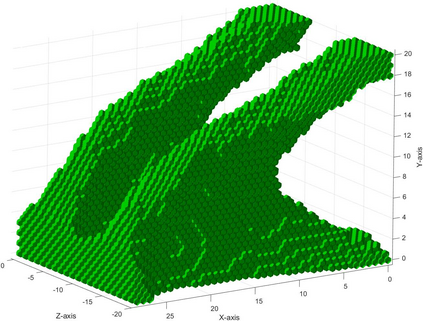
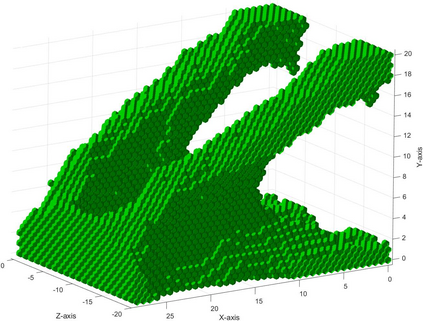
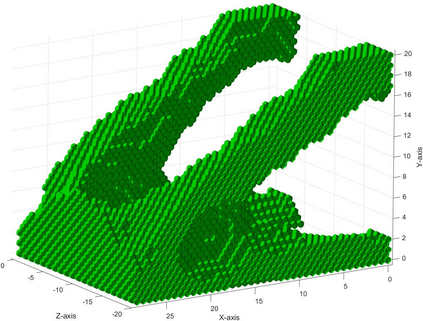
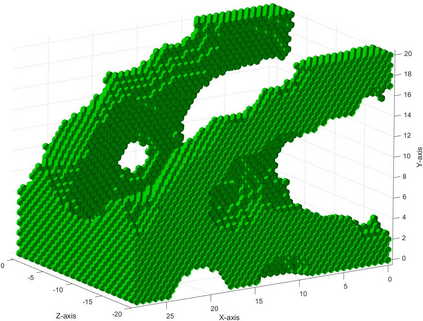
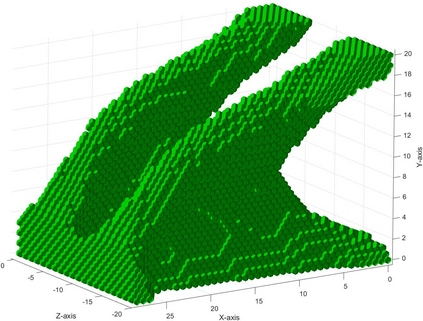
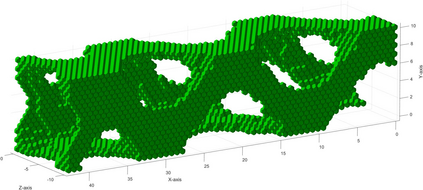
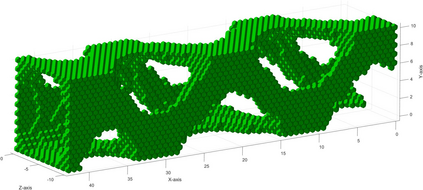
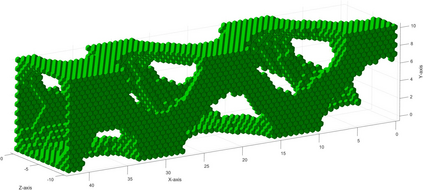
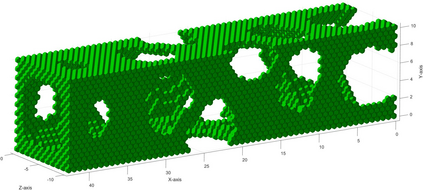
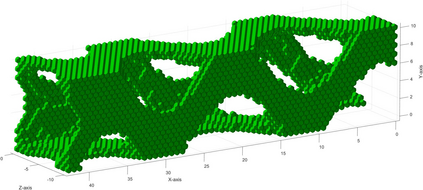
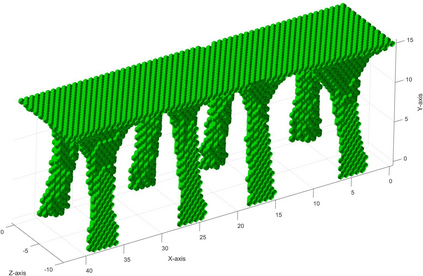
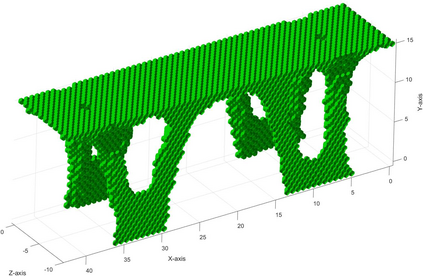
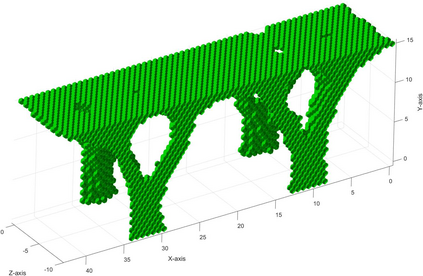
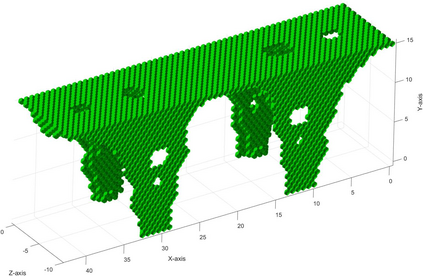
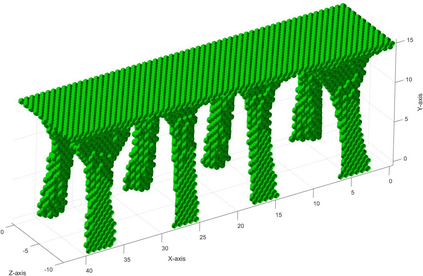
A novel meshing scheme, based on regular tetra-kai-decahedron, also referred to as truncated octahedron, cells is presented for use in spatial topology optimization. A tetra-kai-decahedron mesh ensures face connectivity between elements thereby eliminating singular solutions from the solution space. Various other benefits of implementing the said mesh are also highlighted, and the corresponding finite element is introduced. Material mask overlay strategy or MMOS, a feature based method for topology optimization is extended for use in 3-dimensions (MMOS-3D) via the aforementioned finite element and spheroidal negative masks. Formulation for density computation and sensitivity analysis for gradient based optimization is developed. Examples on traditional structural topology optimization problems are presented with detailed discussion on efficacy of the proposed approach.
翻译:以普通四面形-十二面形(也称为短短八面形)为基础的新式网格计划,被介绍用于空间地形优化;四面形-十二面形网格确保各元素之间的面对面连接,从而从解决方案空间中消除单一的解决方案;还强调了实施上述网格的其他各种好处,并引入了相应的有限要素;材料遮盖重叠战略或MMOS,即基于地表优化的基于地貌的方法(MMOS-3D)通过上述有限元素和甲状腺反面遮罩被扩展用于三面形(MMOS-3D)中;为基于优化的密度计算和敏感度分析制定了方案;介绍了传统结构表层优化问题的实例,并详细讨论了拟议方法的功效。