Regularized Rényi divergence minimization through Bregman proximal gradient algorithms

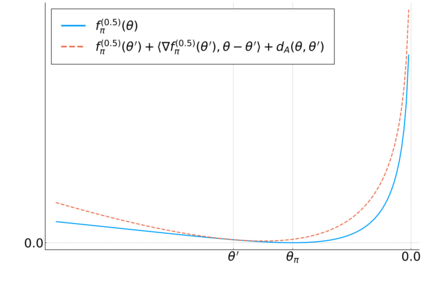
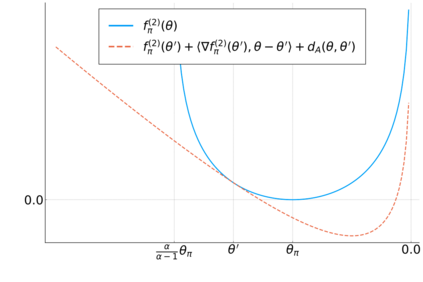
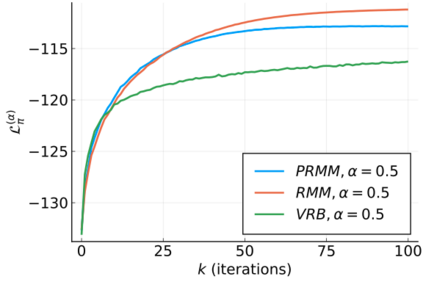
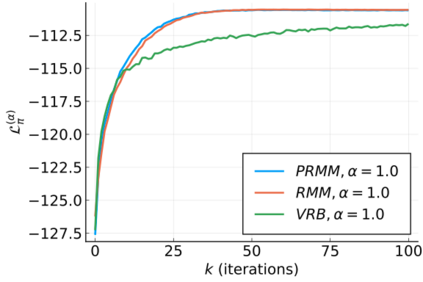
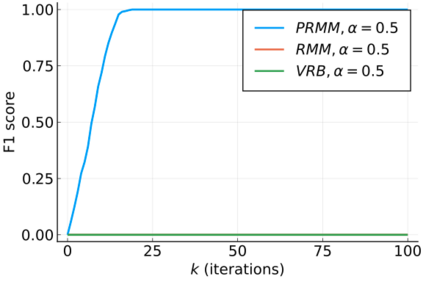
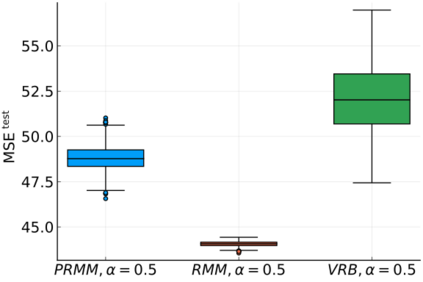
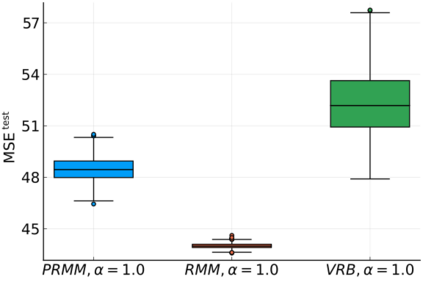
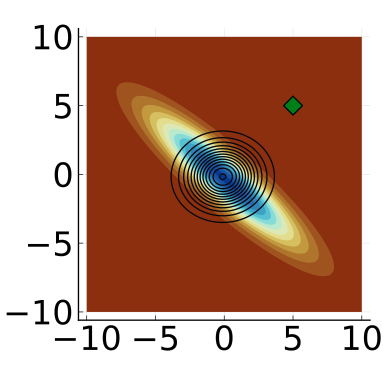
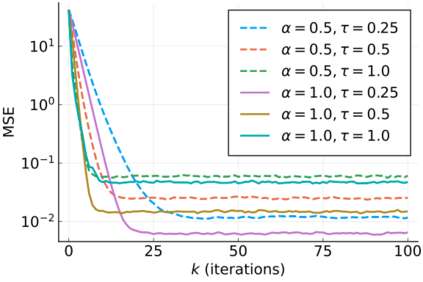
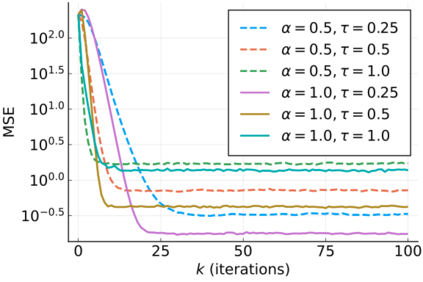
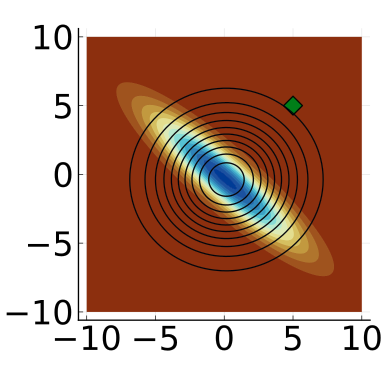
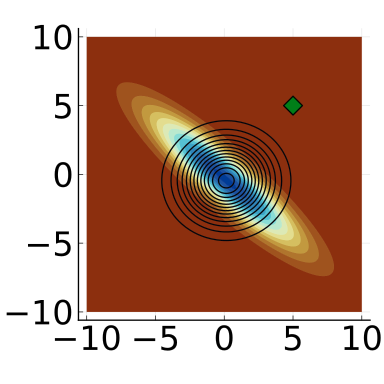
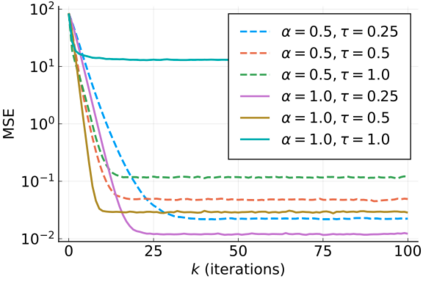
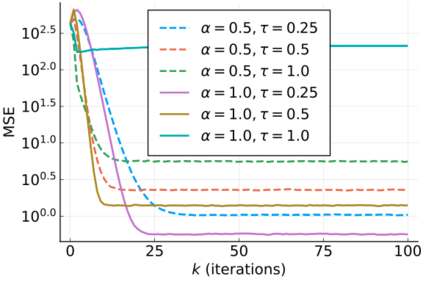
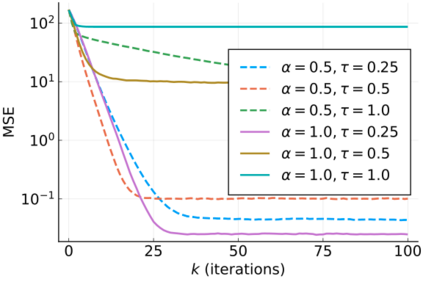
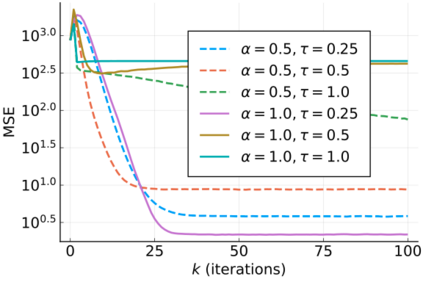
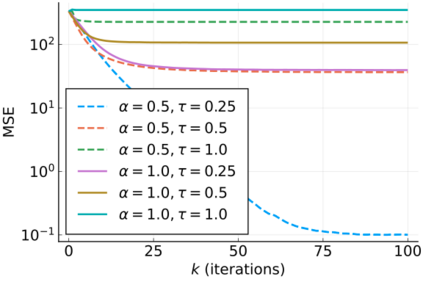
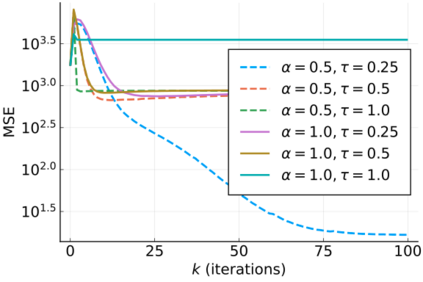
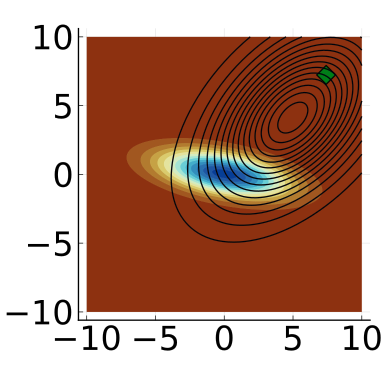
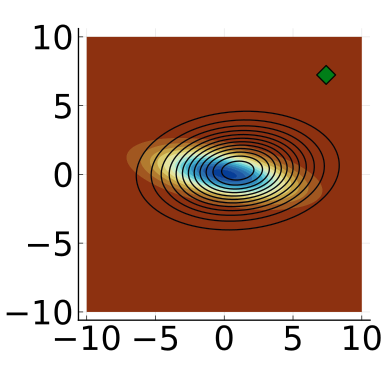
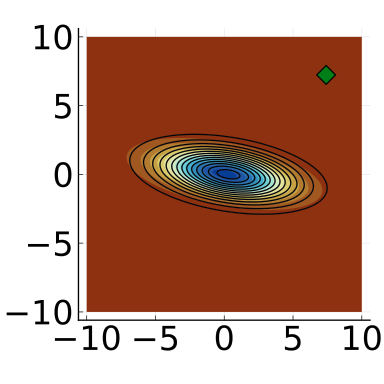
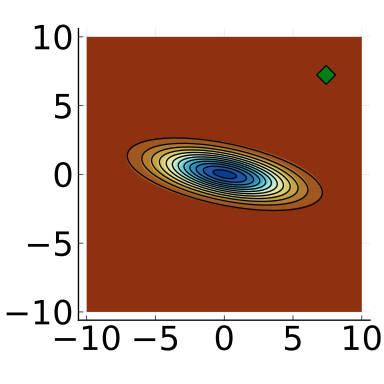
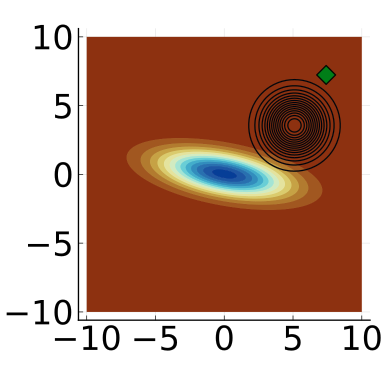
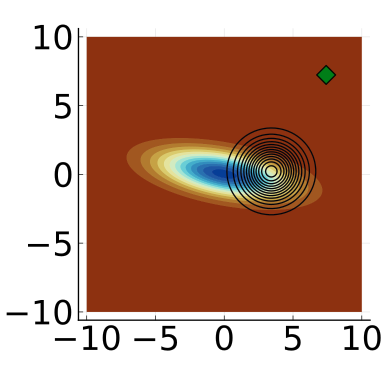
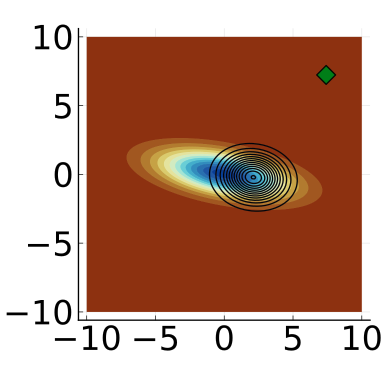
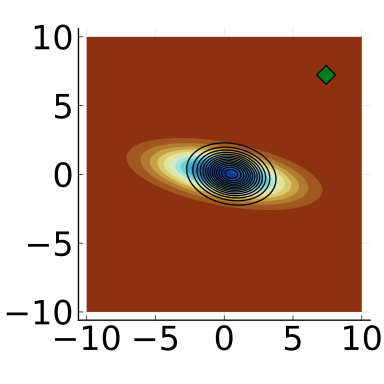
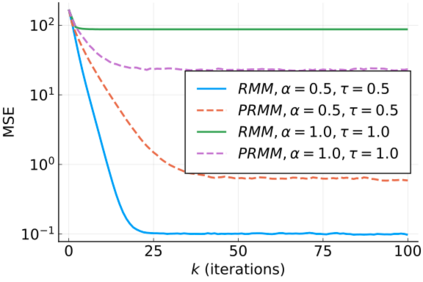
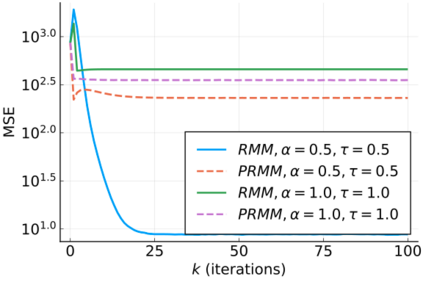
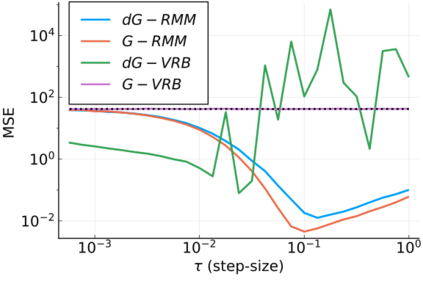
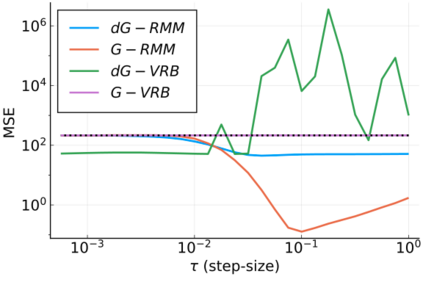
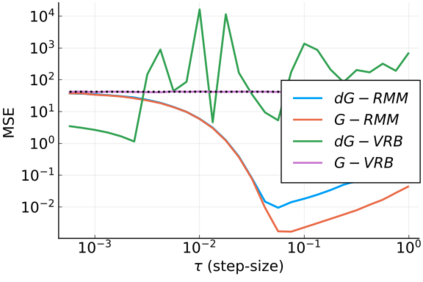
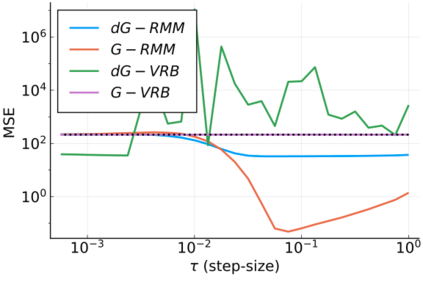
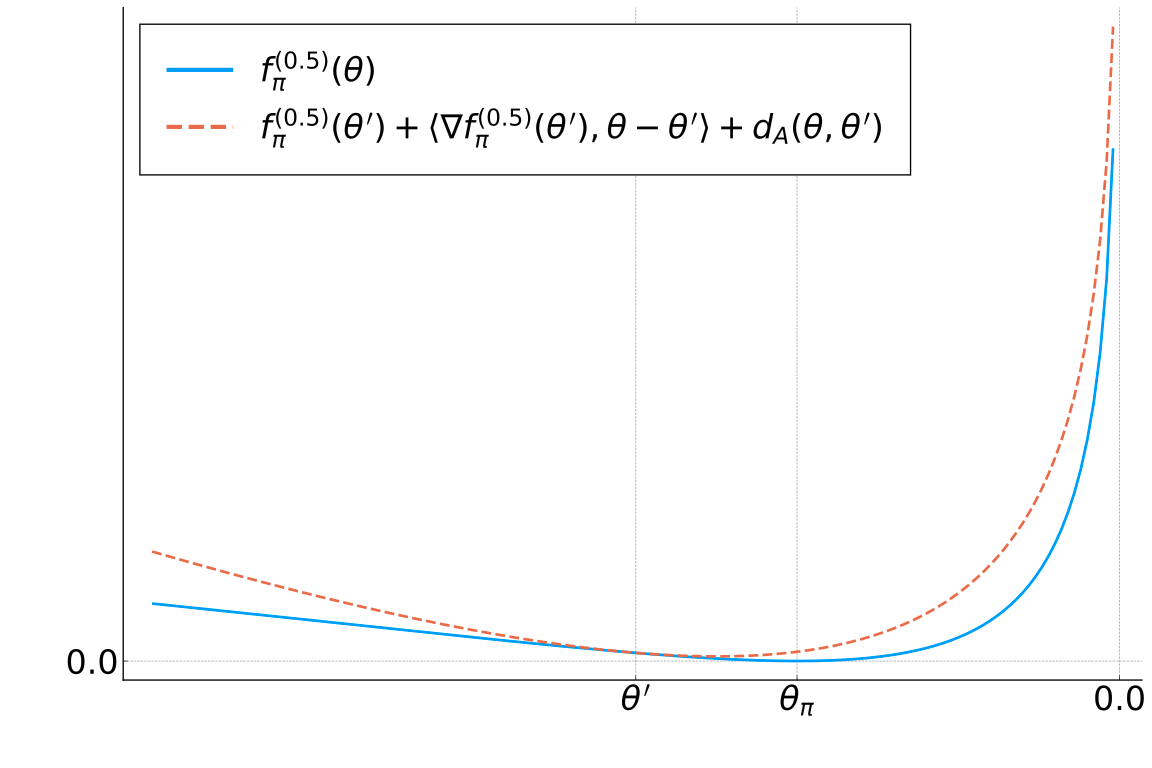
We study the variational inference problem of minimizing a regularized R\'enyi divergence over an exponential family, and propose a relaxed moment-matching algorithm, which includes a proximal-like step. Using the information-geometric link between Bregman divergences and the Kullback-Leibler divergence, this algorithm is shown to be equivalent to a Bregman proximal gradient algorithm. This novel perspective allows us to exploit the geometry of our approximate model while using stochastic black-box updates. We use this point of view to prove strong convergence guarantees including monotonic decrease of the objective, convergence to a stationary point or to the minimizer, and convergence rates. These new theoretical insights lead to a versatile, robust, and competitive method, as illustrated by numerical experiments.