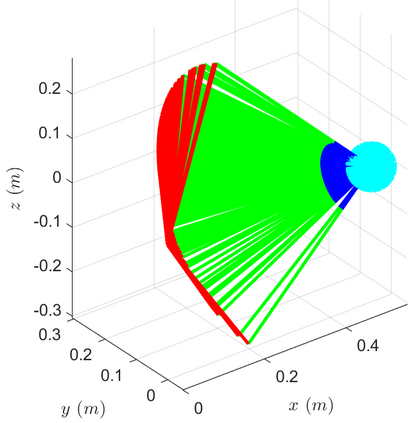
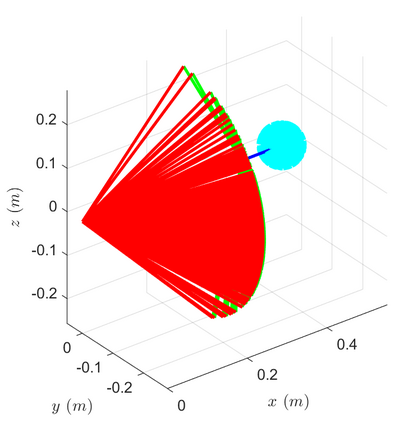
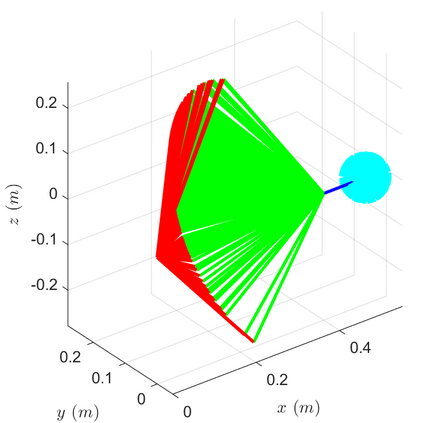
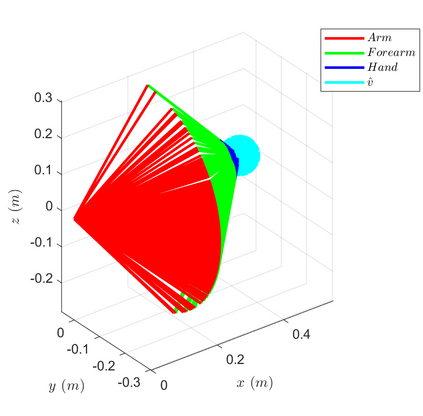
Robots are moving towards applications in less structured environments, but their model-based controllers are challenged by the tasks' complexity and intrinsic environmental unpredictability. Studying biological motor control can provide insights into overcoming these limitations due to the high dexterity and stability observable in humans and animals. This work presents a geometrical solution to the postural optimisation of 7-DoF limbs-like mechanisms, which are robust to singularities and computationally efficient. The theoretical formulation identified two separate decoupled optimisation strategies. The shoulder and elbow strategy align the plane of motion with the expected plane of motion and guarantee the reachability of the end-posture. The wrist strategy ensures the end-effector orientation, which is essential to retain manipulability when nearing a singular configuration. The numerical results confirmed the theoretical observations and allowed us to identify the effect of different grasp strategies on system manipulability. The geometrical method was numerically tested in thousands of configurations proving to be both robust and accurate. The tested scenarios include left and right arm postures, singular configurations, and walking scenarios. The proposed geometrical approach can find application in developing efficient and robust interaction controllers that could be applied in computational neuroscience and robotics.
翻译:机器人正在向结构不严谨的环境中应用,但模型控制器受到任务的复杂性和内在环境不可预测性的挑战。 研究生物运动控制可以提供洞察力,了解如何克服这些限制,因为人类和动物可以看到高度的伸缩性和稳定性。 这项工作为7-DoF四肢类机制的后院优化提供了几何解决方案,这些机制对奇点和计算效率都非常强大。 理论公式确定了两个分离的优化策略。 肩膀和肘部战略将运动的平面与预期的运动平面相匹配,并保证最终的姿势的可达性。 手腕战略确保最终效果方向,这对于在接近单一配置时保持人手性至关重要。 数字结果证实了理论观察,并使我们能够确定不同掌握策略对系统可操作性的影响。 几何方法在数千个配置中经过数字测试,证明既可靠又准确。 测试的情景包括左臂和右臂姿态、奇形配置和行走法情景。 拟议的几何测方法可以在开发高效和稳健的神经控制器中找到应用的机器人。