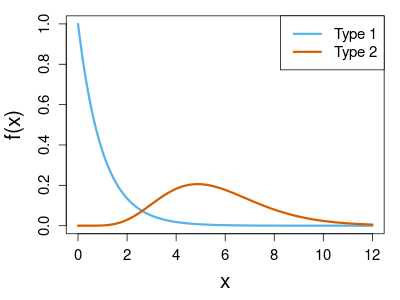
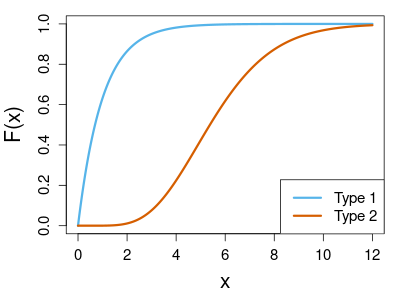
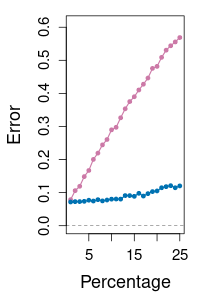
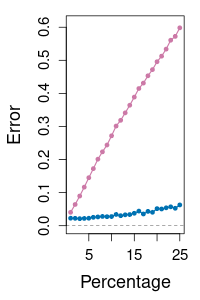
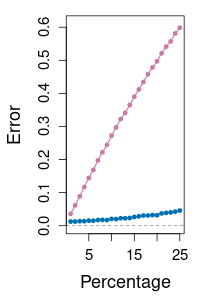
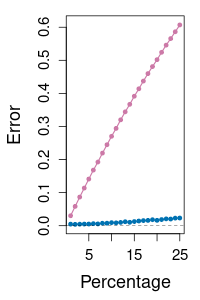
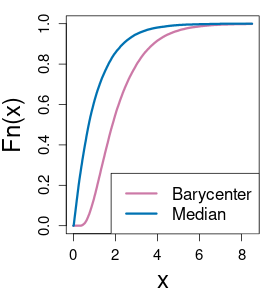
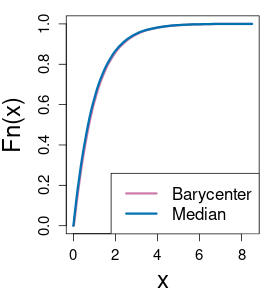
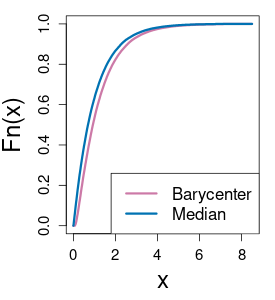
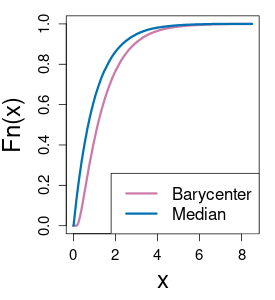
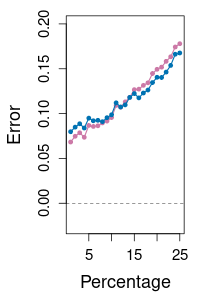
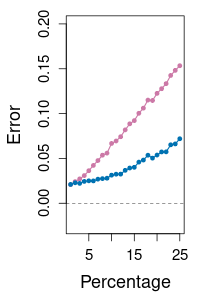
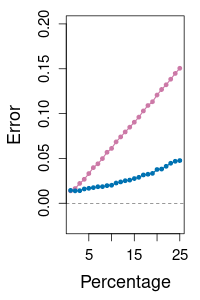
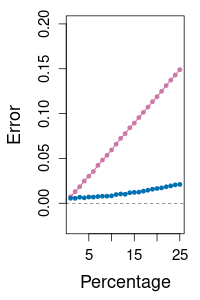
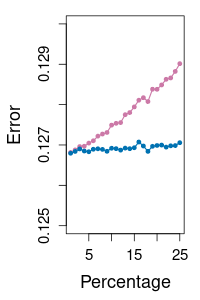
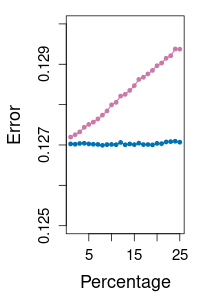
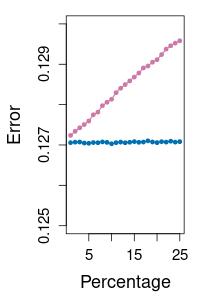
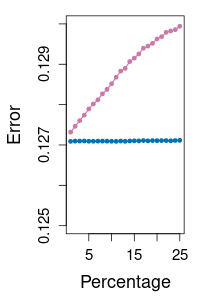
Measures of central tendency such as mean and median are a primary way to summarize a given collection of random objects. In the field of optimal transport, the Wasserstein barycenter corresponds to Fr\'{e}chet or geometric mean of a set of probability measures, which is defined as a minimizer of the sum of squared distances to each element in a given set when the order is 2. We present the Wasserstein median, an equivalent of Fr\'{e}chet median under the 2-Wasserstein metric, as a robust alternative to the Wasserstein barycenter. We first establish existence and consistency of the Wasserstein median. We also propose a generic algorithm that makes use of any established routine for the Wasserstein barycenter in an iterative manner and prove its convergence. Our proposal is validated with simulated and real data examples when the objects of interest are univariate distributions, centered Gaussian distributions, and discrete measures on regular grids.
翻译:在最佳运输领域,瓦森斯坦中枢与一套概率计量方法的Fr\'{e}chet或几何平均值相对应,被定义为在顺序为2时将特定组中每个元素的平方距离之和最小化。我们提出了瓦森斯坦中位数,相当于Wasserstein中位数的Fr\'{e}chet中位数,作为Wasserstein中位数的可靠替代物。我们首先确定了瓦瑟斯坦中位数的存在和一致性。我们还提议了一种通用算法,以迭接方式使用瓦瑟斯坦中枢的任何既定例行程序,并证明其趋同性。我们的提议得到模拟和真实数据实例的验证,当感兴趣的对象为单体分布、中心高斯的分布和常规网格上的离散措施时,我们的提议得到模拟和真实数据示例的验证。