Where to Drill Next? A Dual-Weighted Approach to Adaptive Optimal Design of Groundwater Surveys

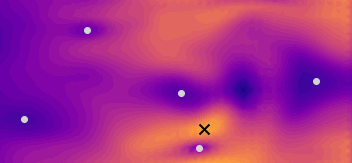
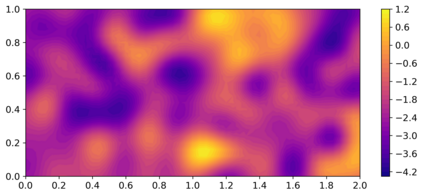
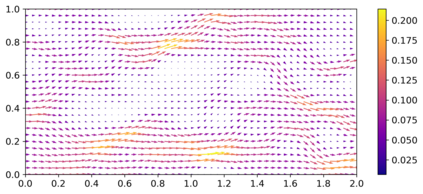
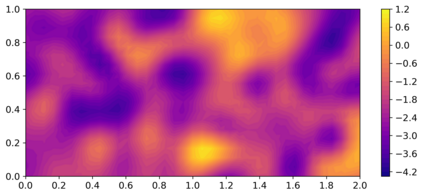
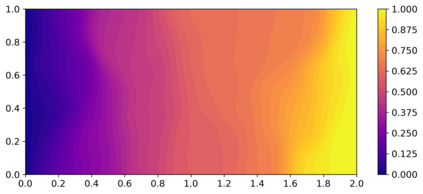
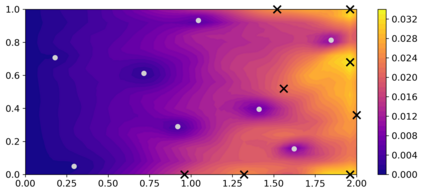
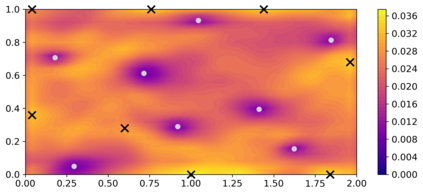
We present a novel approach to adaptive optimal design of groundwater surveys - a methodology for choosing the location of the next monitoring well. Our dual-weighted approach borrows ideas from Bayesian Optimisation and goal-oriented error estimation to propose the next monitoring well, given that some data is already available from existing wells. Our method is distinct from other optimal design strategies in that it does not rely on Fisher Information and it instead directly exploits the posterior uncertainty and the expected solution to a dual (or adjoint) problem to construct an acquisition function that optimally reduces the uncertainty in the model as a whole and some engineering quantity of interest in particular. We demonstrate our approach in the context of 2D groundwater flow example and show that the dual-weighted approach outperforms the baseline approach with respect to reducing the error in the posterior estimate of the quantity of interest.