On a linear Gromov-Wasserstein distance

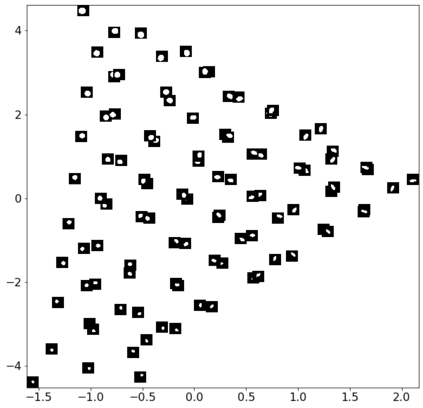
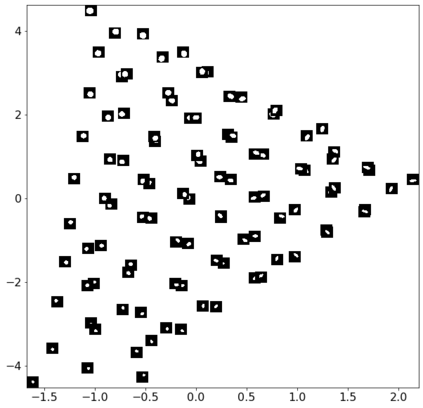
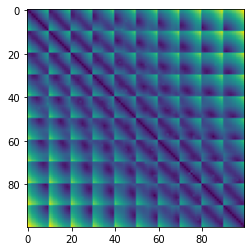
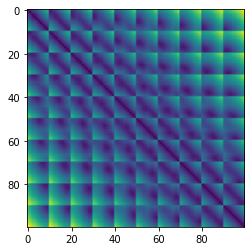
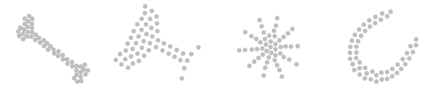
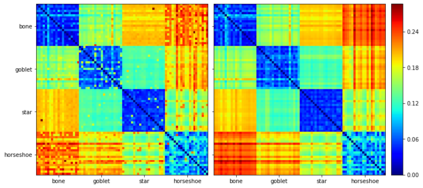
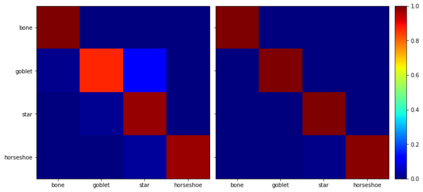
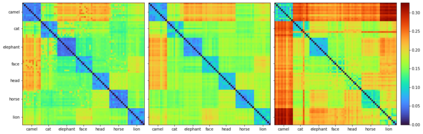
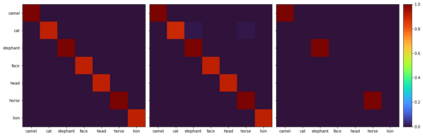
Gromov-Wasserstein distances are generalization of Wasserstein distances, which are invariant under certain distance preserving transformations. Although a simplified version of optimal transport in Wasserstein spaces, called linear optimal transport (LOT), was successfully used in certain applications, there does not exist a notation of linear Gromov-Wasserstein distances so far. In this paper, we propose a definition of linear Gromov-Wasserstein distances. We motivate our approach by a generalized LOT model, which is based on barycentric projections. Numerical examples illustrate that the linear Gromov-Wasserstein distances, similarly as LOT, can replace the expensive computation of pairwise Gromov-Wasserstein distances in certain applications.