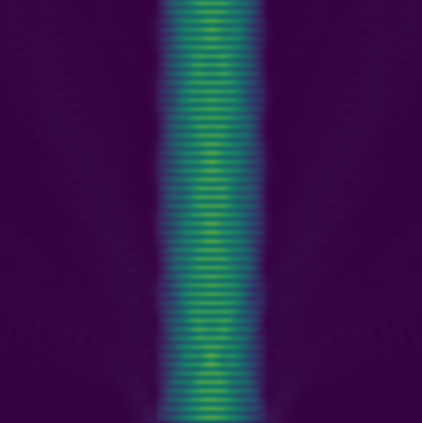
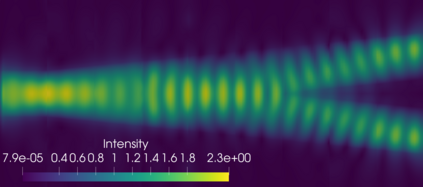
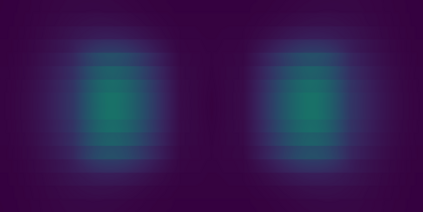
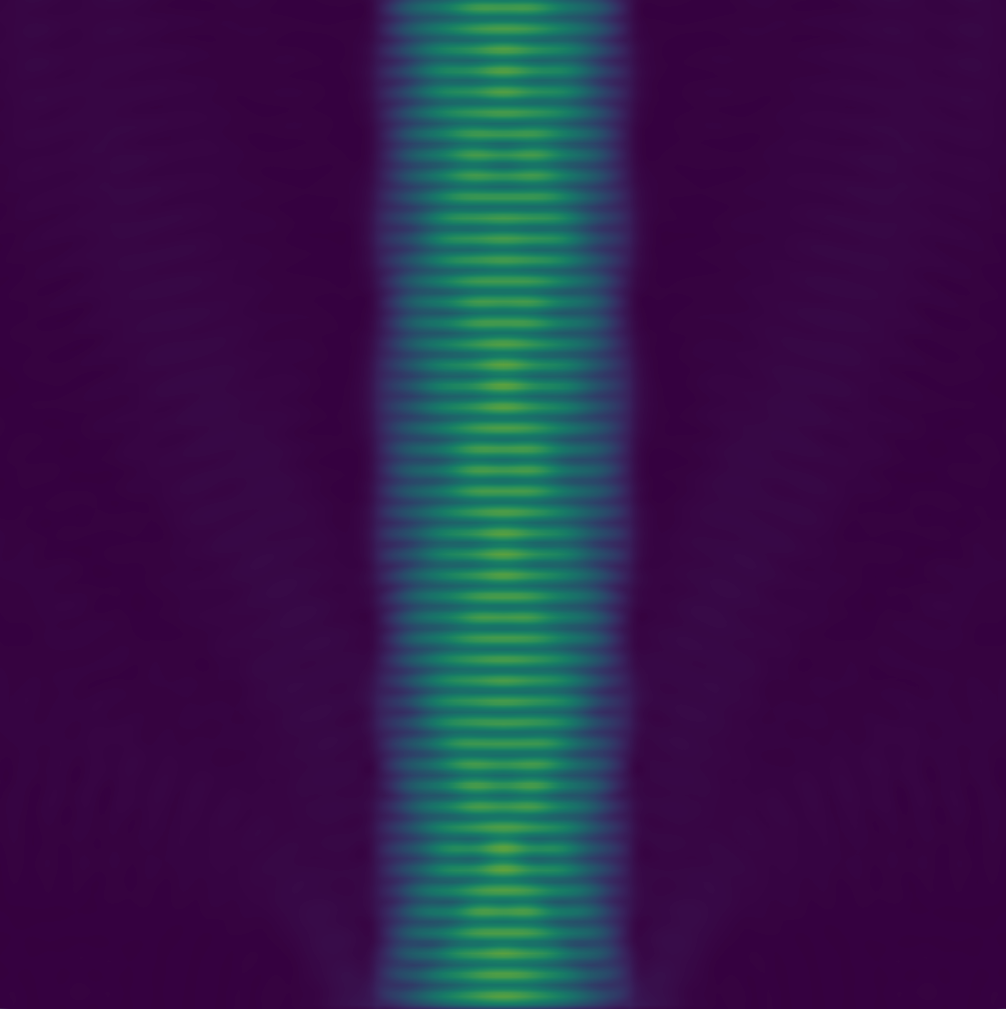
The time harmonic Maxwell equations are of current interest in computational science and applied mathematics with many applications in modern physics. In this work, we present parallel finite element solver for the time harmonic Maxwell equations and compare different preconditioners. We show numerically that standard preconditioners like incomplete LU and the additive Schwarz method lead to slow convergence for iterative solvers like generalized minimal residuals, especially for high wave numbers. Even more we show that also more specialized methods like the Schur complement method also yield slow convergence. As an example for a highly adapted solver for the time harmonic Maxwell equations we use a combination of a block preconditioner and a domain decomposition method (DDM), which also preforms well for high wave numbers. Additionally we discuss briefly further approaches to solve high frequency problems more efficiently. Our developments are done in the open-source finite element library deal.II.
翻译:时间调和 Maxwell 方程式目前对计算科学和应用数学感兴趣, 并具有现代物理学的许多应用。 在这项工作中, 我们为时间调和 Maxwell 方程式提供了平行的有限元素解析器, 并比较了不同的先决条件。 我们用数字显示, 标准前提( 如不完全的LU 和添加的 Schwarz 方法) 导致迭代解答器的缓慢趋同, 如通用最低残留物, 特别是高波数。 我们甚至更多显示, 更专门的方法( 如Schur 补充法) 也会产生缓慢的趋同。 作为时间调适的 Maxwell 方程式的高度调整解析器的一个例子, 我们使用块前置法和域分解法( DDM ) 的组合, 高波数也预示良好。 此外, 我们简要地讨论了更高效地解决高频率问题的进一步方法。 我们在开放源有限元素库交易中做了发展。 II。