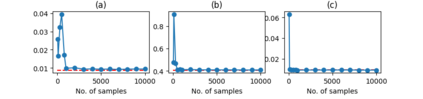
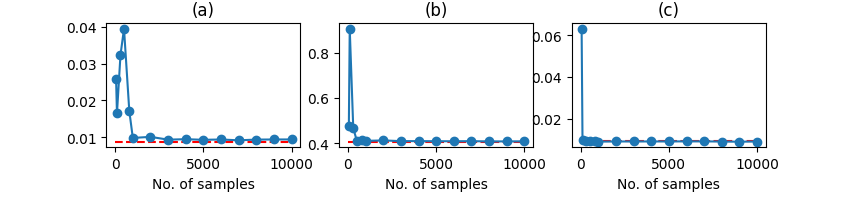
We present an operator-free, measure-theoretic approach to the conditional mean embedding (CME) as a random variable taking values in a reproducing kernel Hilbert space. While the kernel mean embedding of unconditional distributions has been defined rigorously, the existing operator-based approach of the conditional version depends on stringent assumptions that hinder its analysis. We overcome this limitation via a measure-theoretic treatment of CMEs. We derive a natural regression interpretation to obtain empirical estimates, and provide a thorough theoretical analysis thereof, including universal consistency. As natural by-products, we obtain the conditional analogues of the maximum mean discrepancy and Hilbert-Schmidt independence criterion, and demonstrate their behaviour via simulations.
翻译:我们对有条件中值嵌入(CME)提出了一种无操作者、无计量理论的方法,作为一种随机变量,作为复制内核Hilbert空间的随机变量。虽然内核意味着无条件分配的嵌入得到了严格的定义,但有条件版本的现有基于操作者的方法取决于阻碍其分析的严格假设。我们通过对CME的计量理论处理克服了这一限制。我们从自然回归中得出一种解释,以获得经验性估计,并对此进行彻底的理论分析,包括普遍一致性。作为自然副产品,我们获得了最大中值差异和Hilbert-Schmidt独立性标准的有条件类似,并通过模拟来展示其行为。