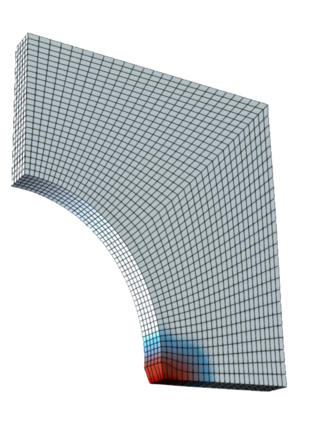
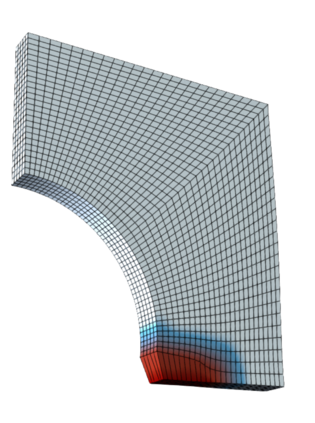
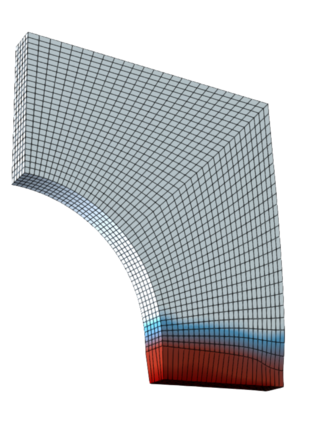
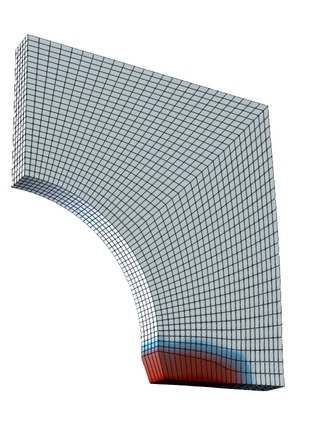
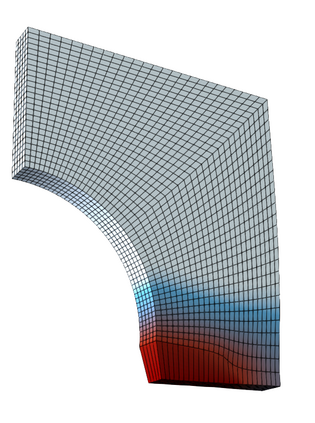
The modeling of damage processes in materials constitutes an ill-posed mathematical problem which manifests in mesh-dependent finite element results. The loss of ellipticity of the discrete system of equations is counteracted by regularization schemes of which the gradient enhancement of the strain energy density is often used. In this contribution, we present an extension of the efficient numerical treatment, which has been proposed in [1], to materials that are subjected to large deformations. Along with the model derivation, we present a technique for element erosion in the case of severely damaged materials. Efficiency and robustness of our approach is demonstrated by two numerical examples.
翻译:材料损害过程模型化是一个错误的数学问题,表现在依赖网状的有限元素结果中。离散方程式的省略性损失通过经常使用电源密度梯度梯度增强的正规化计划得到抵消。在这一贡献中,我们将[1]中提议的高效数字处理方法扩大到受大变形影响的材料。除了模型衍生外,我们还提出了在严重损坏材料的情况下进行元素侵蚀的技术。两个数字例子证明了我们方法的效率和稳健性。