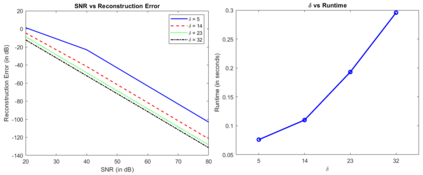
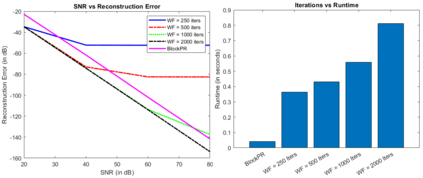
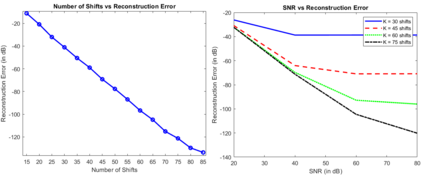
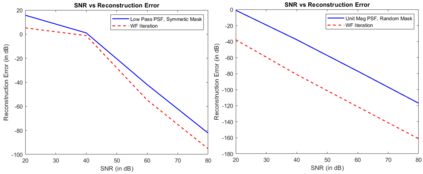
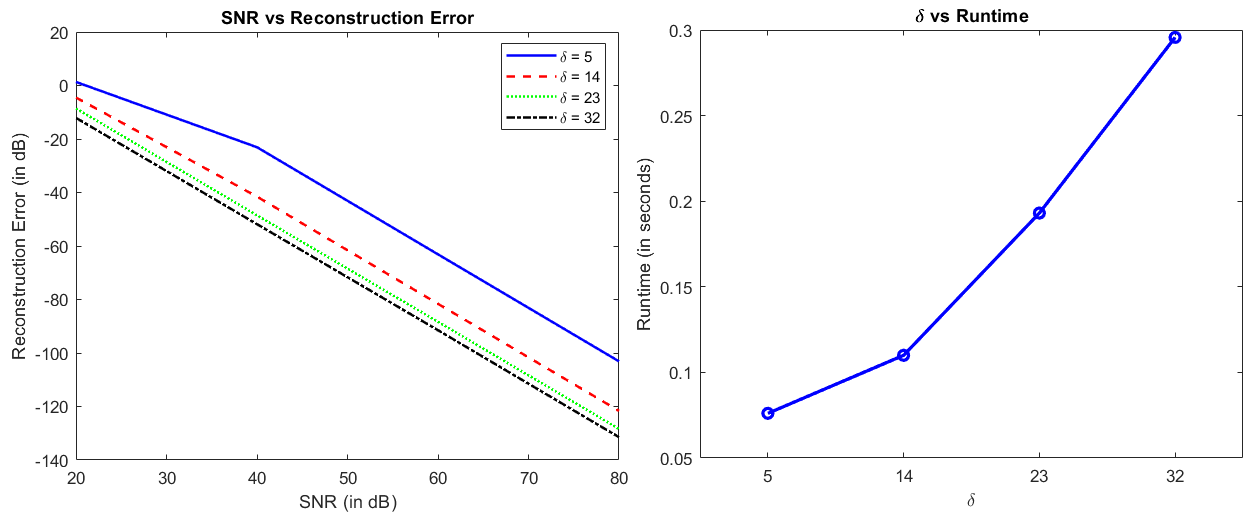
Ptychography is an imaging technique which involves a sample being illuminated by a coherent, localized probe of illumination. When the probe interacts with the sample, the light is diffracted and a diffraction pattern is detected. Then the sample (or probe) is shifted laterally in space to illuminate a new area of the sample whilst ensuring sufficient overlap. Near-field Ptychography (NFP) occurs when the sample is placed at a short defocus distance having a large Fresnel number. In this paper, we prove that certain NFP measurements are robustly invertible (up to an unavoidable global phase ambiguity) by constructing a point spread function and physical mask which leads to a well-conditioned lifted linear system. We then apply a block phase retrieval algorithm using weighted angular synchronization and prove that the proposed approach accurately recovers the measured sample. Finally, we also propose using a Wirtinger Flow for NFP problems and numerically evaluate that alternate approach both against our main proposed approach, as well as with NFP measurements for which our main approach does not apply.
翻译:光谱学是一种成像技术,它涉及通过一个连贯的局部照明探测器对样本进行光照。当探测器与样品发生相互作用时,光线是分解的,并检测出一种分解模式。然后,样品(或探测器)在空间中横向移动,以照亮样品的新区域,同时确保足够的重叠。近场的光谱学(NFP)发生于样品放在一个短距离的脱焦距离上,拥有一个大的Fresnel号。在本文中,我们证明某些NFP测量数据通过构建一个点分布函数和物理遮罩是完全不可忽略的(直至不可避免的全球阶段的模糊性),从而导致一个有良好条件的提升线性系统。我们随后采用一个区段检索算法,使用加权角同步法,证明拟议的方法准确地回收了测量的样品。最后,我们还提议对NFP问题使用Wirtinger流,并对替代方法进行数字评价,既要对照我们的主要方法,又要使用我们的主要方法不适用NFP的测量方法。