Error analysis of first time to a threshold value for partial differential equations

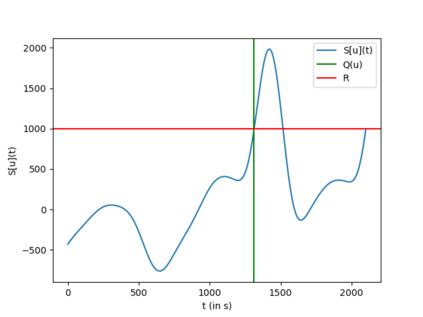
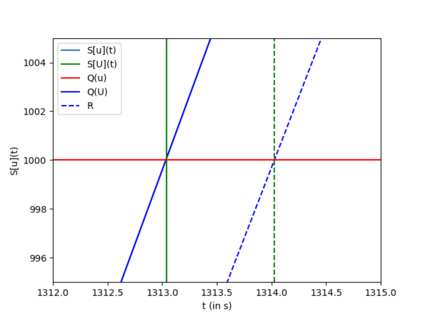
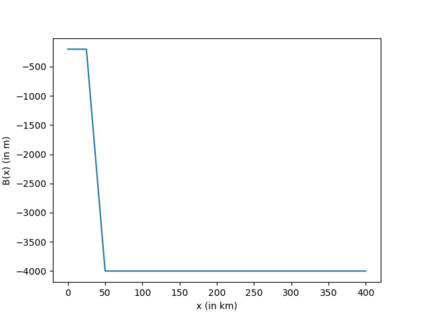
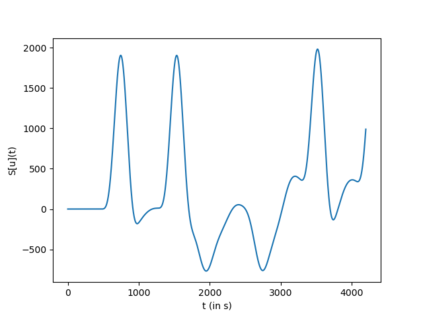
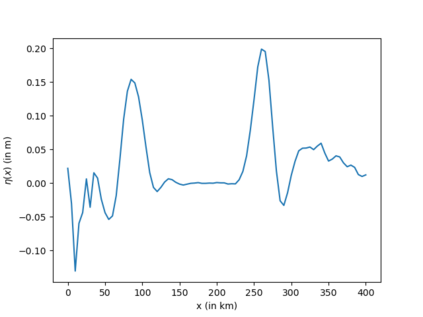
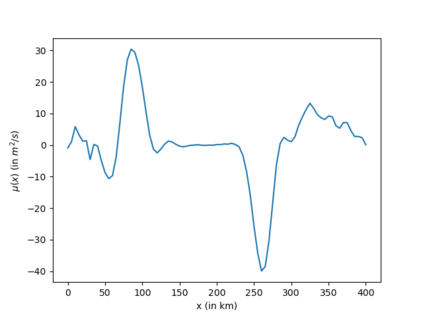
We develop an a posteriori error analysis for a novel quantity of interest (QoI) evolutionary partial differential equations (PDEs). Specifically, the QoI is the first time at which a functional of the solution to the PDE achieves a threshold value signifying a particular event, and differs from classical QoIs which are modeled as bounded linear functionals. We use Taylor's theorem and adjoint based analysis to derive computable and accurate error estimates for linear parabolic and hyperbolic PDEs. Specifically, the heat equation and linearized shallow water equations (SWE) are used for the parabolic and hyperbolic cases, respectively. Numerical examples illustrate the accuracy of the error estimates.