Nonparametric Estimation for SDE with Sparsely Sampled Paths: an FDA Perspective

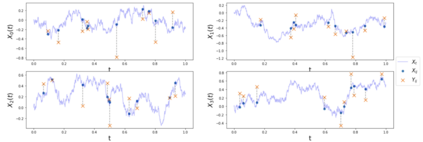
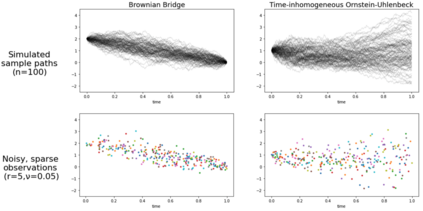
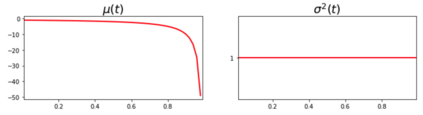
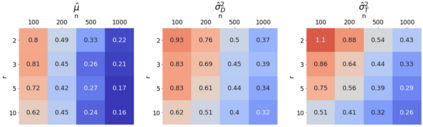
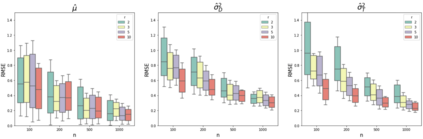
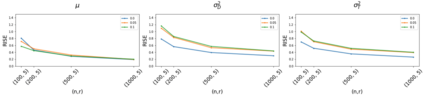
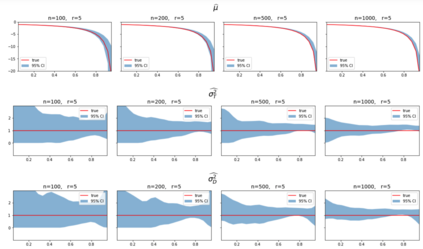
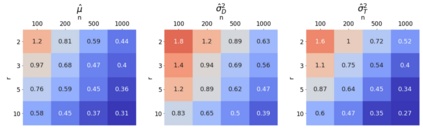
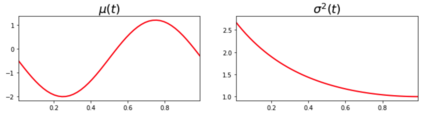
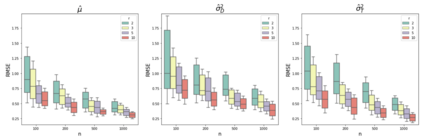
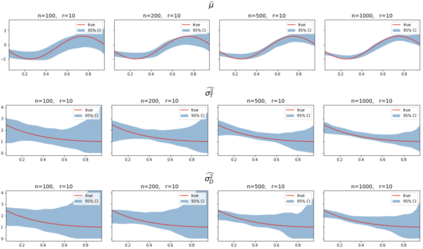
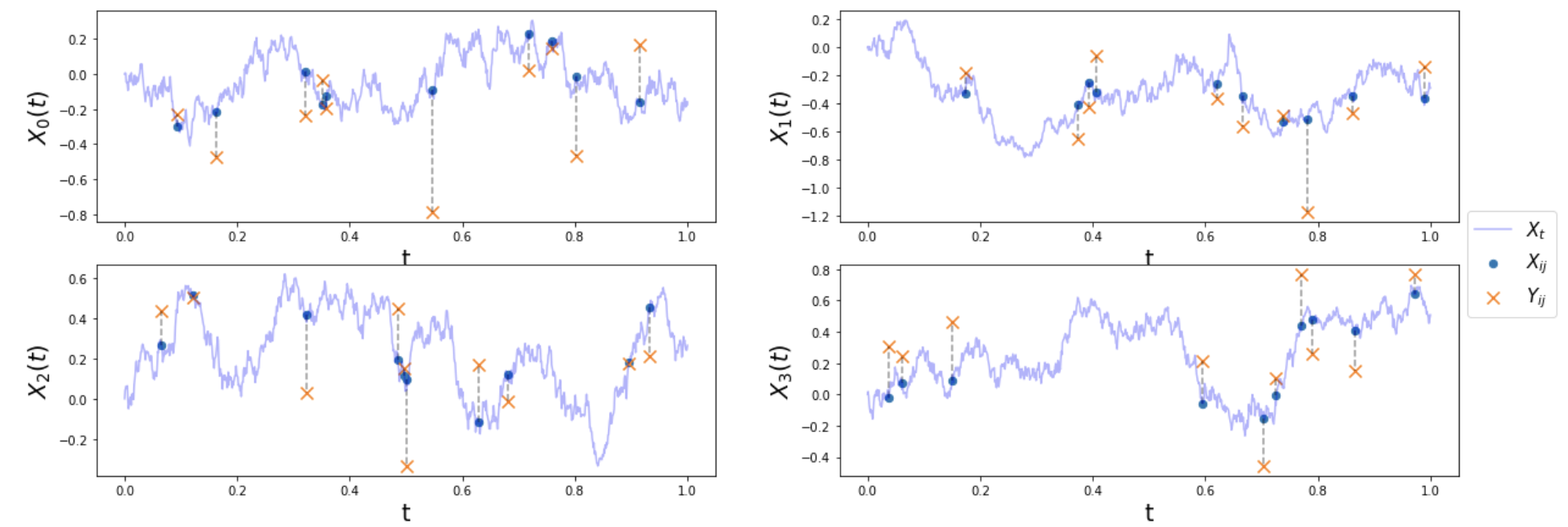
We consider the problem of nonparametric estimation of the drift and diffusion coefficients of a Stochastic Differential Equation (SDE), based on $n$ independent replicates $\left\{X_i(t)\::\: t\in [0,1]\right\}_{1 \leq i \leq n}$, observed sparsely and irregularly on the unit interval, and subject to additive noise corruption. By \textit{sparse} we intend to mean that the number of measurements per path can be arbitrary (as small as two), and remain constant with respect to $n$. We focus on time-inhomogeneous SDE of the form $dX_t = \mu(t)X_t^{\alpha}dt + \sigma(t)X_t^{\beta}dW_t$, where $\alpha \in \{0,1\}$ and $\beta \in \{0,1/2,1\}$, which includes prominent examples such as Brownian motion, Ornstein-Uhlenbeck process, geometric Brownian motion, and Brownian bridge. Our estimators are constructed by relating the local (drift/diffusion) parameters of the diffusion to their global parameters (mean/covariance, and their derivatives) by means of an apparently novel PDE. This allows us to use methods inspired by functional data analysis, and pool information across the sparsely measured paths. The methodology we develop is fully non-parametric and avoids any functional form specification on the time-dependency of either the drift function or the diffusion function. We establish almost sure uniform asymptotic convergence rates of the proposed estimators as the number of observed curves $n$ grows to infinity. Our rates are non-asymptotic in the number of measurements per path, explicitly reflecting how different sampling frequency might affect the speed of convergence. Our framework suggests possible further fruitful interactions between FDA and SDE methods in problems with replication.