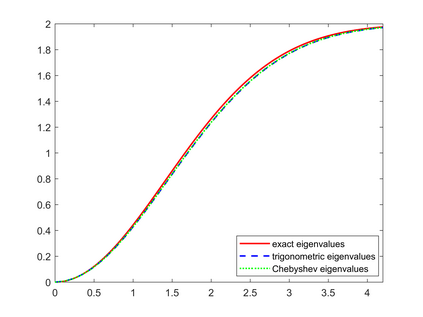
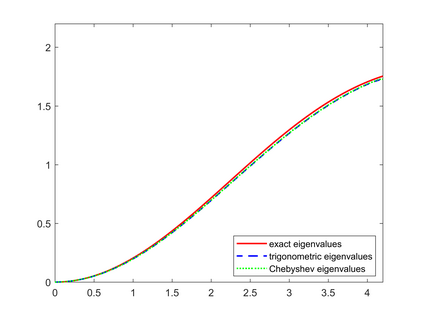
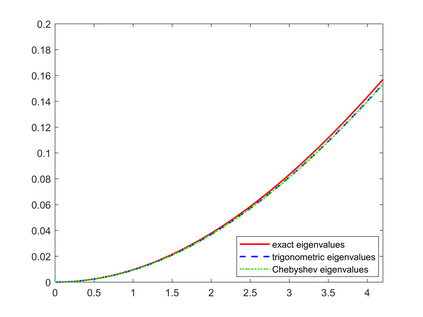
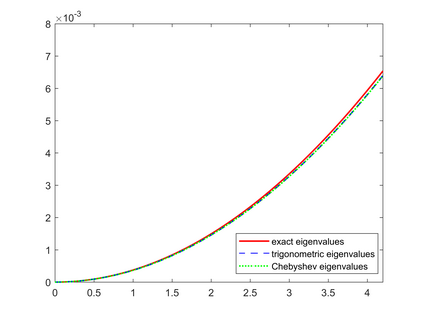
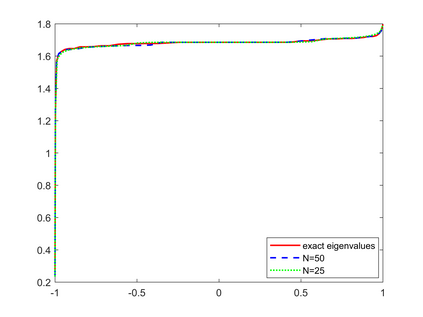
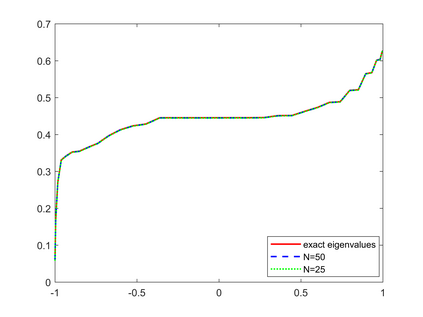
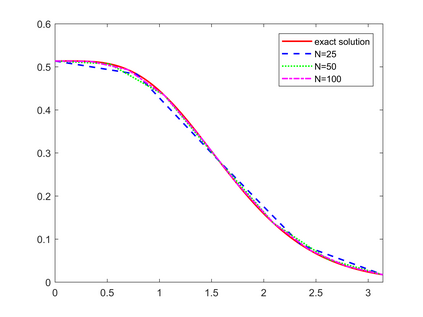
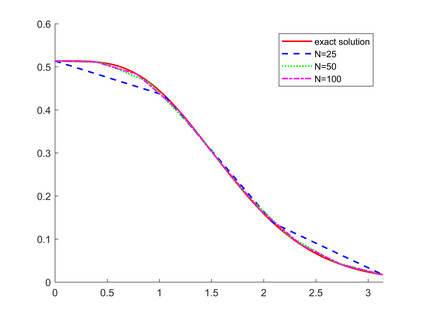
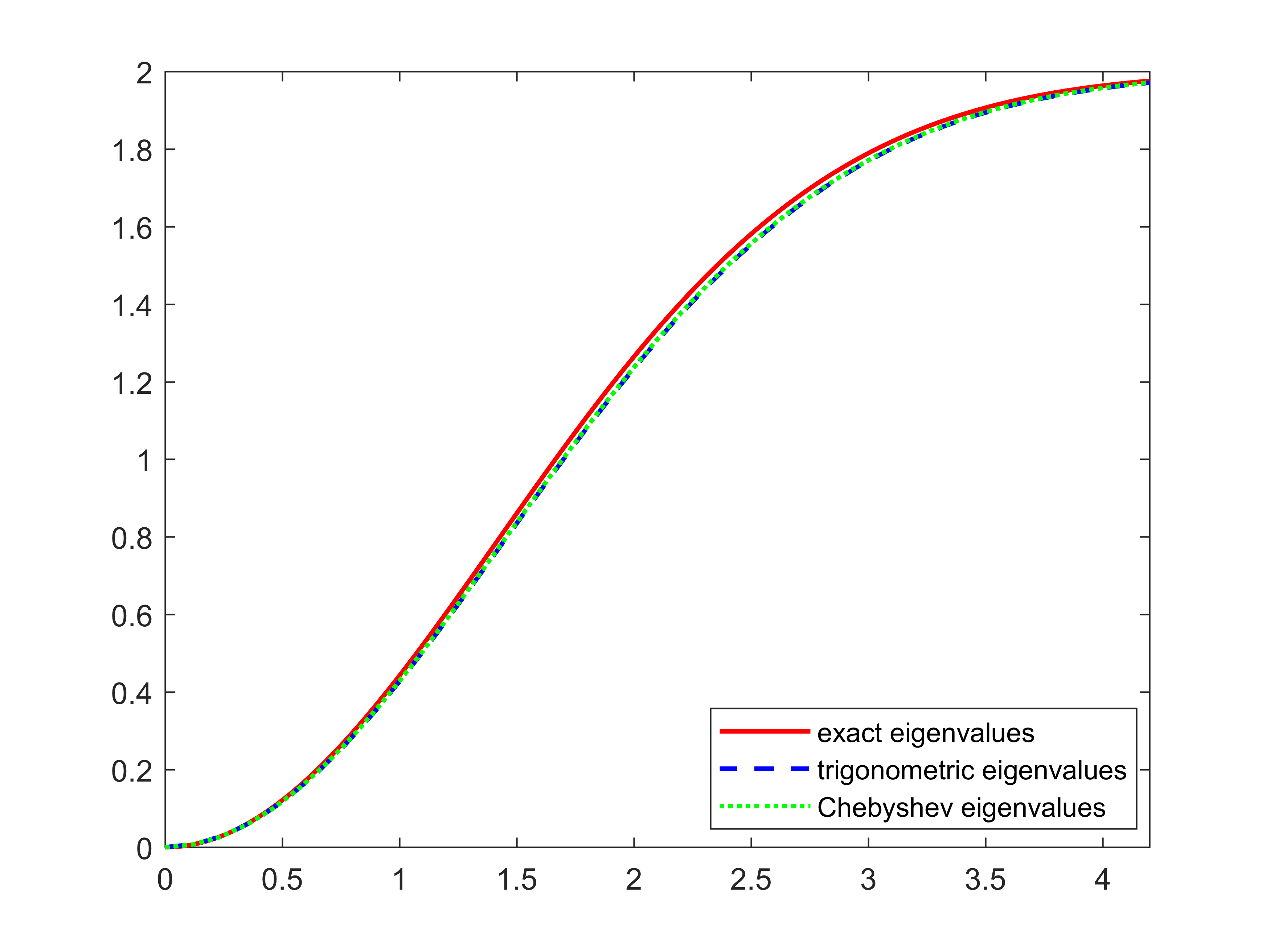
The purpose of this work is to study spectral methods to approximate the eigenvalues of nonlocal integral operators. Indeed, even if the spatial domain is an interval, it is very challenging to obtain closed analytical expressions for the eigenpairs of peridynamic operators. Our approach is based on the weak formulation of eigenvalue problem and we consider as orthogonal basis to compute the eigenvalues a set of Fourier trigonometric or Chebyshev polynomials. We show the order of convergence for eigenvalues and eigenfunctions in $L^2$-norm, and finally, we perform some numerical simulations to compare the two proposed methods.
翻译:这项工作的目的是研究光谱方法,以近似非本地整体操作员的精华值。 事实上,即使空间域是一个间隔,为远地动力操作员的精华元获得封闭的分析表达式也是非常困难的。 我们的方法是基于微弱的精华问题配制,我们认为作为正方位基础来计算精华值的一组Fourier三角测算法或Chebyshev多功能。 我们用$L2-norm来显示精华值和精巧功能的趋同顺序,最后,我们进行了一些数字模拟,以比较两种拟议方法。