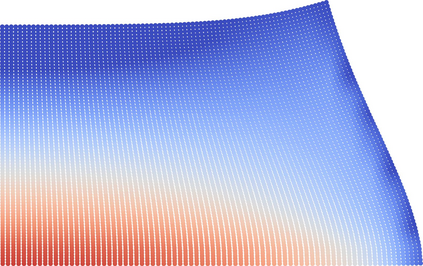
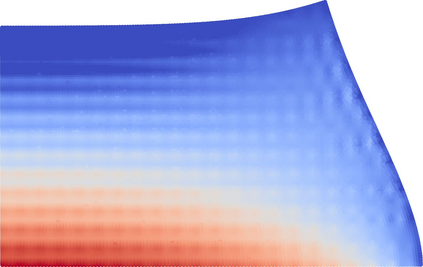
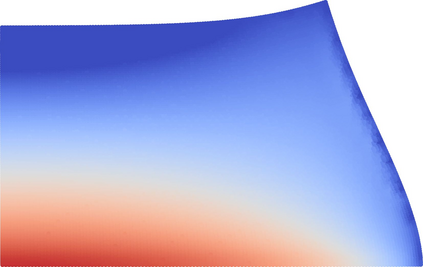
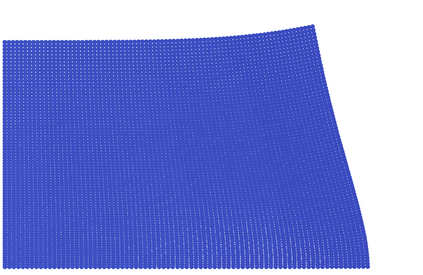
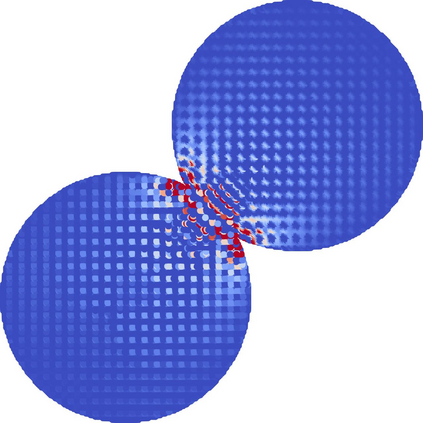
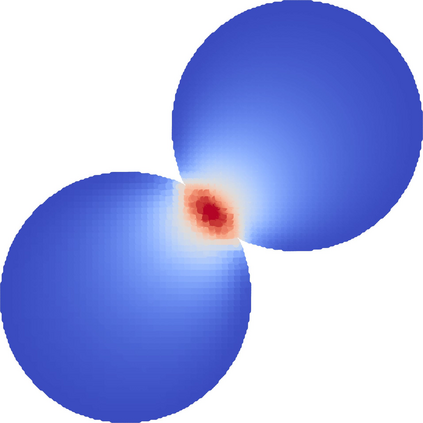
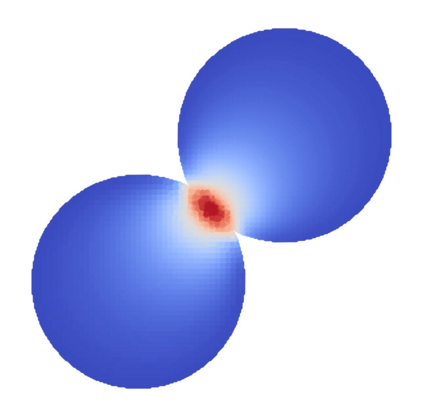
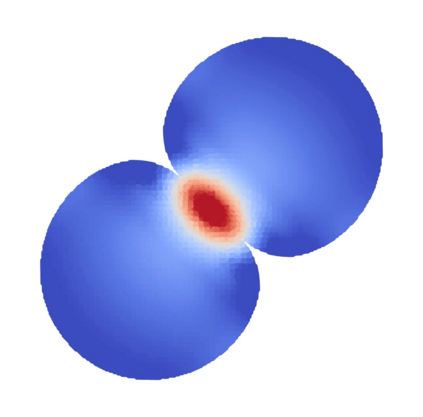
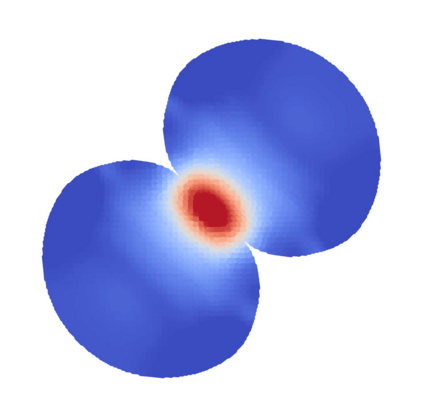
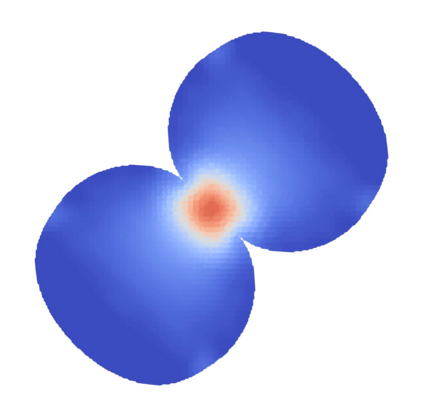
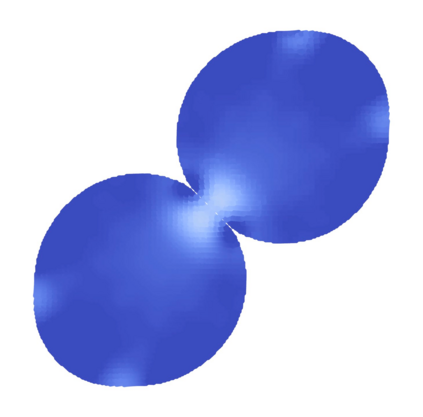
The material point method (MPM) has been increasingly used for the simulation of large deformation processes in fluid-infiltrated porous materials. For undrained poromechanical problems, however, standard MPMs are numerically unstable because they use low-order interpolation functions that violate the inf-sup stability condition. In this work, we develop stabilized MPM formulations for dynamic and quasi-static poromechanics that permit the use of standard low-order interpolation functions notwithstanding the drainage condition. For the stabilization of both dynamic and quasi-static formulations, we utilize the polynomial pressure projection method whereby a stabilization term is augmented to the balance of mass. The stabilization term can be implemented with both the original and generalized interpolation material point (GIMP) methods, and it is compatible with existing time-integration methods. Here we use fully-implicit methods for both dynamic and quasi-static poromechanical problems, aided by a block-preconditioned Newton-Krylov solver. The stabilized MPMs are verified and investigated through several numerical examples under dynamic and quasi-static conditions. Results show that the proposed MPM formulations allow standard low-order interpolation functions to be used for both the solid displacement and pore pressure fields of poromechanical formulations, from undrained to drained conditions, and from dynamic to quasi-static conditions.
翻译:材料点法(MPM)已越来越多地用于模拟流体过滤多孔材料中大型变形过程的模拟。但是,对于未排水的温室机械问题,标准的MPM在数字上不稳定,因为它们使用违反内分稳定条件的低级内插功能。在这项工作中,我们为动态和准静态的温室机械学开发稳定的MMM配方,允许在排水条件下使用标准的低级内插功能。为了稳定动态和准静态配方,我们使用多式压力预测方法,使稳定化术语扩大至质量平衡。稳定化术语可以用原始和通用的内插材料点(GIMP)方法来实施,并与现有的时间融合方法相容。我们在这里,我们使用完全隐蔽的方法处理动态和半静态的内插器问题,同时借助一个固定式的牛顿-克里洛夫溶剂溶剂。稳定的MPMMMM在动态和准静态间流成型中通过几个非数字例子加以核查和调查,从动态和准静态的内插式的内置状态下进行,允许从动态和静态的平流、低压场的研拟的磁场进行标准的状态设计。