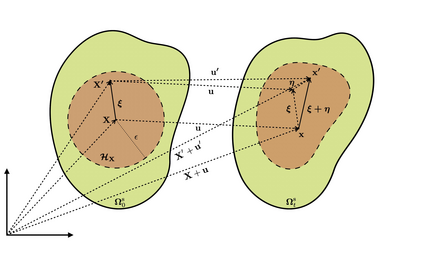
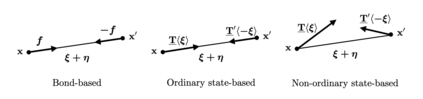
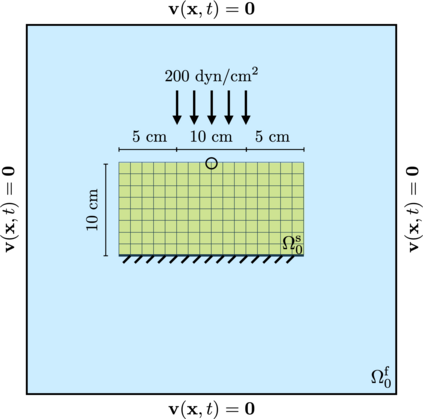
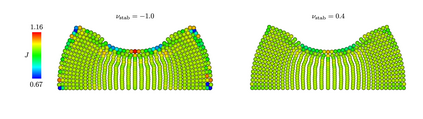
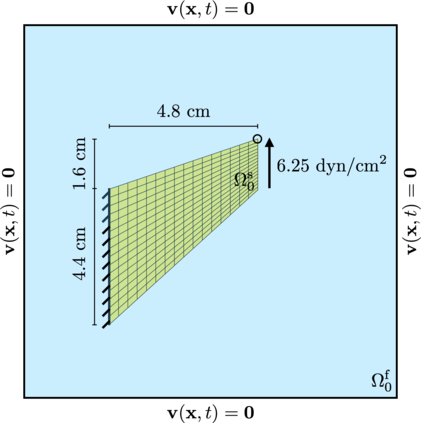
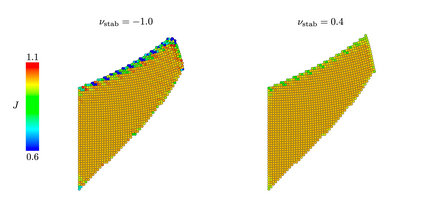
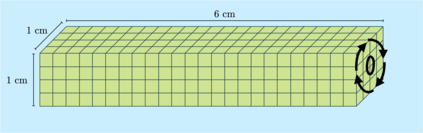
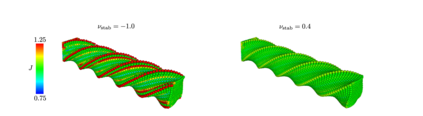
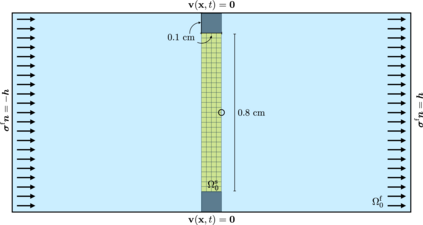
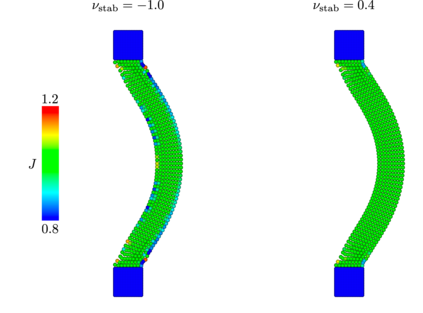
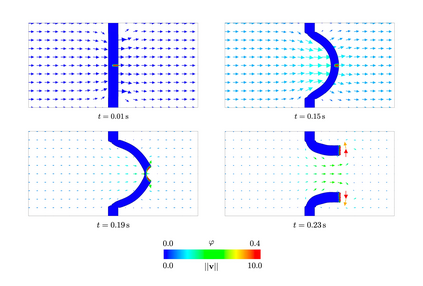
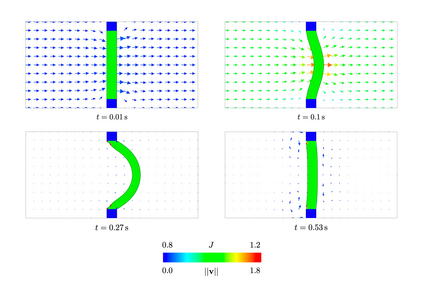
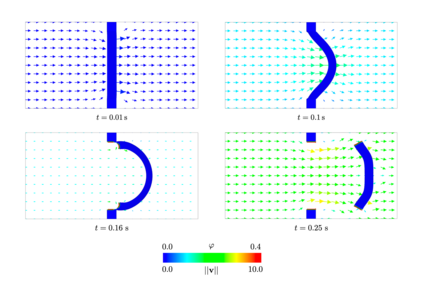
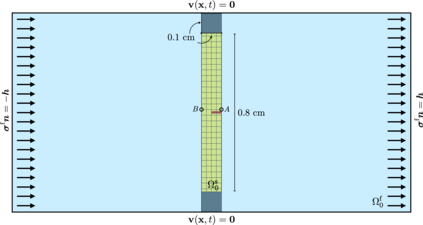
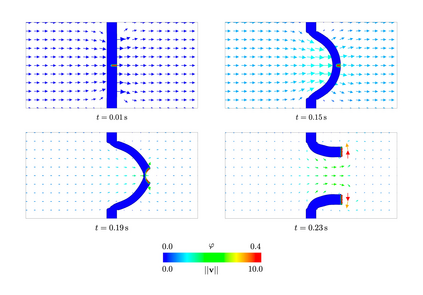
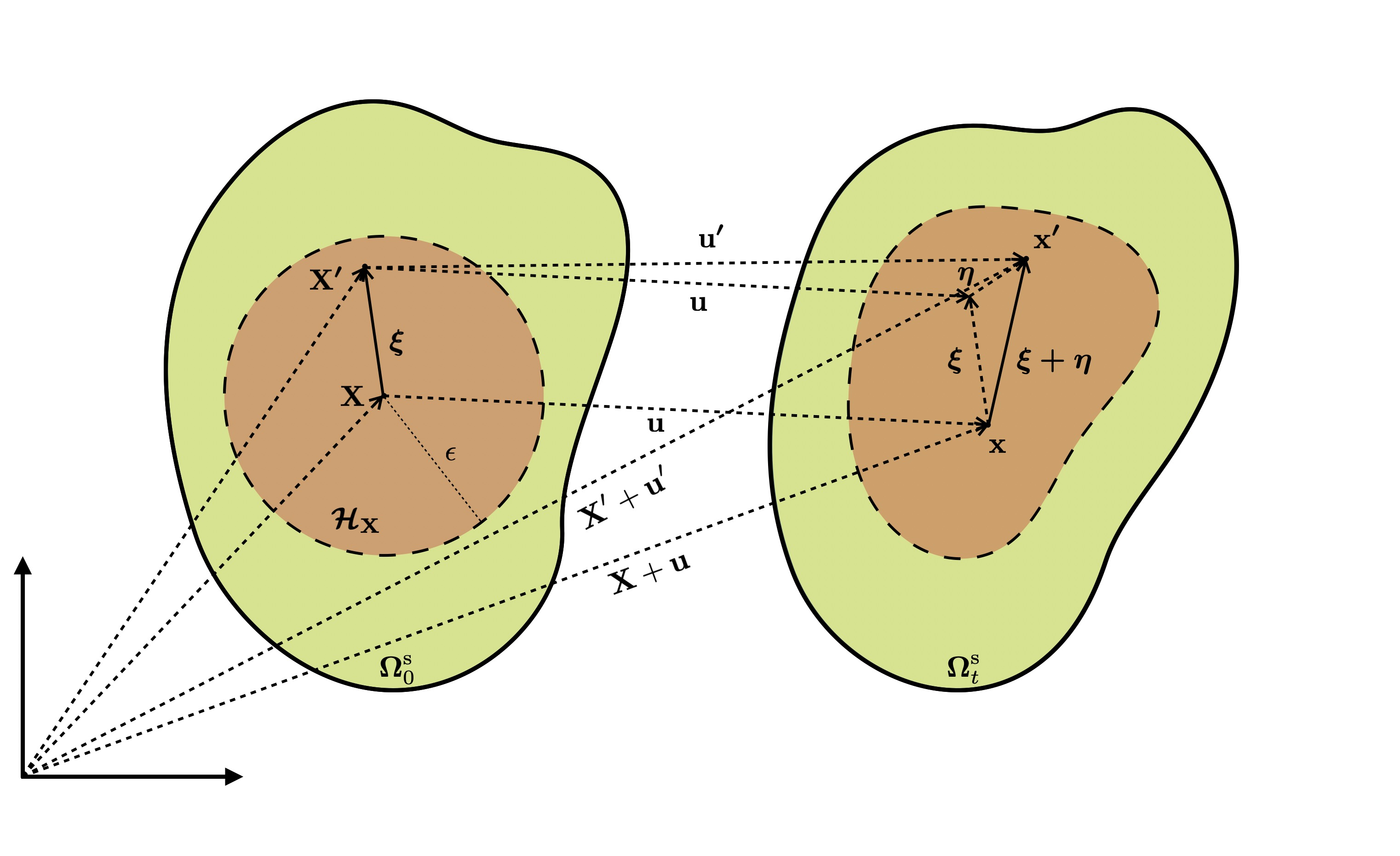
This paper develops and benchmarks an immersed peridynamics method to simulate the deformation, damage, and failure of hyperelastic materials within a fluid-structure interaction framework. The immersed peridynamics method describes an incompressible structure immersed in a viscous incompressible fluid. It expresses the momentum equation and incompressibility constraint in Eulerian form, and it describes the structural motion and resultant forces in Lagrangian form. Coupling between Eulerian and Lagrangian variables is achieved by integral transforms with Dirac delta function kernels, as in standard immersed boundary (IB) methods. The major difference between our approach and conventional IB methods is that we use peridynamics, instead of classical continuum mechanics, to determine the structural forces. We focus on non-ordinary state-based peridynamic material descriptions that allow us to use a constitutive correspondence framework that can leverage well characterized nonlinear constitutive models of soft materials. The convergence and accuracy of our approach are compared to both conventional and immersed finite element methods using widely used benchmark problems of nonlinear incompressible elasticity. We demonstrate that the immersed peridynamics method yields comparable accuracy with similar numbers of structural degrees of freedom for several choices of the size of the peridynamic horizon. We also demonstrate that the method can generate grid-converged simulations of fluid-driven material damage growth, crack formation and propagation, and rupture under large deformations.
翻译:本文开发和基准一种沉浸的远洋动力学方法,以模拟流体结构互动框架内超弹性材料的变形、损坏和故障。 沉浸的远洋动力学方法描述了一种浸泡在粘结性不可压缩液体中的不可压缩结构。 它展示了Eularian形态中的势头方程式和抑制力,并描述了以Lagrangian形态出现的结构运动和结果力。 Eulerian和Lagrangian变异变量之间的结合是通过在标准沉浸性边界(IB)方法中以Dirac delta函数内核整体变形、损坏和超弹性内核变形实现的。 我们的方法与常规和沉淀性变异性内核(IB)方法的主要区别是,我们的方法和常规的IB方法之间的主要区别是,我们使用不压缩性结构动力学,而不是传统的连续体力力力力力力力力力,我们用这个方法的深度变压性变压性精度的精度,我们用一个结构性变精度的精确度方法, 来展示各种不易变压性变压的精确度的精确度的精确度,我们用一系列的精确度法的精确度方法, 。