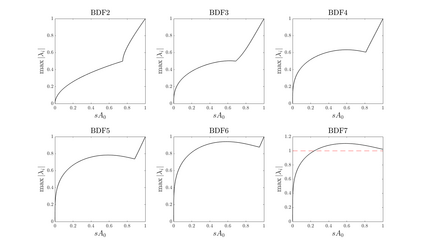
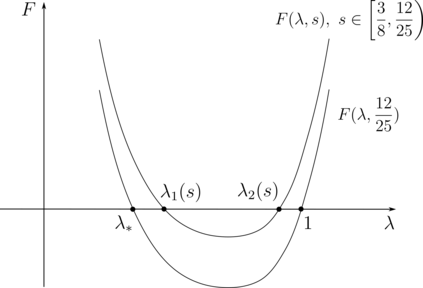
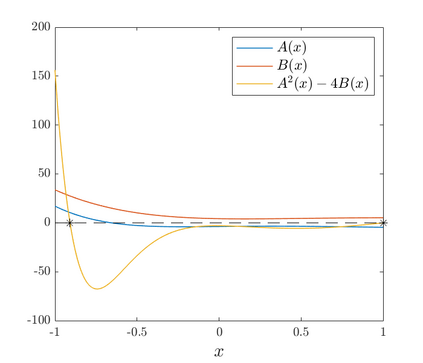
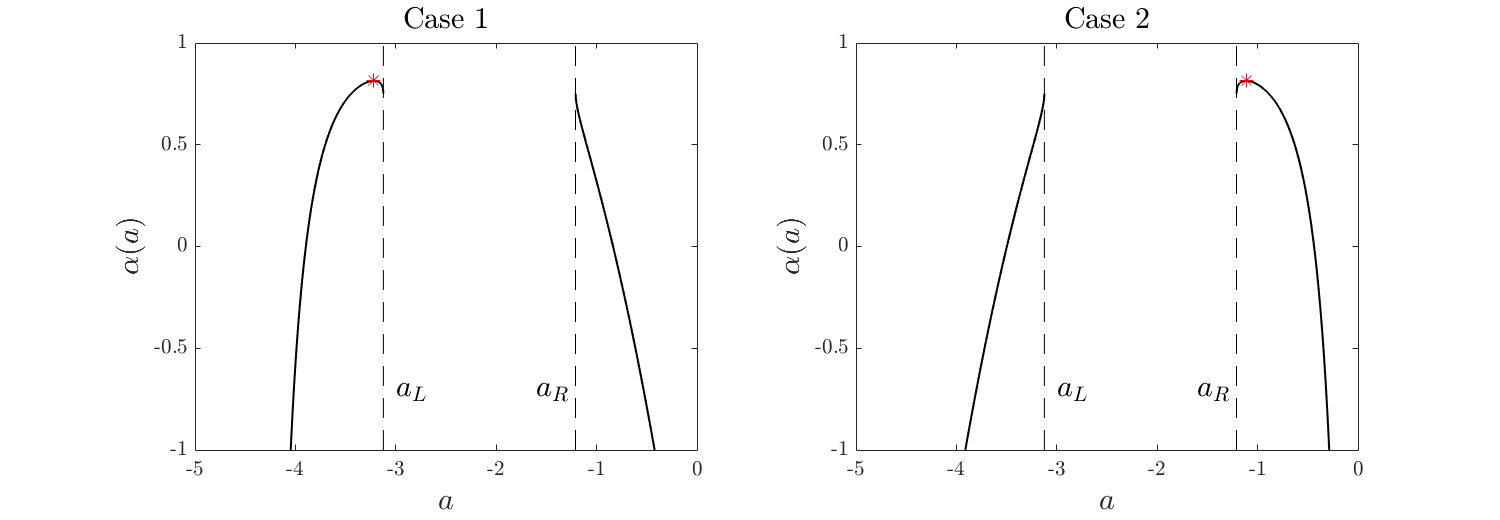
We introduce a new $\mathbf F$-modulated energy stability framework for general linear multistep methods. We showcase the theory for the two dimensional molecular beam epitaxy model with no slope selection which is a prototypical gradient flow with Lipschitz-bounded nonlinearity. We employ a class of representative BDF$k$, $2\le k \le 5$ discretization schemes with explicit $k^{\mathrm{th}}$-order extrapolation of the nonlinear term. We prove the uniform-in-time boundedness of high Sobolev norms of the numerical solution. The upper bound is unconditional, i.e. regardless of the size of the time step. We develop a new algebraic theory and calibrate nearly optimal and \emph{explicit} maximal time step constraints which guarantee monotonic $\mathbf F$-modulated energy dissipation.
翻译:我们为普通线性多步法引入一个新的 $mathbf F$ 调制的能源稳定性框架。 我们展示了两个维分子束相上层税模型的理论, 没有斜度选择, 这是一种原始的梯度流, 由利普西茨限制的非直线性。 我们使用一组具有代表性的 BDF$k$, 2\le k k\le 5$ 的离散计划, 对非线性术语进行明确的 $kämathrm{th\\ $- ord 外推。 我们证明了数字解决方案高索博列夫规范在时间上的一致约束性。 上层约束是无条件的, 也就是说, 不论时间步骤大小如何 。 我们开发了新的代数理论, 校准了近乎最佳的 和 emph{ Explicide} 最大时间步限制, 保证单体 $\ mathbf F$- 调化的能量消散 。