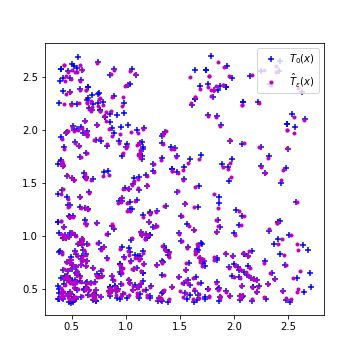
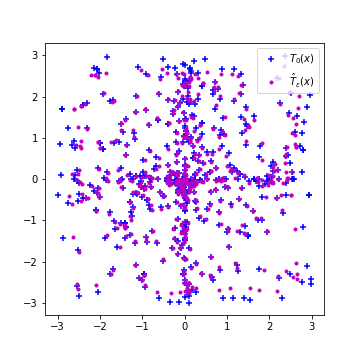
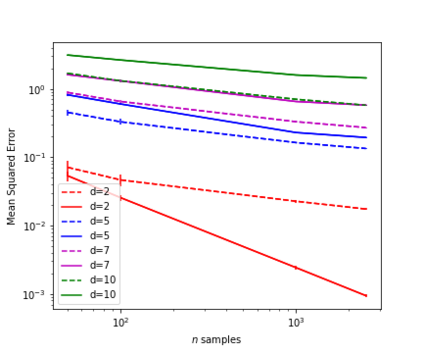
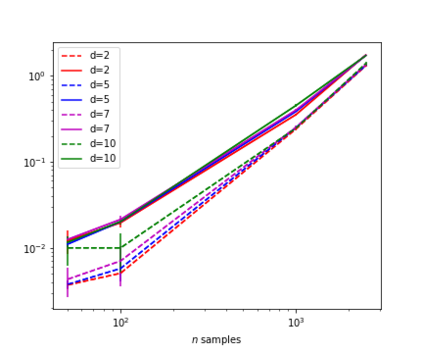
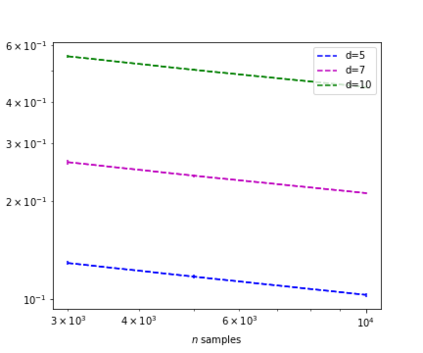
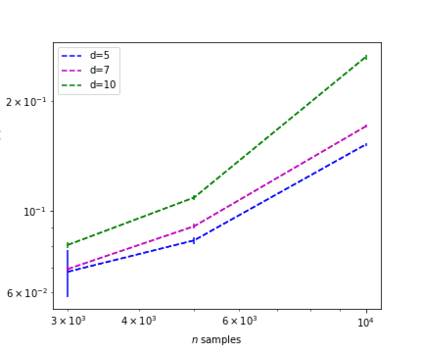
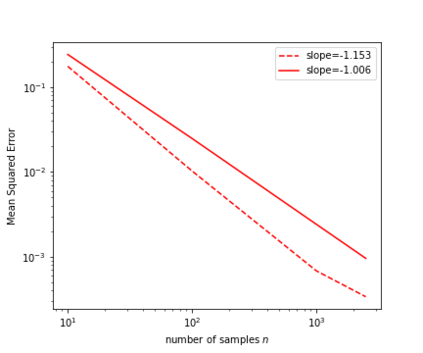
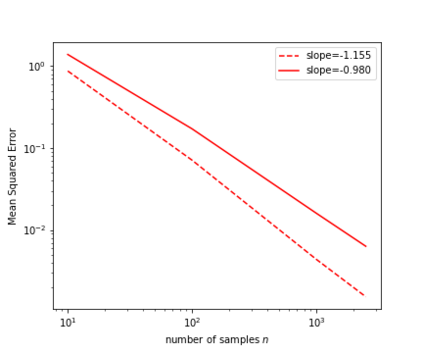
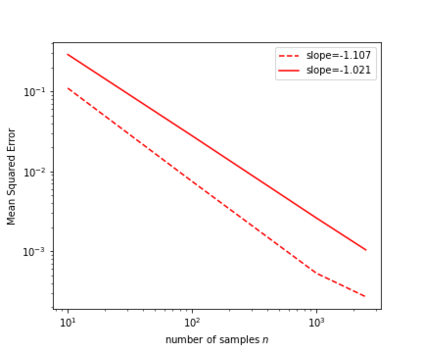
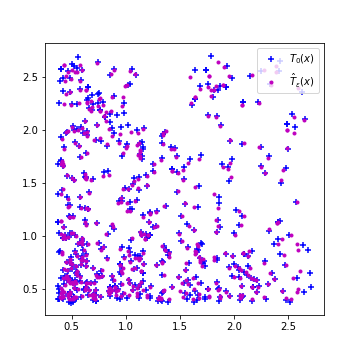
We develop a computationally tractable method for estimating the optimal map between two distributions over $\mathbb{R}^d$ with rigorous finite-sample guarantees. Leveraging an entropic version of Brenier's theorem, we show that our estimator -- the barycentric projection of the optimal entropic plan -- is easy to compute using Sinkhorn's algorithm. As a result, unlike current approaches for map estimation, which are slow to evaluate when the dimension or number of samples is large, our approach is parallelizable and extremely efficient even for massive data sets. Under smoothness assumptions on the optimal map, we show that our estimator enjoys comparable statistical performance to other estimators in the literature, but with much lower computational cost. We showcase the efficacy of our proposed estimator through numerical examples. Our proofs are based on a modified duality principle for entropic optimal transport and on a method for approximating optimal entropic plans due to Pal (2019).
翻译:我们开发了一种可计算、可移动的方法来估计美元=mathbb{R ⁇ d$以上两个分布区间的最佳地图, 并且有严格的有限抽样保证。 我们利用一个正方位版的Brenier 的理论, 我们显示我们的估计器 -- -- 最佳的昆虫计划的以巴中心为中心预测 -- -- 很容易使用Sinkhorn的算法来计算。 因此, 与目前的地图估计方法不同, 目前的地图估计方法在评估样本的大小或数量时速度缓慢, 我们的方法是平行的, 并且极其高效的, 即使是对大量数据集也是如此。 在最佳地图的平稳假设下, 我们显示我们的估计器具有与其他文献中估计器的可比的统计性能, 但是计算成本要低得多。 我们通过数字示例来展示我们提议的估计器的功效。 我们的证明依据的是经过修改的关于最优度运输的双重性原则, 以及由Pal( 2019) 应对的接近最优度计划的方法。