Decomposing Impact on Longitudinal Outcome of Time-varying Covariate into Baseline Effect and Temporal Effect

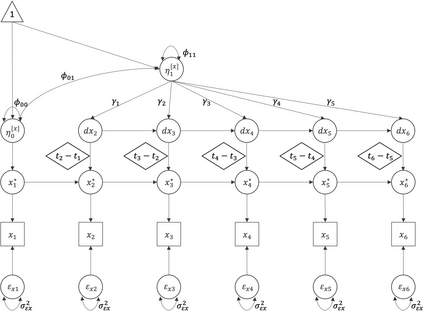
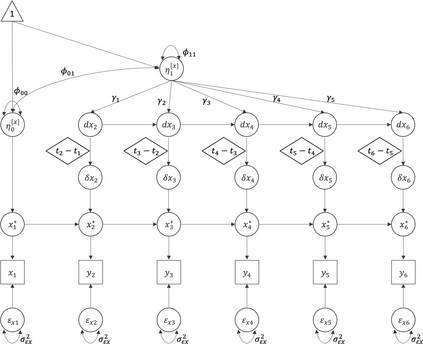
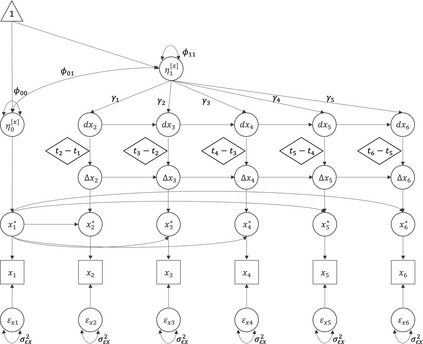
Longitudinal processes are often associated with each other over time; therefore, it is important to investigate the associations among developmental processes and understand their joint development. The latent growth curve model (LGCM) with a time-varying covariate (TVC) provides a method to estimate the TVC's effect on a longitudinal outcome while simultaneously modeling the outcome's change. However, it does not allow the TVC to predict variations in the random growth coefficients. We propose decomposing the TVC's effect into initial trait and temporal states using three methods to address this limitation. In each method, the baseline of the TVC is viewed as an initial trait, and the corresponding effects are obtained by regressing random intercepts and slopes on the baseline value. Temporal states are characterized as (1) interval-specific slopes, (2) interval-specific changes, or (3) changes from the baseline at each measurement occasion, depending on the method. We demonstrate our methods through simulations and real-world data analyses, assuming a linear-linear functional form for the longitudinal outcome. The results demonstrate that LGCMs with a decomposed TVC can provide unbiased and precise estimates with target confidence intervals. We also provide OpenMx and Mplus 8 code for these methods with commonly used linear and nonlinear functions.