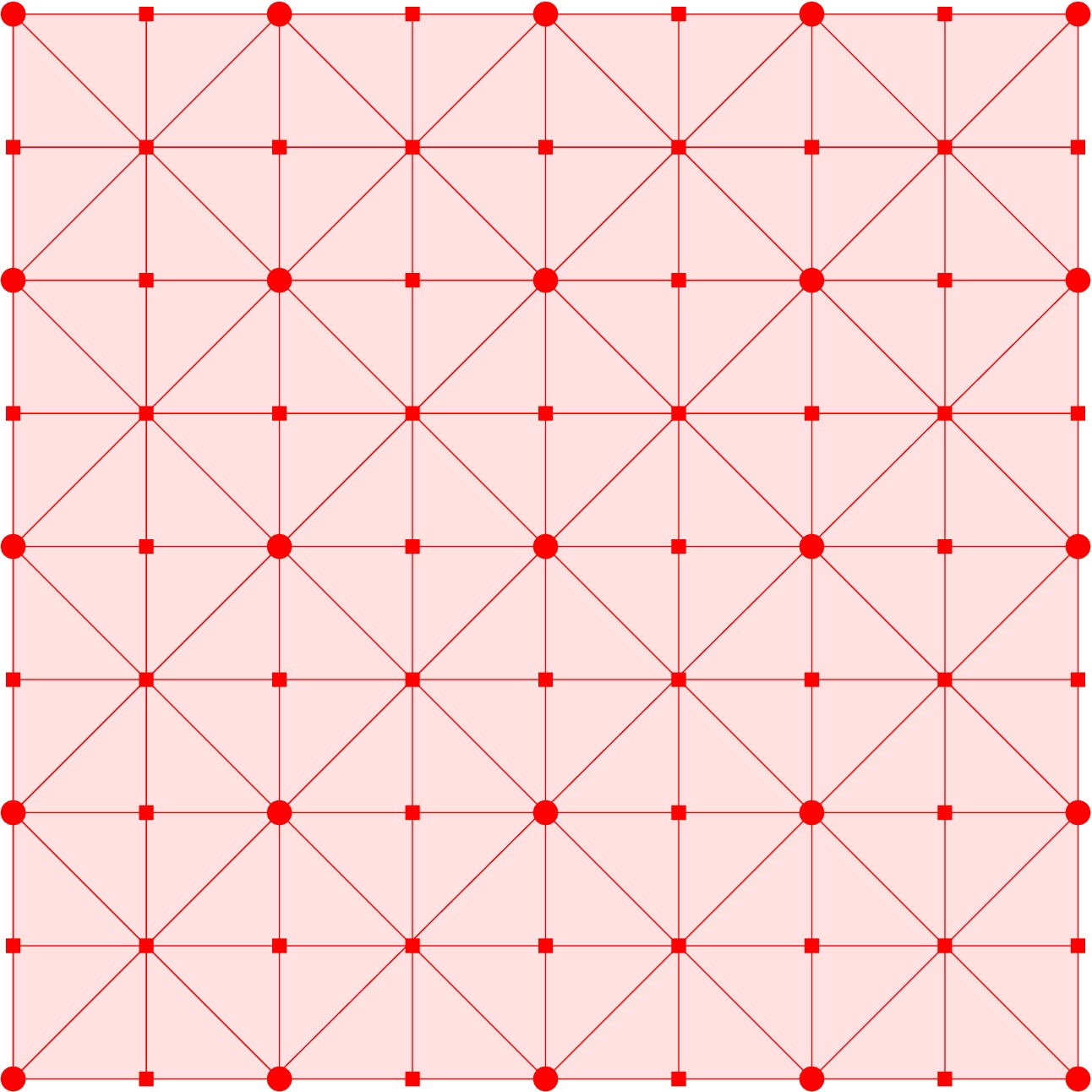
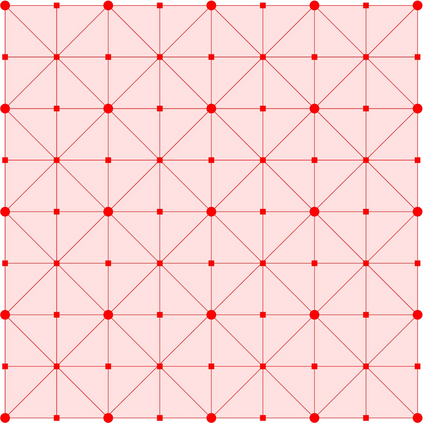
Rips complexes are important structures for analyzing topological features of metric spaces. Unfortunately, generating these complexes is expensive because of a combinatorial explosion in the complex size. For $n$ points in $\mathbb{R}^d$, we present a scheme to construct a $2$-approximation of the filtration of the Rips complex in the $L_\infty$-norm, which extends to a $2d^{0.25}$-approximation in the Euclidean case. The $k$-skeleton of the resulting approximation has a total size of $n2^{O(d\log k +d)}$. The scheme is based on the integer lattice and simplicial complexes based on the barycentric subdivision of the $d$-cube. We extend our result to use cubical complexes in place of simplicial complexes by introducing cubical maps between complexes. We get the same approximation guarantee as the simplicial case, while reducing the total size of the approximation to only $n2^{O(d)}$ (cubical) cells. There are two novel techniques that we use in this paper. The first is the use of acyclic carriers for proving our approximation result. In our application, these are maps which relate the Rips complex and the approximation in a relatively simple manner and greatly reduce the complexity of showing the approximation guarantee. The second technique is what we refer to as scale balancing, which is a simple trick to improve the approximation ratio under certain conditions.
翻译:复杂的裂纹是分析公制空间地形特征的重要结构。 不幸的是, 生成这些复杂点是昂贵的, 因为复杂大小的组合式爆炸。 对于$mathbb{R ⁇ d$的美元点数, 我们提出一个计划, 在$L ⁇ infty$- norm 中, 构建一个以$$ ⁇ infty$- norm为核心的裂纹过滤法, 以2d ⁇ 0. 25美元为基数。 在 Euclidean 案中, 使用美元- a problical Complication 来取代简单复杂的复杂点。 由此产生的近似精度中, $2 ⁇ O( d\log k +d)} 的美元- skeleton 的基数总大小为$2 ⁇ oqual 。 这个方案的基础是整齐整的拉特和简略的基数。 我们的精确度, 我们的精确度和精确度的精确度将比值推算为两个。 我们的精确度 。 在这种精确度中, 我们的精确度中, 我们的精确度中, 我们的精确度是使用这个精确度的计算中, 。