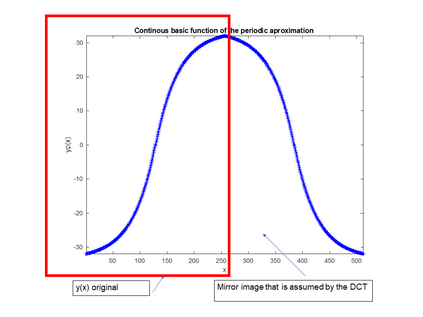
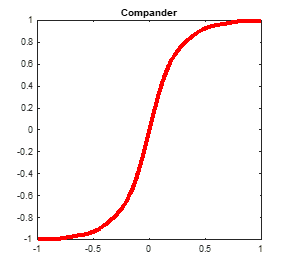
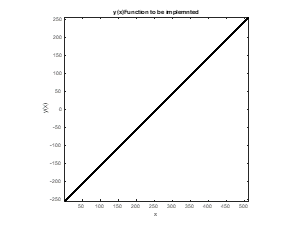
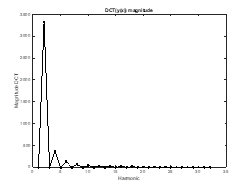
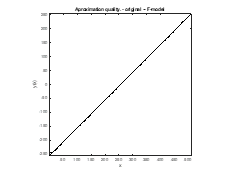
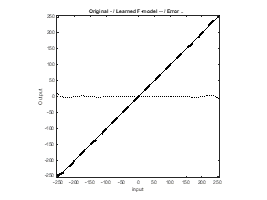
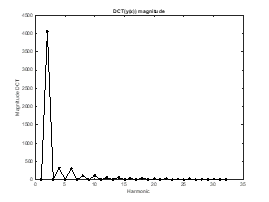
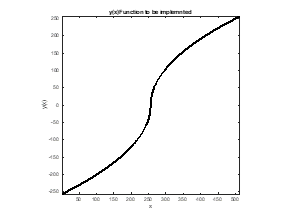
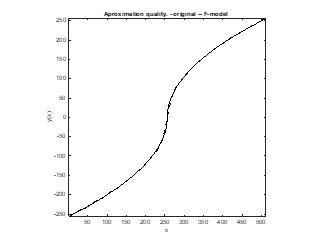
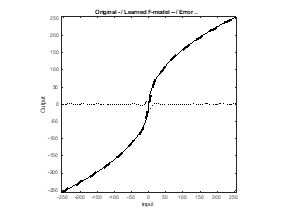
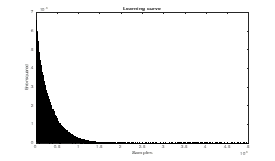
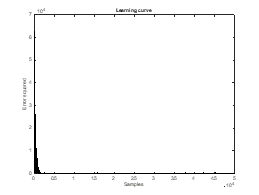
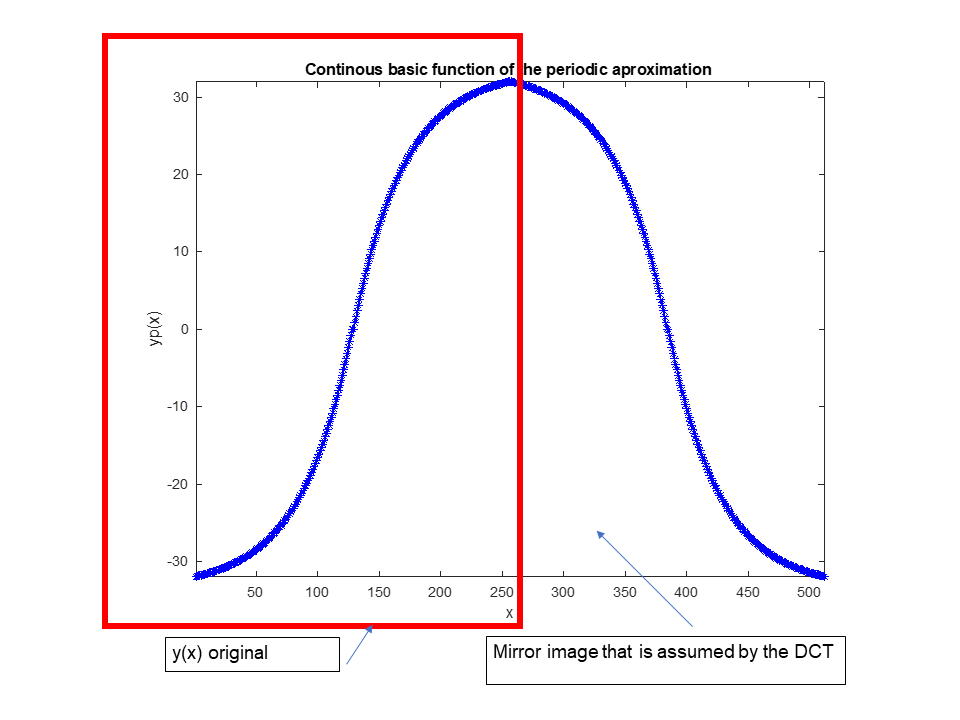
This paper studies the cosine as basis function for the approximation of univariate and continuous functions without memory. This work studies a supervised learning to obtain the approximation coefficients, instead of using the Discrete Cosine Transform (DCT). Due to the finite dynamics and orthogonality of the cosine basis functions, simple gradient algorithms, such as the Normalized Least Mean Squares (NLMS), can benefit from it and present a controlled and predictable convergence time and error misadjustment. Due to its simplicity, the proposed technique ranks as the best in terms of learning quality versus complexity, and it is presented as an attractive technique to be used in more complex supervised learning systems. Simulations illustrate the performance of the approach. This paper celebrates the 50th anniversary of the publication of the DCT by Nasir Ahmed in 1973.
翻译:暂无翻译