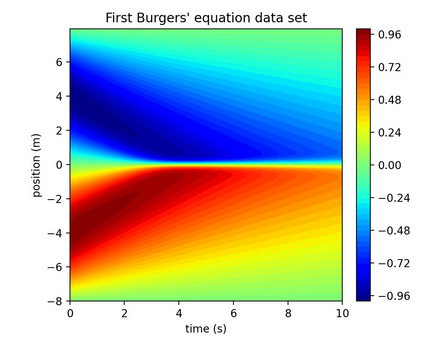
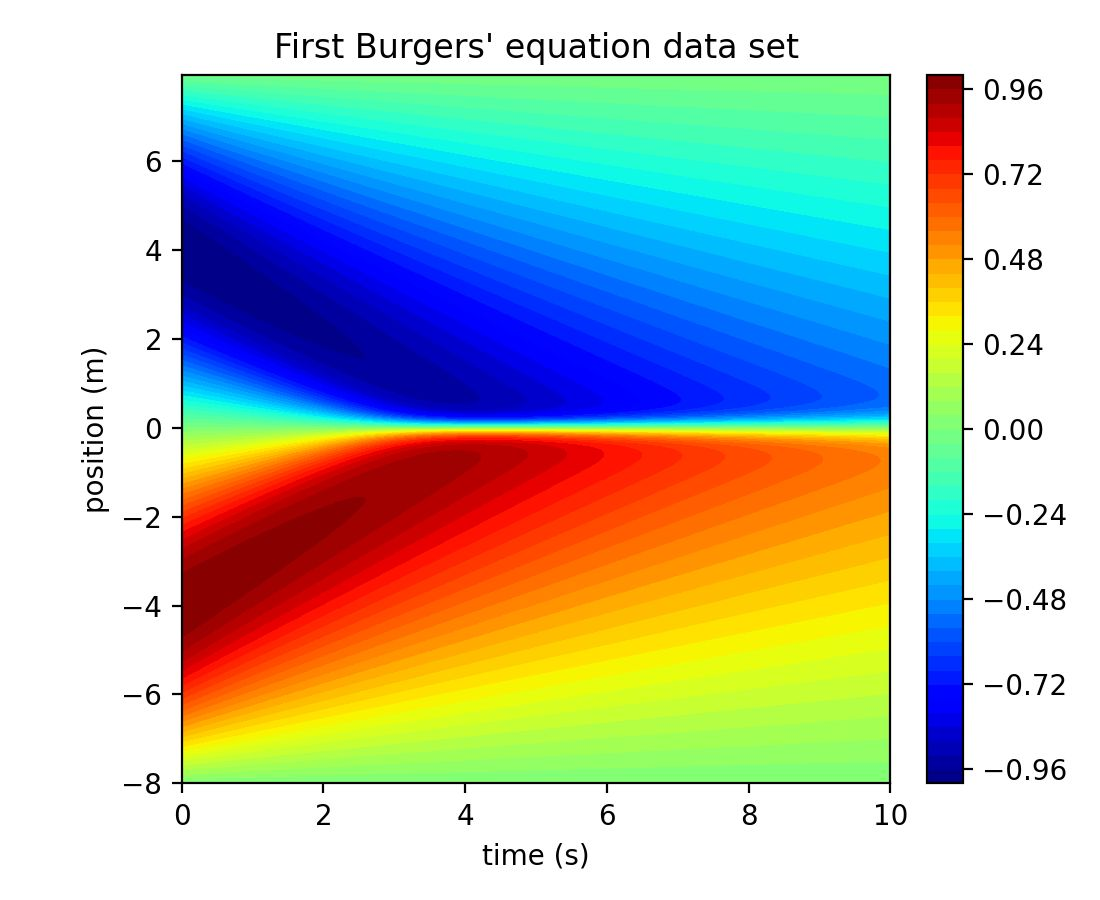
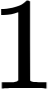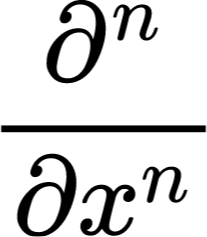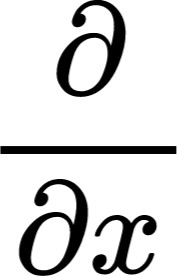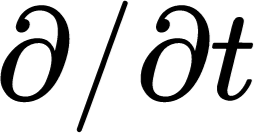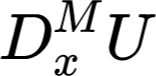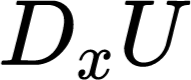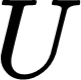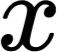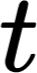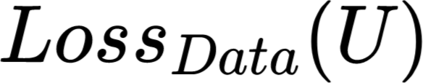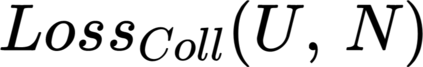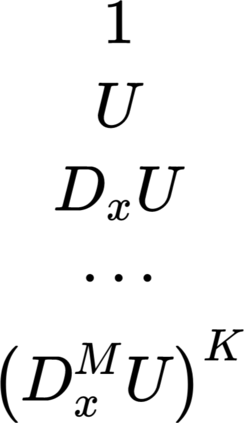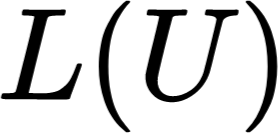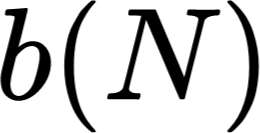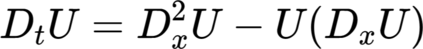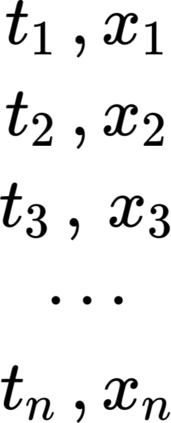PDE discovery shows promise for uncovering predictive models for complex physical systems but has difficulty when measurements are sparse and noisy. We introduce a new approach for PDE discovery that uses two Rational Neural Networks and a principled sparse regression algorithm to identify the hidden dynamics that govern a system's response. The first network learns the system response function, while the second learns a hidden PDE which drives the system's evolution. We then use a parameter-free sparse regression algorithm to extract a human-readable form of the hidden PDE from the second network. We implement our approach in an open-source library called PDE-READ. Our approach successfully identifies the Heat, Burgers, and Korteweg-De Vries equations with remarkable consistency. We demonstrate that our approach is unprecedentedly robust to both sparsity and noise and is, therefore, applicable to real-world observational data.
翻译:PDE发现显示发现复杂物理系统的预测模型的前景,但在测量工作分散和吵闹时有困难。我们引入了PDE发现的新方法,该方法使用两个理性神经网络和一条有原则的稀有回归算法来识别系统响应的隐藏动态。第一个网络学习系统响应功能,而第二个网络则学习一个驱动系统进化的隐藏的PDE。然后我们使用一个无参数的稀有回归算法从第二个网络中提取一个隐藏的PDE的人类可读形式。我们在一个名为PDE-READ的开放源码图书馆中应用了我们的方法。我们的方法成功地以显著的一致性确认了热量、布尔格斯和Korteweg-De Vries等方程式。我们证明我们的方法对空间和噪音都具有前所未有的活力,因此适用于现实世界的观测数据。