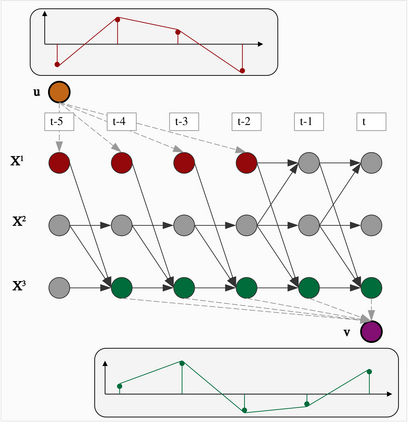
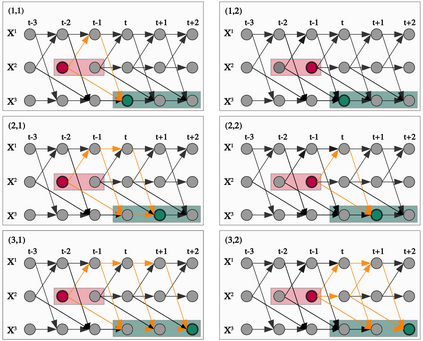
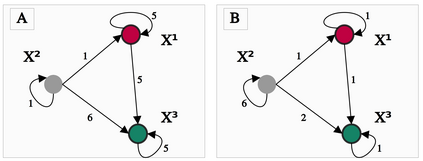
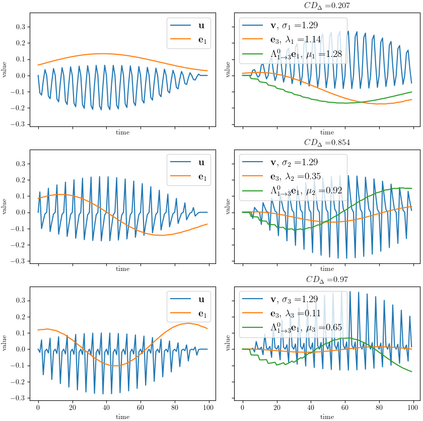
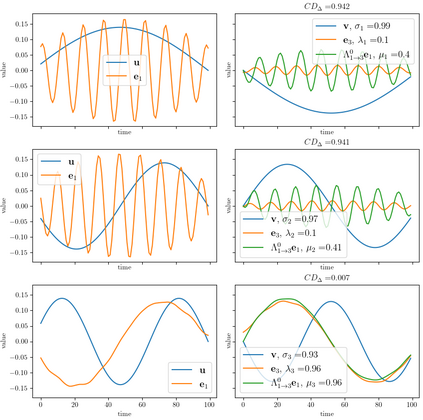
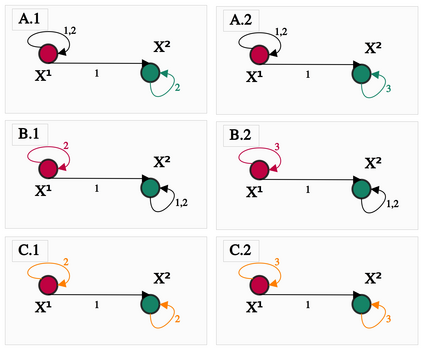
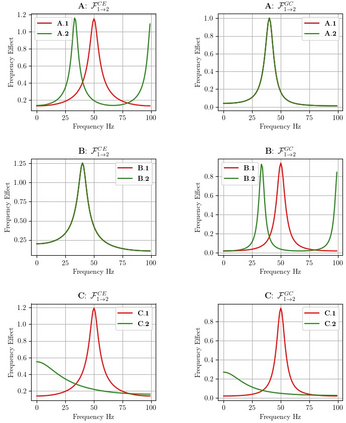
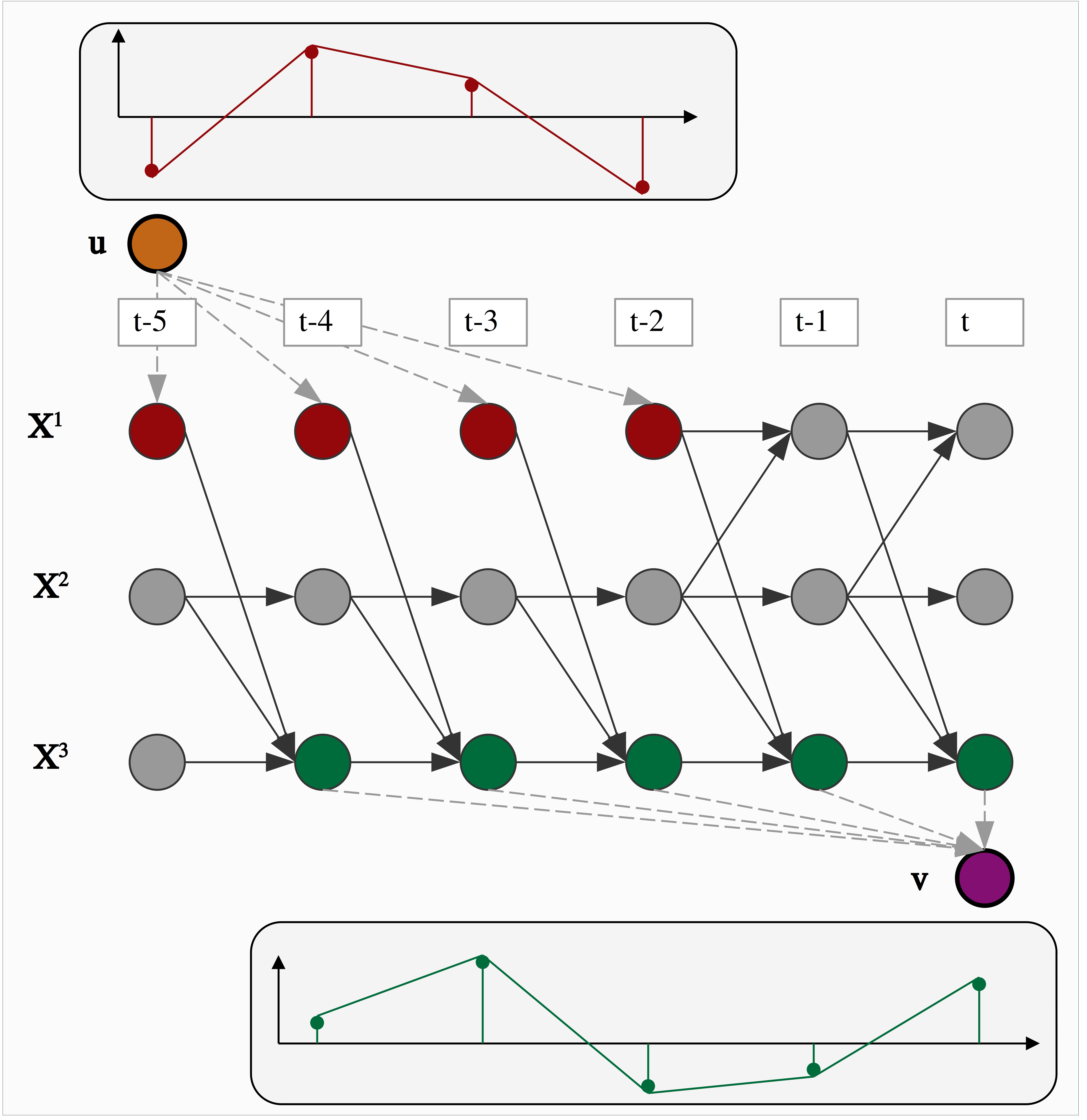
Complex dynamical systems are prevalent in many scientific disciplines. In the analysis of such systems two aspects are of particular interest: 1) the temporal patterns along which they evolve and 2) the underlying causal mechanisms. Time-series representations like discrete Fourier and wavelet transforms have been widely applied in order to obtain insights on the temporal structure of complex dynamical systems. Questions of cause and effect can be formalized in the causal inference framework. We propose an elementary and systematic approach to combine time-series representations with causal inference. Our method is based on a notion of causal effects from a cause on an effect process with respect to a pair of temporal patterns. In particular, our framework can be used to study causal effects in the frequency domain. We will see how our approach compares to the well known Granger Causality in the frequency domain. Furthermore, using a singular value decomposition we establish a representation of how one process drives another over a time-window of specified length in terms of temporal impulse-response patterns. To these we will refer to as Causal Orthogonal Functions (COF), a causal analogue of the temporal patterns derived with covariance-based multivariate Singular Spectrum Analysis (mSSA).
翻译:在许多科学学科中,复杂的动态系统十分普遍。在分析这些系统时,有两个方面特别值得注意:(1) 其演变过程中的时间模式和(2) 内在因果关系机制。时间序列的表示方式,如离散的Fourier和波盘变换,已被广泛应用,以便了解复杂的动态系统的暂时结构。因果关系问题可以在因果推断框架中正式确定。我们提出一种基本和系统的办法,将时间序列的表示方式与因果推断结合起来。我们的方法基于一个原因对一对时间模式的影响过程的因果关系概念。特别是,我们的框架可以用来研究频率领域的因果关系。我们将看到我们的方法如何与频率领域的已知的Granger Causality相比较。此外,我们使用一个单一价值的分解法,确定一个过程如何驱动另一个过程,在时间序列中以特定时间长度的时微反应模式。我们将这些方法称为Causal Orthoal condiction 函数(COF),即以星系图分析为基础的时空模型的因果类比。