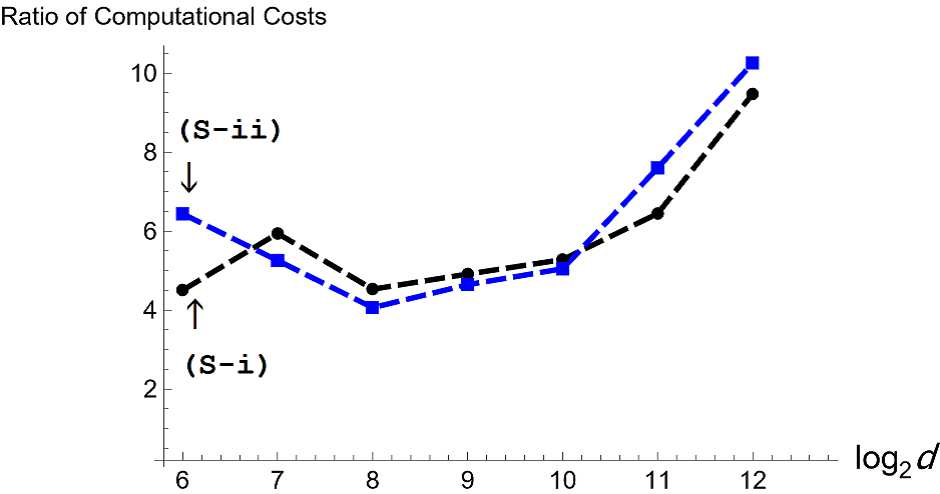
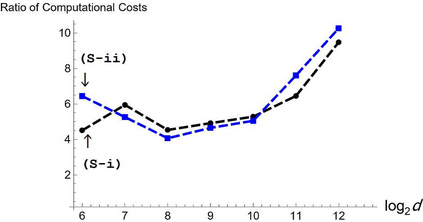
Sparse principal component analysis (SPCA) methods have proven to efficiently analyze high-dimensional data. Among them, threshold-based SPCA (TSPCA) is computationally more cost-effective than regularized SPCA, based on L1 penalties. We herein present an investigation of the efficacy of TSPCA for high-dimensional data settings and illustrate that, for a suitable threshold value, TSPCA achieves satisfactory performance for high-dimensional data. Thus, the performance of the TSPCA depends heavily on the selected threshold value. To this end, we propose a novel thresholding estimator to obtain the principal component (PC) directions using a customized noise-reduction methodology. The proposed technique is consistent under mild conditions, unaffected by threshold values, and therefore yields more accurate results quickly at a lower computational cost. Furthermore, we explore the shrinkage PC directions and their application in clustering high-dimensional data. Finally, we evaluate the performance of the estimated shrinkage PC directions in actual data analyses.
翻译:暂无翻译