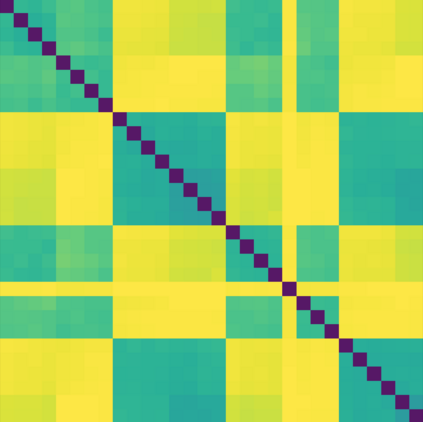
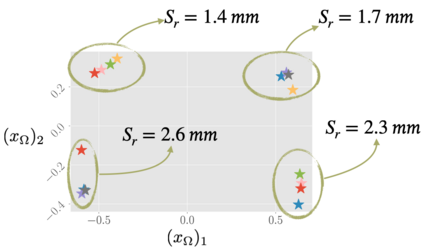
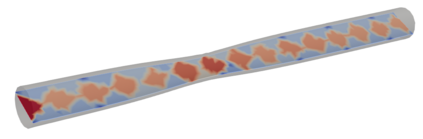
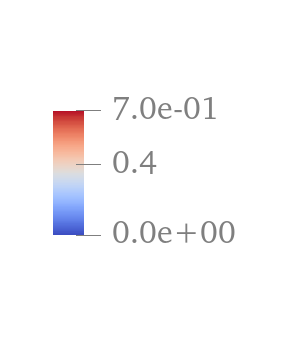
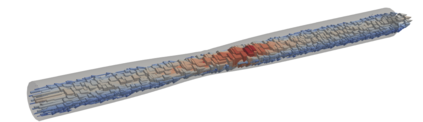
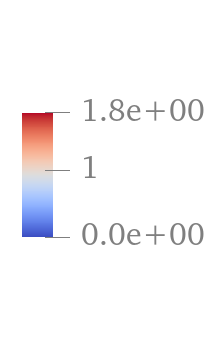
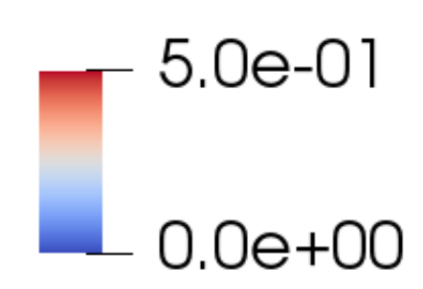
We develop a mathematical and numerical framework to solve state estimation problems for applications that present variations in the shape of the spatial domain. This situation arises typically in a biomedical context where inverse problems are posed on certain organs or portions of the body which inevitably involve morphological variations. If one wants to provide fast reconstruction methods, the algorithms must take into account the geometric variability. We develop and analyze a method which allows to take this variability into account without needing any a priori knowledge on a parametrization of the geometrical variations. For this, we rely on morphometric techniques involving Multidimensional Scaling, and couple them with reconstruction algorithms that make use of reduced model spaces pre-computed on a database of geometries. We prove the potential of the method on a synthetic test problem inspired from the reconstruction of blood flows and quantities of medical interest with Doppler ultrasound imaging.
翻译:我们开发了一个数学和数字框架,以解决在空间领域形态上存在差异的应用的国家估计问题。这种情况通常发生在生物医学背景下,即某些器官或身体部分出现反向问题,不可避免地涉及形态变异。如果想要提供快速重建方法,算法必须考虑到几何变异性。我们开发和分析一种方法,可以将这种变异性考虑在内,而不必事先了解几何变异的近似性。为此,我们依赖涉及多层面增强的测深技术,并将之与利用地理特征数据库中预编的减少的模型空间的重建算法相结合。我们证明了该方法在从重建血液流动和多普勒超声波成像中医疗利益的数量中激发的合成测试问题方面的潜力。