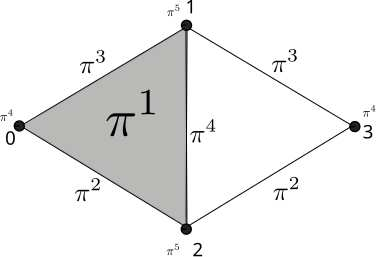
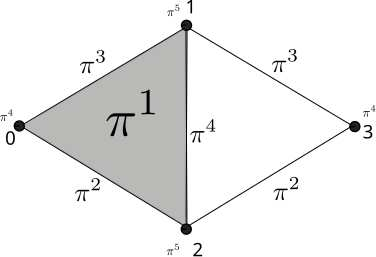
We provide a bottom up construction of torsion generators for weighted homology of a weighted complex over a discrete valuation ring $R=\mathbb{F}[[\pi]]$. This is achieved by starting from a basis for classical homology of the $n$-th skeleton for the underlying complex with coefficients in the residue field $\mathbb{F}$ and then lifting it to a basis for the weighted homology with coefficients in the ring $R$. Using the latter, a bijection is established between $n+1$ and $n$ dimensional simplices whose weight ratios provide the exponents of the $\pi$-monomials that generate each torsion summand in the structure theorem of the weighted homology modules over $R$. We present algorithms that subsume the torsion computation by reducing it to normalization over the residue field of $R$, and describe a Python package we implemented that takes advantage of this reduction and performs the computation efficiently.
翻译:我们提供自下而上推力生成器,用于加权复合体的加权同质,而不是离散估值环[R ⁇ mathbb{F}][[\pi]]]美元。这是从以下一种基础开始的:对基础复合体的美元骨架的典型同质学基础,其残留区系数为$\mathbb{F}美元,然后将其提升为对数值的加权同质学基础。利用后者,在一美元+1美元和一元一元一模之间建立了一个双轨,其重量比率提供了在加权同质单元结构结构中产生每托尔索和超过$的每个托尔索的推力。我们提出算法,通过减少对余量的残余区值的正常化来吸收托尔计算,并描述我们利用这一减值并高效进行计算的一个Python软件包。