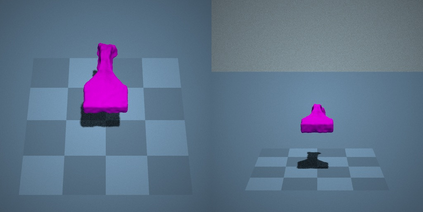
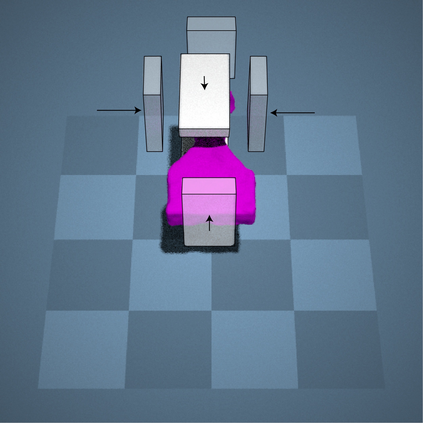
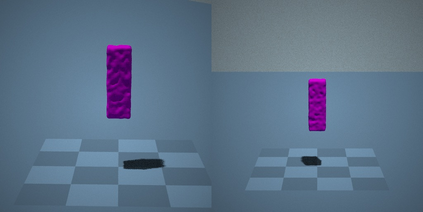
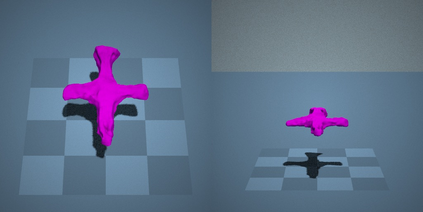
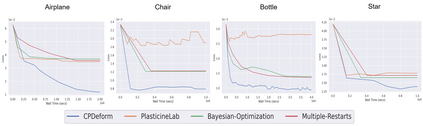
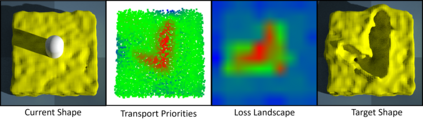
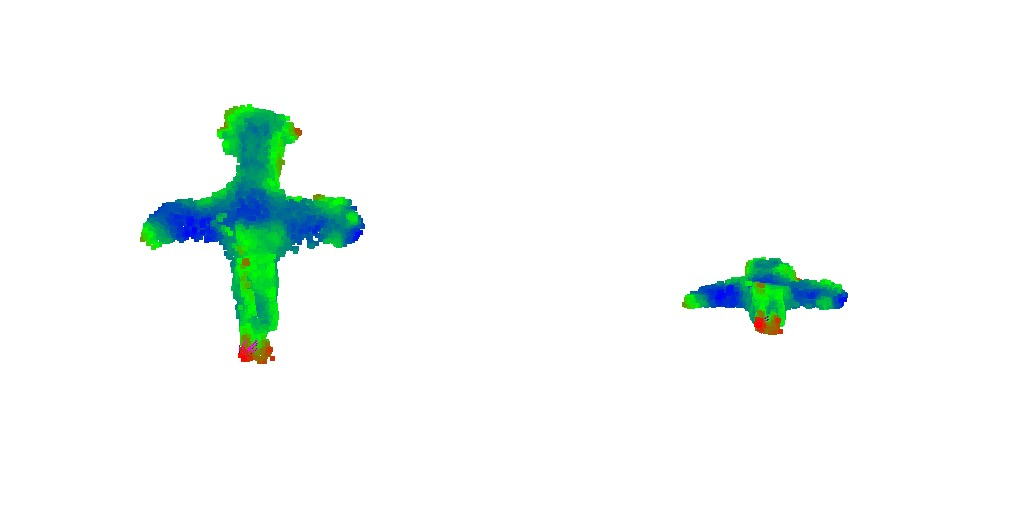
Differentiable physics has recently been shown as a powerful tool for solving soft-body manipulation tasks. However, the differentiable physics solver often gets stuck when the initial contact points of the end effectors are sub-optimal or when performing multi-stage tasks that require contact point switching, which often leads to local minima. To address this challenge, we propose a contact point discovery approach (CPDeform) that guides the stand-alone differentiable physics solver to deform various soft-body plasticines. The key idea of our approach is to integrate optimal transport-based contact points discovery into the differentiable physics solver to overcome the local minima from initial contact points or contact switching. On single-stage tasks, our method can automatically find suitable initial contact points based on transport priorities. On complex multi-stage tasks, we can iteratively switch the contact points of end-effectors based on transport priorities. To evaluate the effectiveness of our method, we introduce PlasticineLab-M that extends the existing differentiable physics benchmark PlasticineLab to seven new challenging multi-stage soft-body manipulation tasks. Extensive experimental results suggest that: 1) on multi-stage tasks that are infeasible for the vanilla differentiable physics solver, our approach discovers contact points that efficiently guide the solver to completion; 2) on tasks where the vanilla solver performs sub-optimally or near-optimally, our contact point discovery method performs better than or on par with the manipulation performance obtained with handcrafted contact points.
翻译:最近,不同物理学最近被展示为解决软体操纵任务的有力工具。然而,当终端效应器最初的接触点是亚最佳的,或者当执行需要切换联络点的多阶段任务时,不同物理学求解器往往被卡住。为了应对这一挑战,我们建议采用接触点发现点发现点方法(CP Deform),引导独立、不同物理求解器变形各种软体塑胶。我们的方法的关键理念是将基于最佳运输的接触点融入不同的物理求解答器中,以克服从初始接触点或接触转换到当地迷你点。在单阶段任务中,我们的方法可以自动找到基于运输优先事项的适合的初步接触点。在复杂的多阶段任务中,我们可以根据运输优先事项反复转换终端效应的接触点。为了评估我们的方法的有效性,我们引入了可塑ineLab-M,将现有的不同物理基准SdaliineLab 扩展到7个具有挑战性的多级软体操作任务。关于单级接触点的广泛实验结果显示:1)在多级的操作点上,在多级的操作点上,在可操作性操作点上,在可操作中,在可操作的操作点上,在多级的操作点上,在可操作点上,在可操作的操作中,在可操作中,在可操作的操作中,在可操作的操作点上,在可操作的操作的操作点上,在可操作的操作点上,在可操作点上,在可操作的操作点上,在可操作下进行更上,在可操作点上,在可操作的操作的操作性上,在可操作的操作性上,在可操作性上,在可操作性上,在可操作性操作性上,在可操作性上,在可操作性上,在可操作的操作的操作性上,在可操作性上,在可操作性上,在可操作性上,在可操作性上,在可操作性上,在可操作性上,在可操作的操作的操作性上,在可操作性上,在可操作性上,在可操作性上进行。