The Max k-Cut Game: On Stable Optimal Colorings

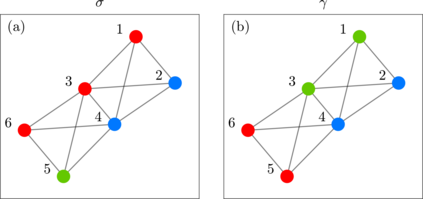
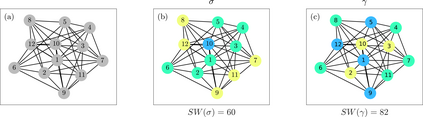
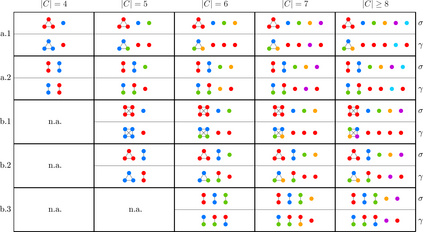
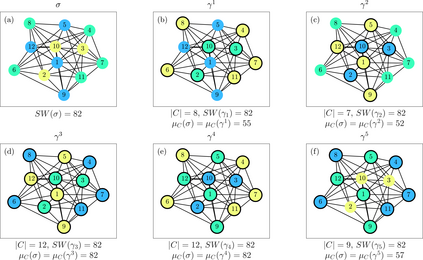
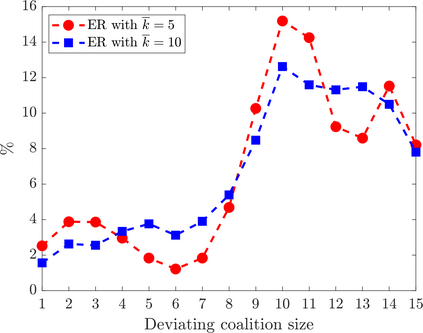
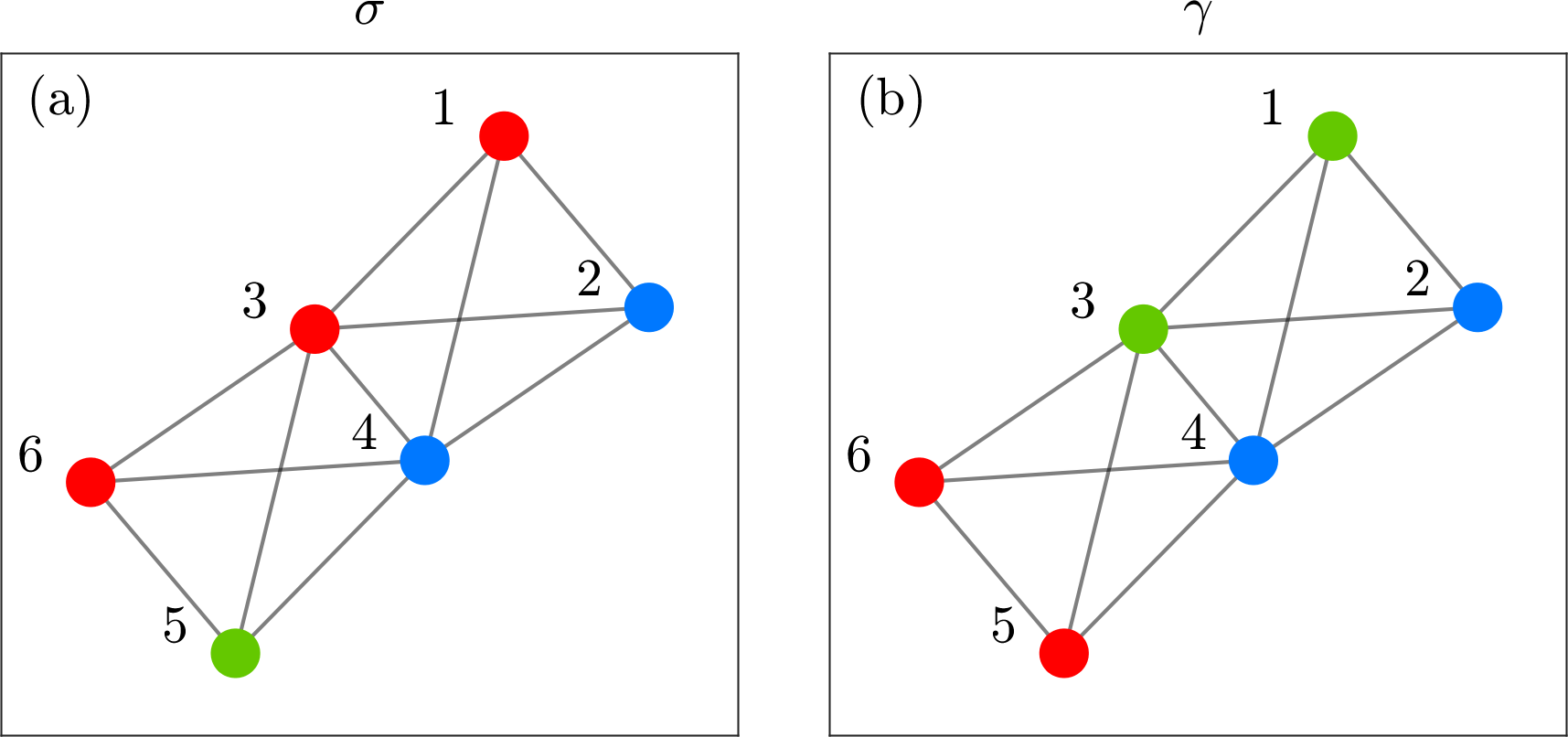
We study the max k-cut game on an undirected and unweighted graph in order to find out whether an optimal solution is also a strong equilibrium. While we do fail to show that, by proving an alternate formula for computing the cut value difference for a strong deviation, we show that optimal solutions are 7-stable equilibria. Furthermore, we prove some properties of minimal subsets with respect to a strong deviation, showing that each of their nodes will deviate towards the color of one of their neighbors and that those subsets induce connected subgraphs.