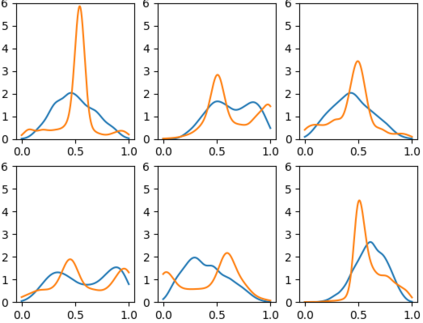
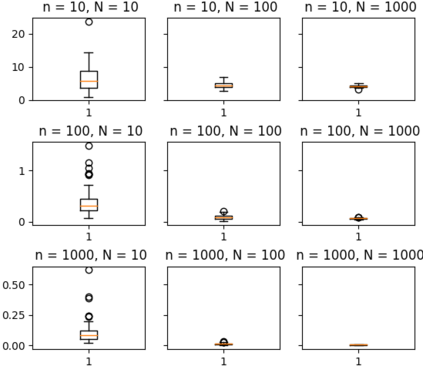
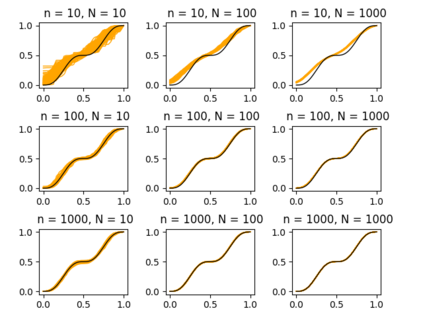
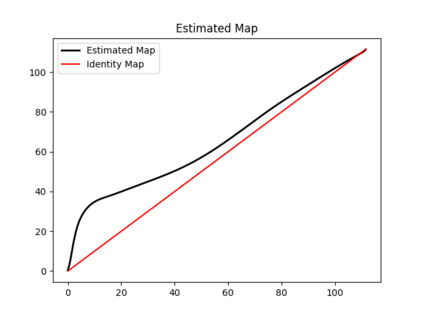
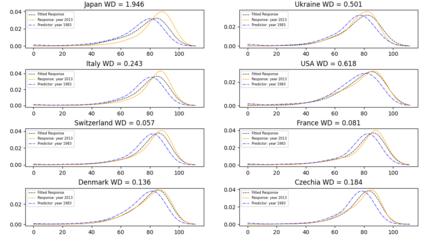
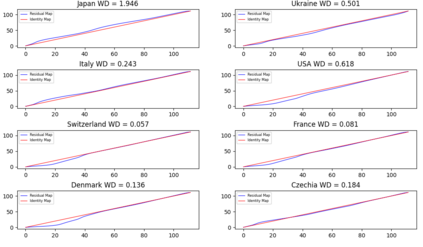
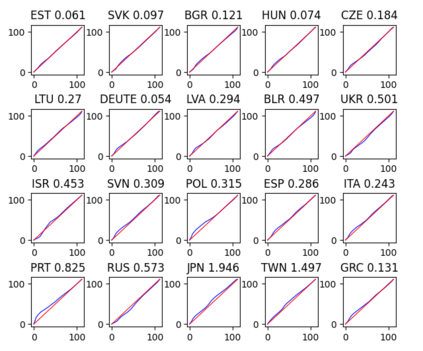
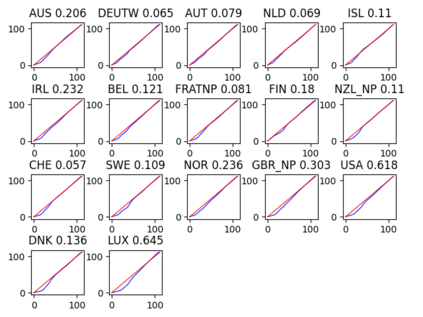
We present a framework for performing regression when both covariate and response are probability distributions on a compact interval $\Omega\subset\mathbb{R}$. Our regression model is based on the theory of optimal transportation and links the conditional Fr\'echet mean of the response distribution to the covariate distribution via an optimal transport map. We define a Fr\'echet-least-squares estimator of this regression map, and establish its consistency and rate of convergence to the true map, under both full and partial observation of the regression pairs. Computation of the estimator is shown to reduce to an isotonic regression problem, and thus our regression model can be implemented with ease. We illustrate our methodology using real and simulated data.
翻译:当共变和反应都是紧凑间隔 $\ Omega\ sub\ mathbb{R}$ 的概率分布时,我们提出了一个进行回归的框架。我们的回归模型基于最佳运输理论,并通过最佳运输图将有条件的Fr\'echet 响应分布值与共变分布值连接起来。我们定义了该回归地图的 Fr\'echet-least-squares 估测器,并在完全和部分观察回归配对下,确定了其一致性和与真实地图的趋同率。测量仪的计算结果显示它会降低到一个同位素回归问题,因此我们的回归模型可以轻松地实施。我们用真实和模拟的数据来说明我们的方法。