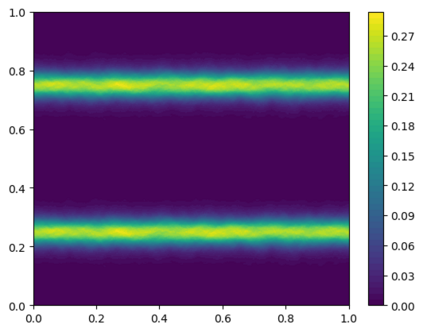
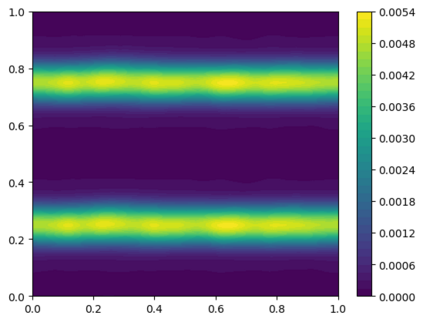
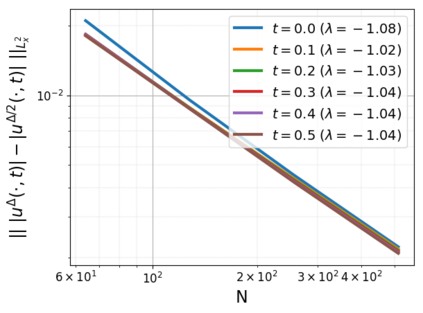
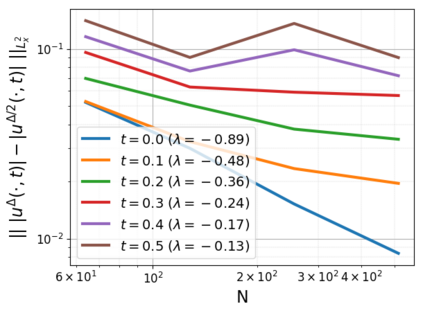
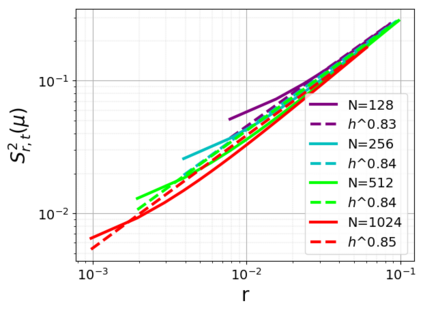
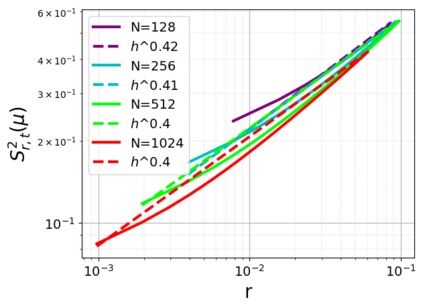
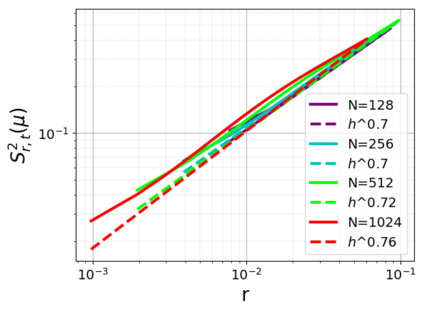
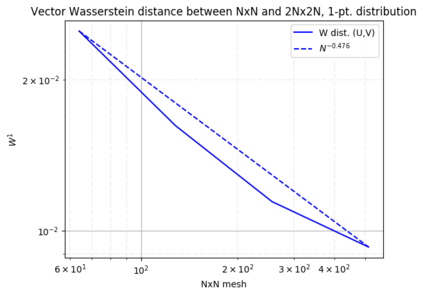
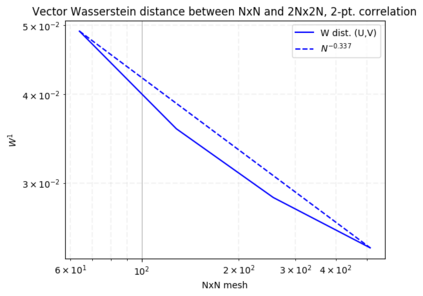
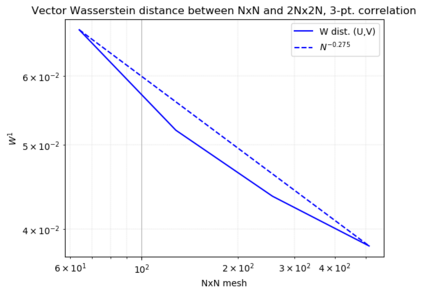
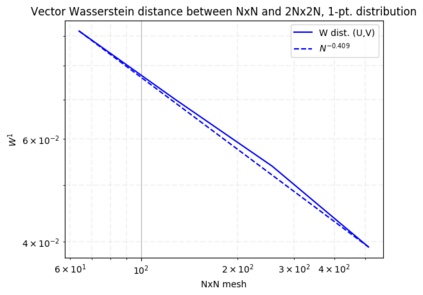
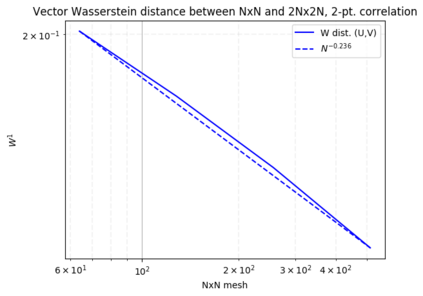
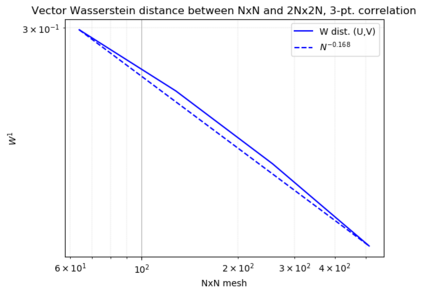
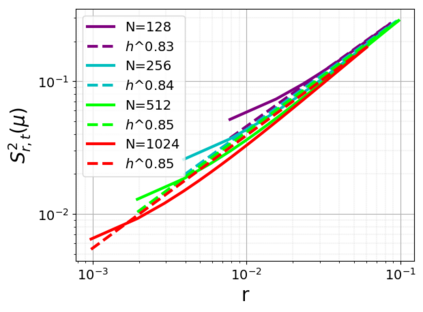
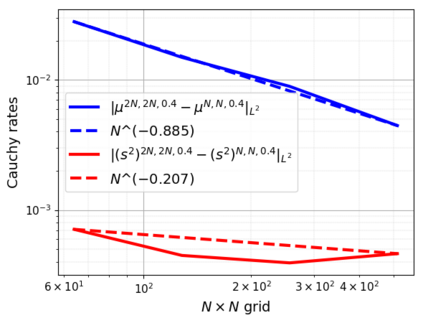
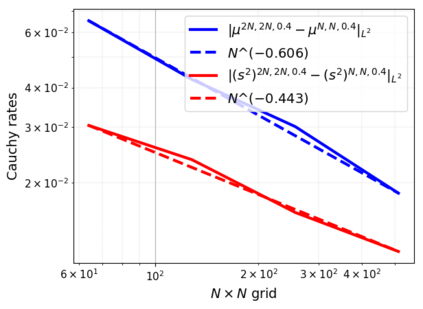
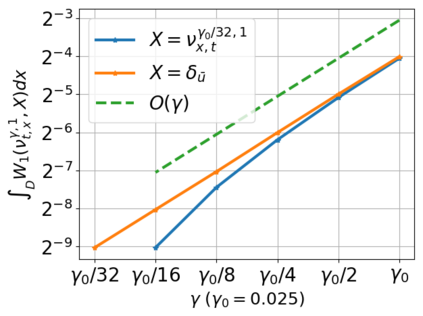
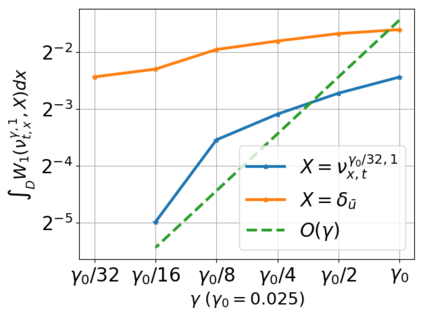
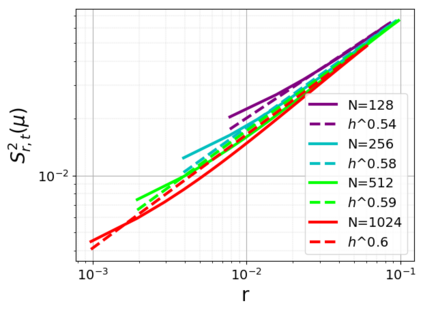
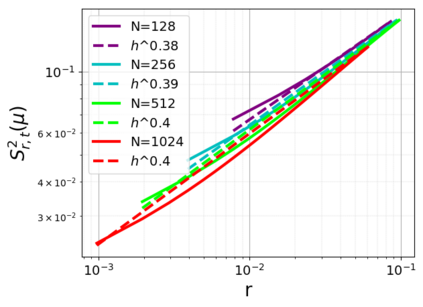
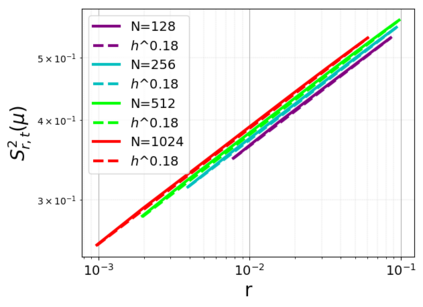
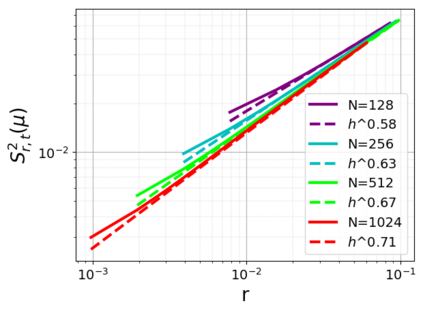
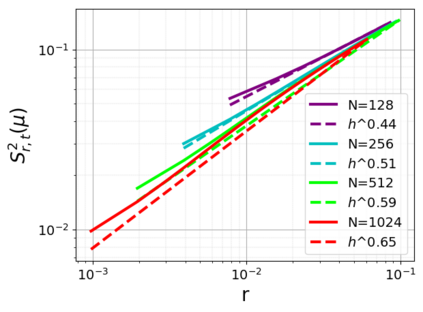
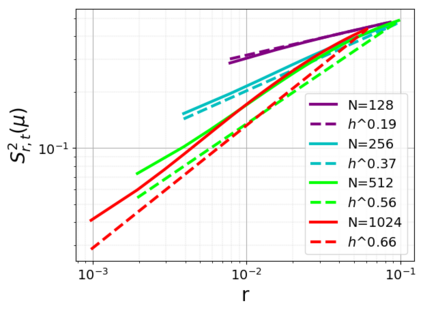
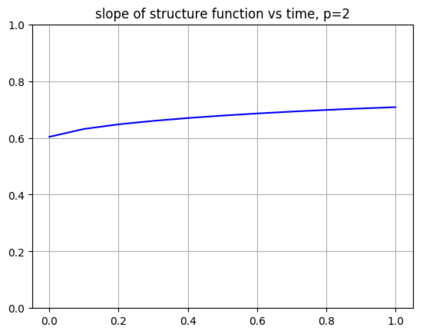
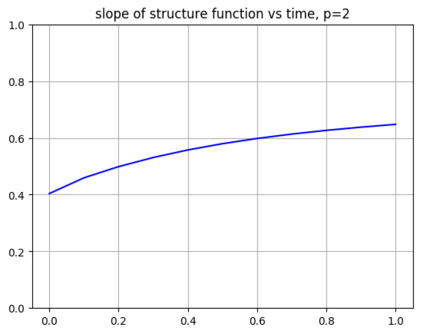
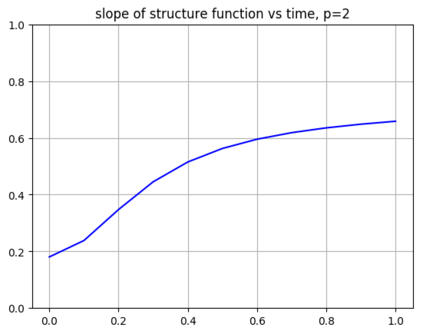
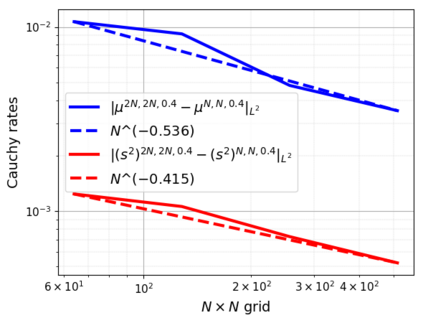
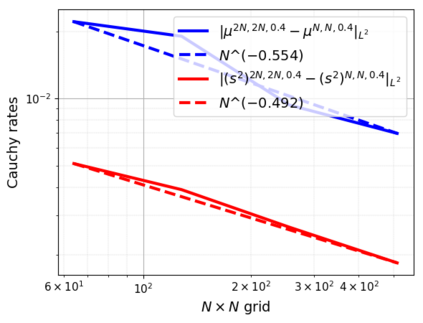
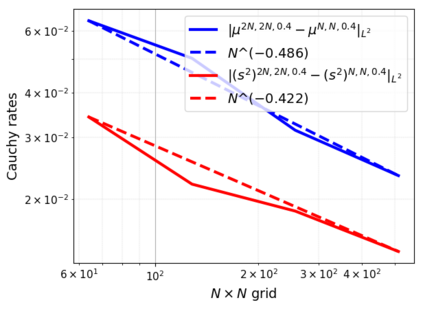
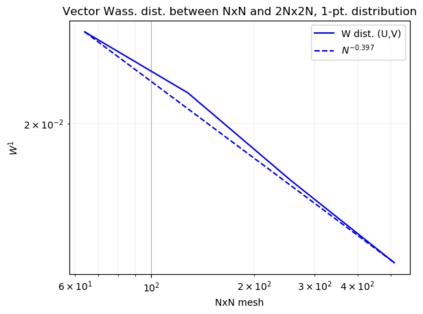
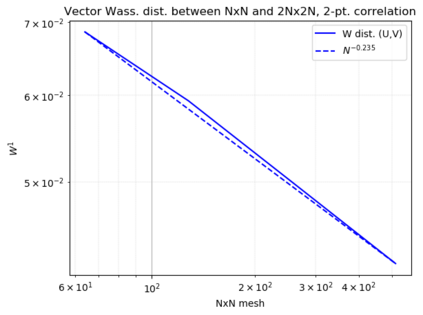
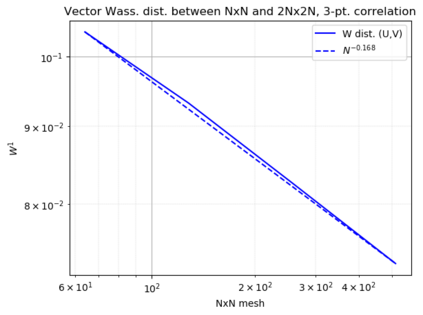
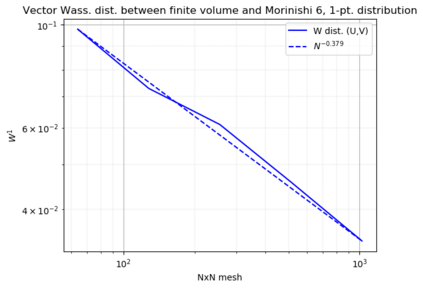
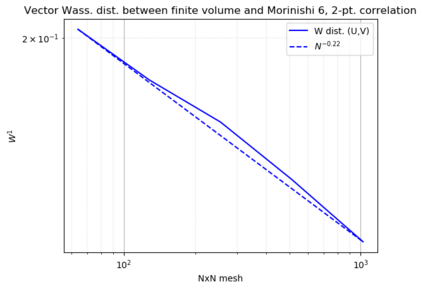
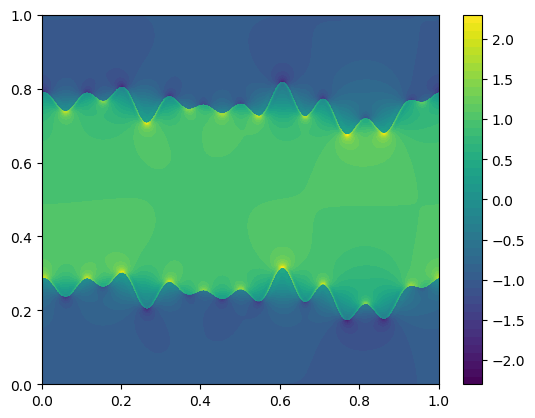
We present an efficient numerical scheme based on Monte Carlo integration to approximate statistical solutions of the incompressible Euler equations. The scheme is based on finite volume methods, which provide a more flexible framework than previously existing spectral methods for the computation of statistical solutions for incompressible flows. This finite volume scheme is rigorously proven to, under experimentally verifiable assumptions, converge in an appropriate topology and with increasing resolution to a statistical solution. The convergence obtained is stronger than that of measure-valued solutions, as it implies convergence of multi-point correlation marginals. We present results of numerical experiments which support the claim that the aforementioned assumptions are very natural, and appear to hold in practice.
翻译:我们提出了一个基于蒙特卡洛一体化的高效数字计划,以近似于无法压缩的 Euler 等式的统计解决办法。这个计划基于数量有限的方法,比以前现有的光谱方法更灵活地计算不可压缩流动的统计解决办法。这个数量有限计划严格地证明,根据实验性可核查的假设,它以适当的地貌和日益清晰的分辨率汇集到一个统计解决办法中。所取得的趋同比量值解决方案的趋同强,因为它意味着多点相关边际的趋同。我们提出了数字实验的结果,支持上述假设非常自然并似乎在实际中存在的说法。