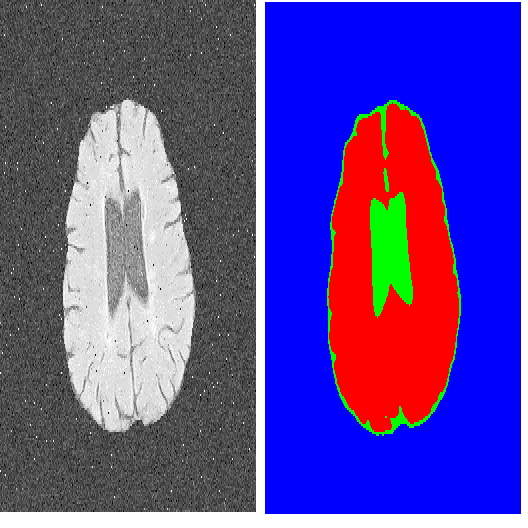
Although a vast body of literature relates to image segmentation methods that use deep neural networks (DNNs), less attention has been paid to assessing the statistical reliability of segmentation results. In this study, we interpret the segmentation results as hypotheses driven by DNN (called DNN-driven hypotheses) and propose a method by which to quantify the reliability of these hypotheses within a statistical hypothesis testing framework. Specifically, we consider a statistical hypothesis test for the difference between the object and background regions. This problem is challenging, as the difference would be falsely large because of the adaptation of the DNN to the data. To overcome this difficulty, we introduce a conditional selective inference (SI) framework -- a new statistical inference framework for data-driven hypotheses that has recently received considerable attention -- to compute exact (non-asymptotic) valid p-values for the segmentation results. To use the conditional SI framework for DNN-based segmentation, we develop a new SI algorithm based on the homotopy method, which enables us to derive the exact (non-asymptotic) sampling distribution of DNN-driven hypothesis. We conduct experiments on both synthetic and real-world datasets, through which we offer evidence that our proposed method can successfully control the false positive rate, has good performance in terms of computational efficiency, and provides good results when applied to medical image data.
翻译:虽然大量文献涉及使用深层神经网络的图像分解方法(DNNs),但对评估分解结果的统计可靠性没有给予足够的重视。在本研究中,我们将分解结果解释为由DNN(称为DNN驱动的假设)驱动的假设(称为DNN驱动的假设),并提出在统计假设测试框架内量化这些假设的可靠性的方法。具体地说,我们考虑对对象和背景区域之间的差异进行统计假设测试。由于DNN对数据进行了调整,这一问题具有挑战性,差异会大得不实。为了克服这一困难,我们引入了一个有条件的选择性推断框架 -- -- 数据分解假设(称为DNN)驱动的假设(称为DNN(D-N)驱动的假设)驱动的假设的新的统计推论框架 -- -- 近来引起相当关注的新的数据分解假设 -- -- 来计算这些假设结果的可靠性。为了使用基于DNNNE的分解的有条件的SI框架,我们开发了一个新的SI算法,因为这种差异会大得不实。为了克服这一困难,我们引入了一种有条件的(非抽象的)选择性)选择性推算法,因此,我们引入了一种有条件的分算法的分算法,我们得以在对DNNNNNP-M-M-S-S-S-S-C-Cx的模型的模型的模型的模型的模型的模型的精确性数据进行了正确的分析结果的模型的模型的精确的模拟法中提供了我们提出的正确分析法。