PSpan:Mining Frequent Subnets of Petri Nets

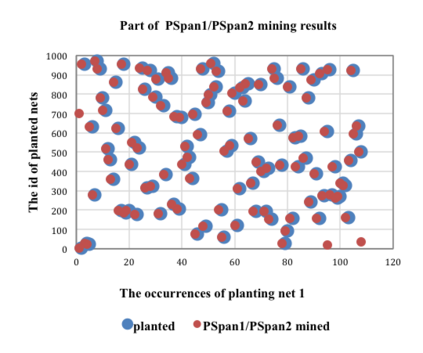
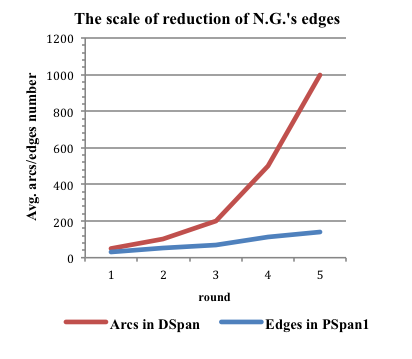
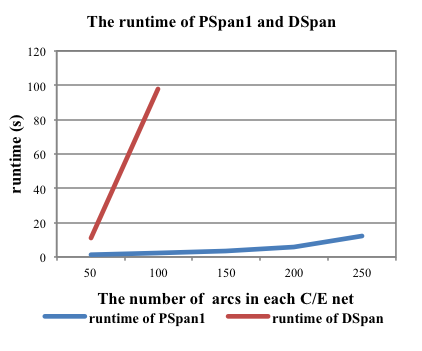
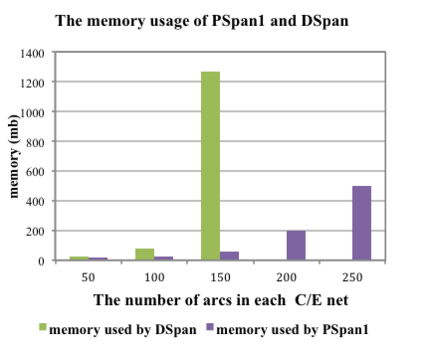
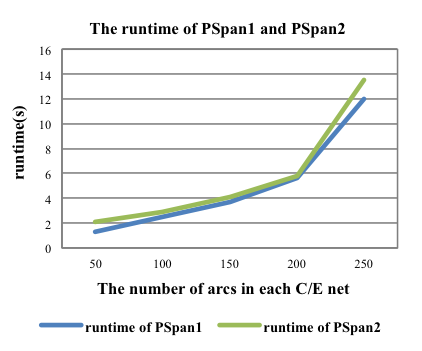
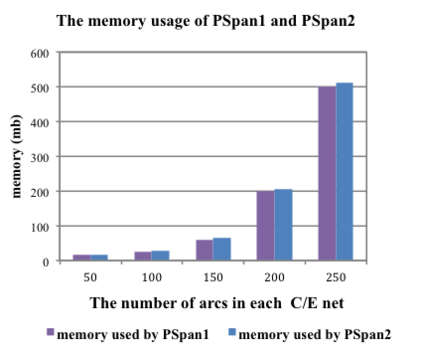
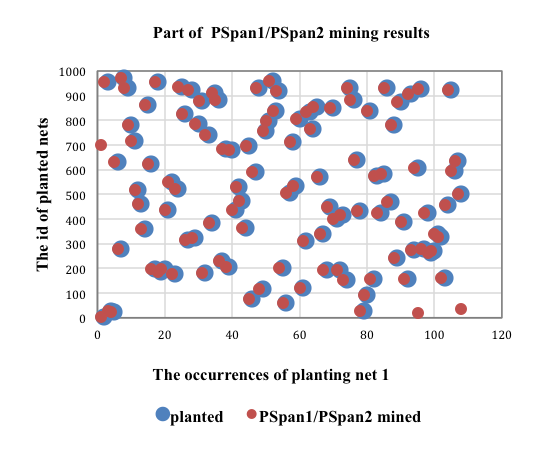
This paper proposes for the first time an algorithm PSpan for mining frequent complete subnets from a set of Petri nets. We introduced the concept of complete subnets and the net graph representation. PSpan transforms Petri nets in net graphs and performs sub-net graph mining on them, then transforms the results back to frequent subnets. PSpan follows the pattern growth approach and has similar complexity like gSpan in graph mining. Experiments have been done to confirm PSpan's reliability and complexity. Besides C/E nets, it applies also to a set of other Petri net subclasses.