Quantum soft-covering lemma with applications to rate-distortion coding, resolvability and identification via quantum channels

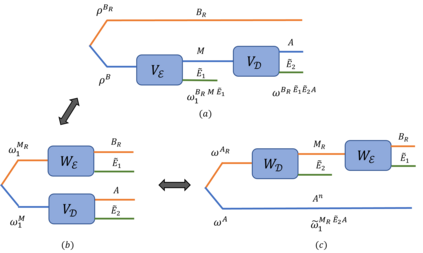
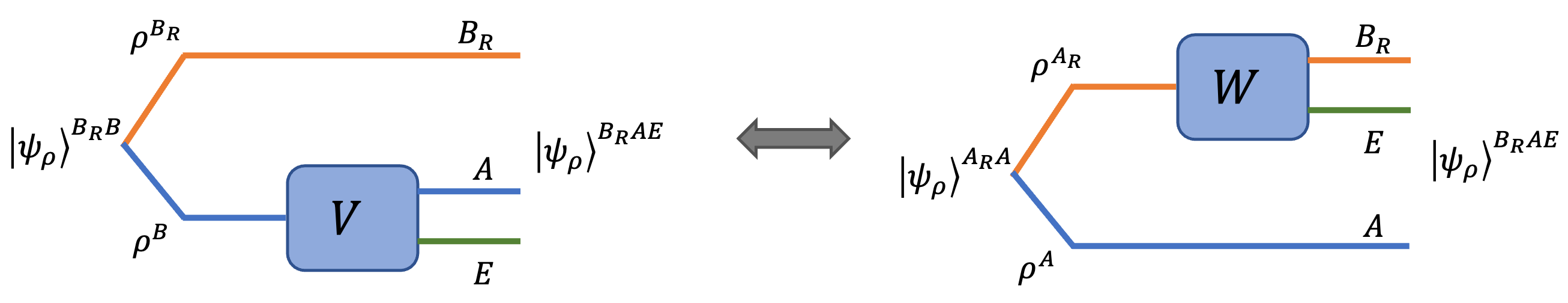
We propose a quantum soft-covering problem for a given general quantum channel and one of its output states, which consists in finding the minimum rank of an input state needed to approximate the given channel output. We then prove a one-shot quantum covering lemma in terms of smooth min-entropies by leveraging decoupling techniques from quantum Shannon theory. This covering result is shown to be equivalent to a coding theorem for rate distortion under a posterior (reverse) channel distortion criterion [Atif, Sohail, Pradhan, arXiv:2302.00625]. Both one-shot results directly yield corollaries about the i.i.d. asymptotics, in terms of the coherent information of the channel. The power of our quantum covering lemma is demonstrated by two additional applications: first, we formulate a quantum channel resolvability problem, and provide one-shot as well as asymptotic upper and lower bounds. Secondly, we provide new upper bounds on the unrestricted and simultaneous identification capacities of quantum channels, in particular separating for the first time the simultaneous identification capacity from the unrestricted one, proving a long-standing conjecture of the last author.