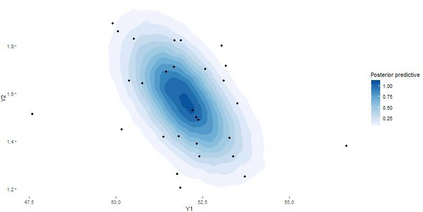
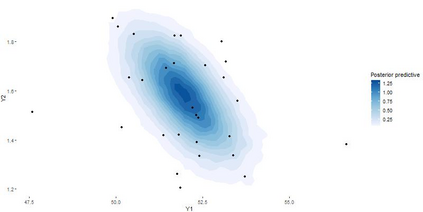
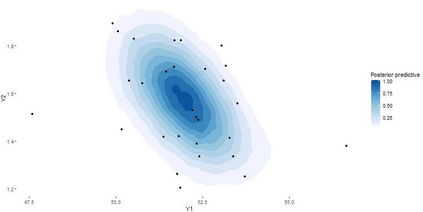
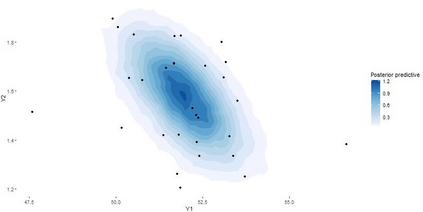
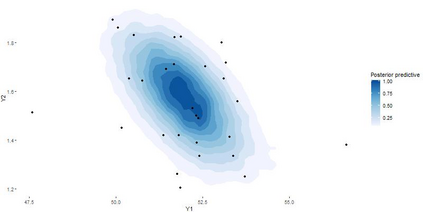
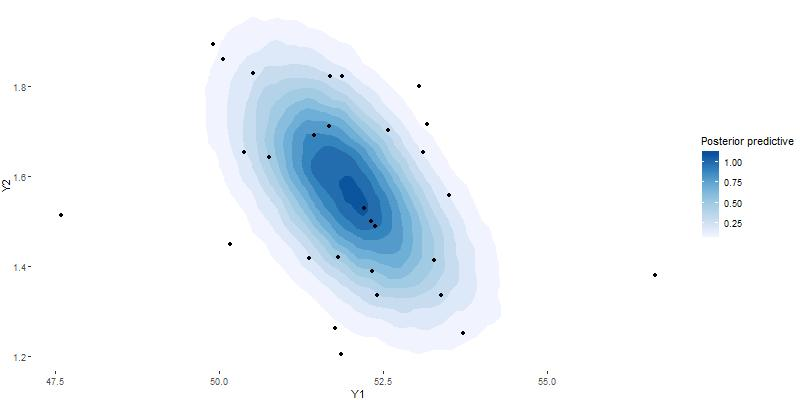
Stochastic inverse problems are typically encountered when it is wanted to quantify the uncertainty affecting the inputs of computer models. They consist in estimating input distributions from noisy, observable outputs, and such problems are increasingly examined in Bayesian contexts where the targeted inputs are affected by stochastic uncertainties. In this regard, a stochastic input can be qualified as meaningful if it explains most of the output uncertainty. While such inverse problems are characterized by identifiability conditions, constraints of "signal to noise", that can formalize this meaningfulness, should be accounted for within the definition of the model, prior to inference. This article investigates the possibility of forcing a solution to be meaningful in the context of parametric uncertainty quantification, through the tools of global sensitivity analysis and information theory (variance, entropy, Fisher information). Such forcings have mainly the nature of constraints placed on the input covariance, and can be made explicit by considering linear or linearizable models. Simulated experiments indicate that, when injected into the modeling process, these constraints can limit the influence of measurement or process noise on the estimation of the input distribution, and let hope for future extensions in a full non-linear framework, for example through the use of linear Gaussian mixtures.
翻译:暂无翻译