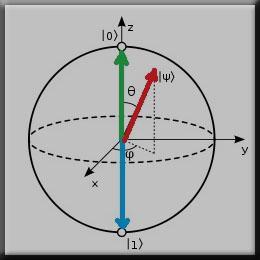
This paper demonstrates that some non-classical models of human decision-making can be run successfully as circuits on quantum computers. Since the 1960s, many observed cognitive behaviors have been shown to violate rules based on classical probability and set theory. For example, the order in which questions are posed in a survey affects whether participants answer 'yes' or 'no', so the population that answers 'yes' to both questions cannot be modeled as the intersection of two fixed sets. It can, however, be modeled as a sequence of projections carried out in different orders. This and other examples have been described successfully using quantum probability, which relies on comparing angles between subspaces rather than volumes between subsets. Now in the early 2020s, quantum computers have reached the point where some of these quantum cognitive models can be implemented and investigated on quantum hardware, by representing the mental states in qubit registers, and the cognitive operations and decisions using different gates and measurements. This paper develops such quantum circuit representations for quantum cognitive models, focusing particularly on modeling order effects and decision-making under uncertainty. The claim is not that the human brain uses qubits and quantum circuits explicitly (just like the use of Boolean set theory does not require the brain to be using classical bits), but that the mathematics shared between quantum cognition and quantum computing motivates the exploration of quantum computers for cognition modeling. Key quantum properties include superposition, entanglement, and collapse, as these mathematical elements provide a common language between cognitive models, quantum hardware, and circuit implementations.
翻译:该论文展示了一些非经典人类决策模型可以在量子计算机上成功地运行为电路。自20世纪60年代以来,许多观察到的认知行为已被证明违反基于经典概率和集合论的规则。例如,在调查中提出的问题顺序影响参与者回答“是”或“否”的问题,因此回答两个问题的人口不能被建模为两个固定集合的交集。然而,它可以被建模为不同顺序进行的一系列投影。这和其他例子都可以用量子概率成功地描述,该概率依赖于比较子空间之间的角度,而不是在子集之间的体积。现在在2020年代初,量子计算机已经达到了一些这些量子认知模型可以在量子硬件上实施和研究的地步,通过在qubit寄存器中表示心理状态,并使用不同的门和测量表示认知操作和决策。本文为量子认知模型开发了这样的量子电路表示,重点关注建模顺序效应和不确定性下的决策。宣称的并不是人脑明确使用量子比特和量子电路(就像使用布尔集合论并不要求大脑使用经典比特一样),而是量子认知和量子计算之间共享的数学语言促进了探索对认知建模进行量子计算。关键的量子属性包括叠加、纠缠和坍缩,因为这些数学元素为认知模型、量子硬件和电路实现提供了一种常见的语言。