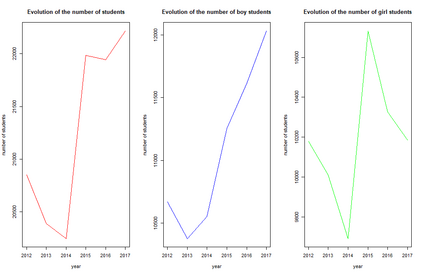
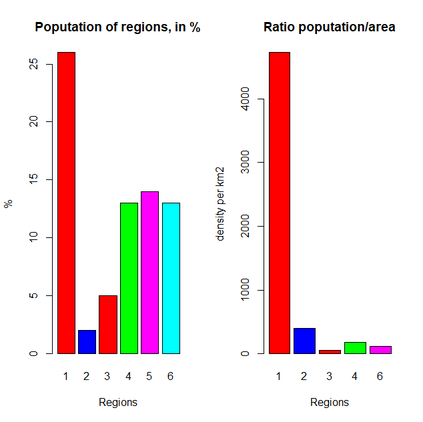
In this paper we want to find a statistical rule that assigns a passing or failing grade to students who undertook at least three exams out of four in a national exam, instead of completely dismissing them students. While it is cruel to declare them as failing, especially if the reason for their absence it not intentional, they should have demonstrated enough merit in the three exams taken to deserve a chance to be declared passing. We use a special classification method and nearest neighbors methods based on the average grade and on the most modal grade to build a statistical rule in a supervised learning process. The study is built on the national GABECE educational data which is a considerable data covering seven years and all the six regions of the Gambia.
翻译:在这份文件中,我们想找到一条统计规则,规定在全国考试中,每四人中至少有三次考试的成绩或不及格的学生,而不是完全开除他们;虽然宣布他们失败是残酷的,特别是如果他们缺席的原因不是故意的,但他们本应在三次考试中表现出足够的功绩,值得宣布考试通过;我们根据平均年级和最典型的年级,使用一种特殊的分类方法和最近的邻里方法,在有监督的学习过程中建立统计规则;研究以冈比亚GABECE国家教育数据为基础,这是冈比亚7年和所有6个地区的大量数据。