Singular value distribution of dense random matrices with block Markovian dependence

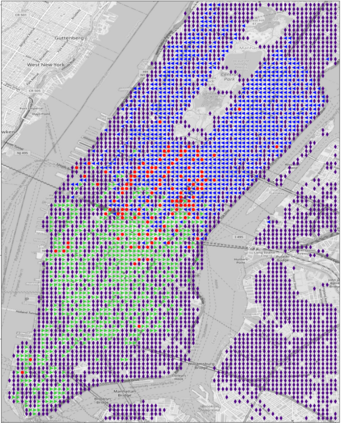
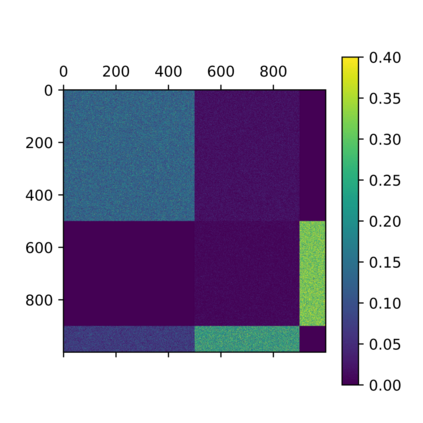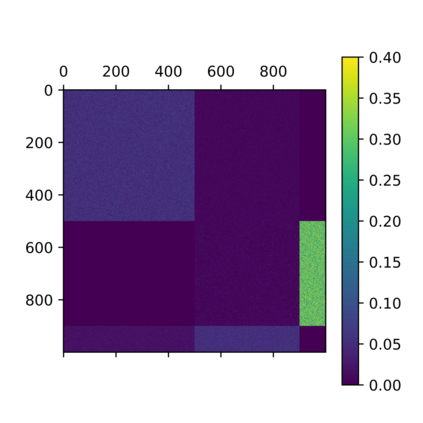A block Markov chain is a Markov chain whose state space can be partitioned into a finite number of clusters such that the transition probabilities only depend on the clusters. Block Markov chains thus serve as a model for Markov chains with communities. This paper establishes limiting laws for the singular value distributions of the empirical transition matrix and empirical frequency matrix associated to a sample path of the block Markov chain whenever the length of the sample path is $\Theta(n^2)$ with $n$ the size of the state space. The proof approach is split into two parts. First, we introduce a class of symmetric random matrices with dependence called approximately uncorrelated random matrices with variance profile. We establish their limiting eigenvalue distributions by means of the moment method. Second, we develop a coupling argument to show that this general-purpose result applies to block Markov chains.