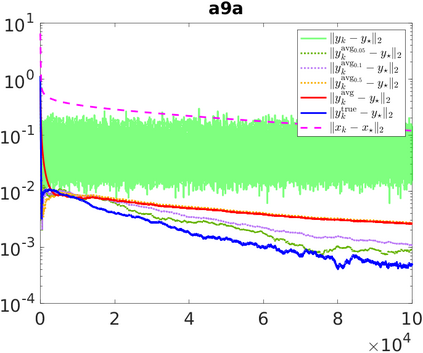
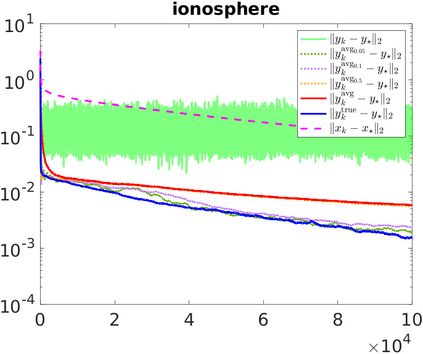
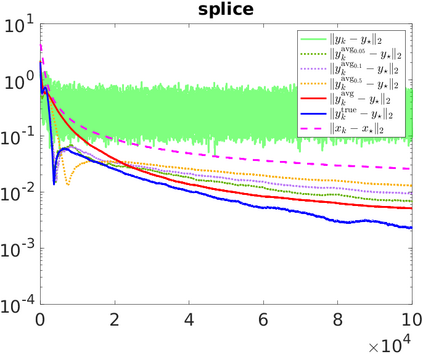
Stochastic sequential quadratic optimization (SQP) methods for solving continuous optimization problems with nonlinear equality constraints have attracted attention recently, such as for solving large-scale data-fitting problems subject to nonconvex constraints. However, for a recently proposed subclass of such methods that is built on the popular stochastic-gradient methodology from the unconstrained setting, convergence guarantees have been limited to the asymptotic convergence of the expected value of a stationarity measure to zero. This is in contrast to the unconstrained setting in which almost-sure convergence guarantees (of the gradient of the objective to zero) can be proved for stochastic-gradient-based methods. In this paper, new almost-sure convergence guarantees for the primal iterates, Lagrange multipliers, and stationarity measures generated by a stochastic SQP algorithm in this subclass of methods are proved. It is shown that the error in the Lagrange multipliers can be bounded by the distance of the primal iterate to a primal stationary point plus the error in the latest stochastic gradient estimate. It is further shown that, subject to certain assumptions, this latter error can be made to vanish by employing a running average of the Lagrange multipliers that are computed during the run of the algorithm. The results of numerical experiments are provided to demonstrate the proved theoretical guarantees.
翻译:暂无翻译