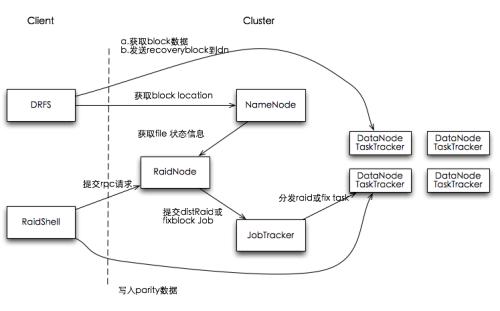
There are different ways to realize Reed Solomon (RS) codes. While in the storage community, using the generator matrices to implement RS codes is more popular, in the coding theory community the generator polynomials are typically used to realize RS codes. Prominent exceptions include HDFS-RAID, which uses generator polynomial based erasure codes, and extends the Apache Hadoop's file system. In this paper we evaluate the performance of an implementation of polynomial realization of Reed-Solomon codes, along with our optimized version of it, against that of a widely-used library (Jerasure) that implements the main matrix realization alternatives. Our experimental study shows that despite significant performance gains yielded by our optimizations, the polynomial implementations' performance is constantly inferior to those of matrix realization alternatives in general, and that of Cauchy bit matrices in particular.
翻译:实现Reed Solomon(RS)代码有不同的方式。 在储存社区,使用发电机矩阵实施Reed-Solomon(RS)代码更为流行,而在编码理论界,发电机多式计算机通常用于实现RS代码。显著的例外包括HDFS-RAID(HDFS-RAID),它使用发电机多式加密代码,扩展了Apache Hadoop的档案系统。在本文件中,我们对照一个广泛使用的图书馆(Jerasure)实施Reed-Solomon(Jerasure)实施Reed-Solomon(Solomon)代码的绩效,对照一个广泛使用的图书馆(Jerasure)实施主要矩阵实现替代工具的情况,我们实验研究表明,尽管我们的优化取得了显著的绩效收益,但多式计算机执行的性能一直低于总的矩阵实现替代工具,特别是Cauchy Bit矩阵的性能。