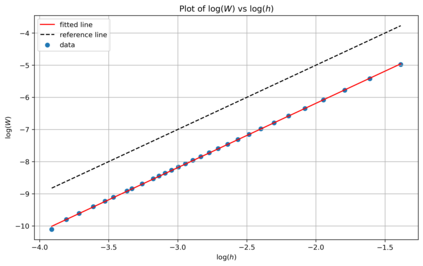
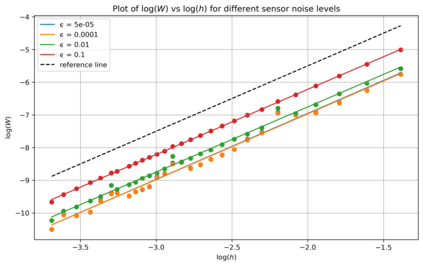
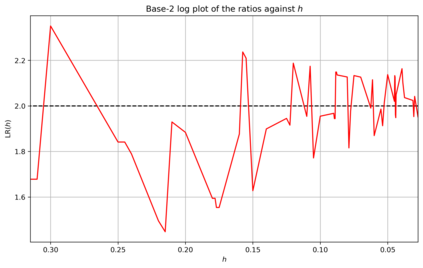
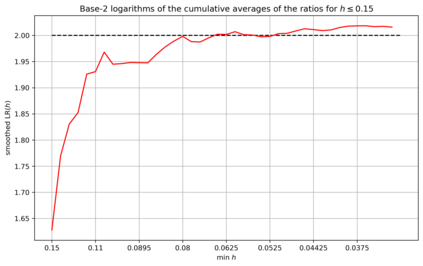
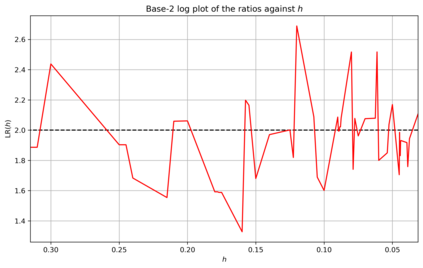
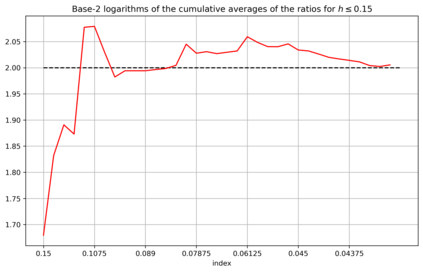
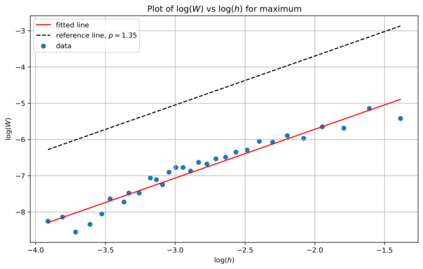
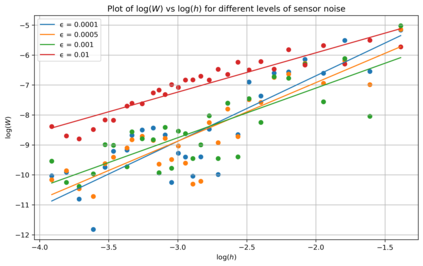
The statistical finite element method (StatFEM) is an emerging probabilistic method that allows observations of a physical system to be synthesised with the numerical solution of a PDE intended to describe it in a coherent statistical framework, to compensate for model error. This work presents a new theoretical analysis of the statistical finite element method demonstrating that it has similar convergence properties to the finite element method on which it is based. Our results constitute a bound on the Wasserstein-2 distance between the ideal prior and posterior and the StatFEM approximation thereof, and show that this distance converges at the same mesh-dependent rate as finite element solutions converge to the true solution. Several numerical examples are presented to demonstrate our theory, including an example which test the robustness of StatFEM when extended to nonlinear quantities of interest.
翻译:统计有限要素方法(StatfFEM)是一种新出现的概率方法,它使得对物理系统的观测能够与PDE的数值解决方案相结合,而PDE的数值解决方案旨在用一致的统计框架来描述它,以弥补模型错误。这项工作对统计有限要素方法进行了新的理论分析,表明它与它所依据的有限要素方法具有相似的趋同性。我们的结果构成了对Wasserstein-2距离的束缚,该距离在理想的前端和后端与SatFEM的近似之间,并表明这一距离与以真正解决方案趋同的有限要素解决方案相同,以网状依赖的速率趋近。我们提出了几个数字示例,以证明我们的理论,包括一个实例,用以测试SatFEM在扩展至非线性利益数量时的稳健性。