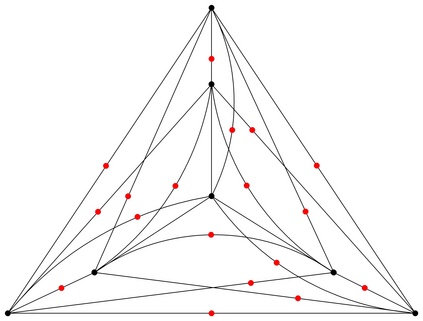
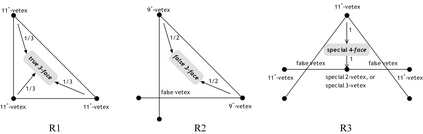
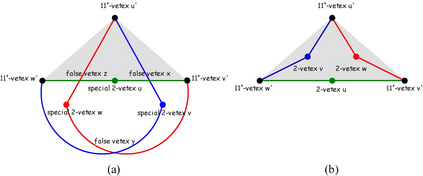
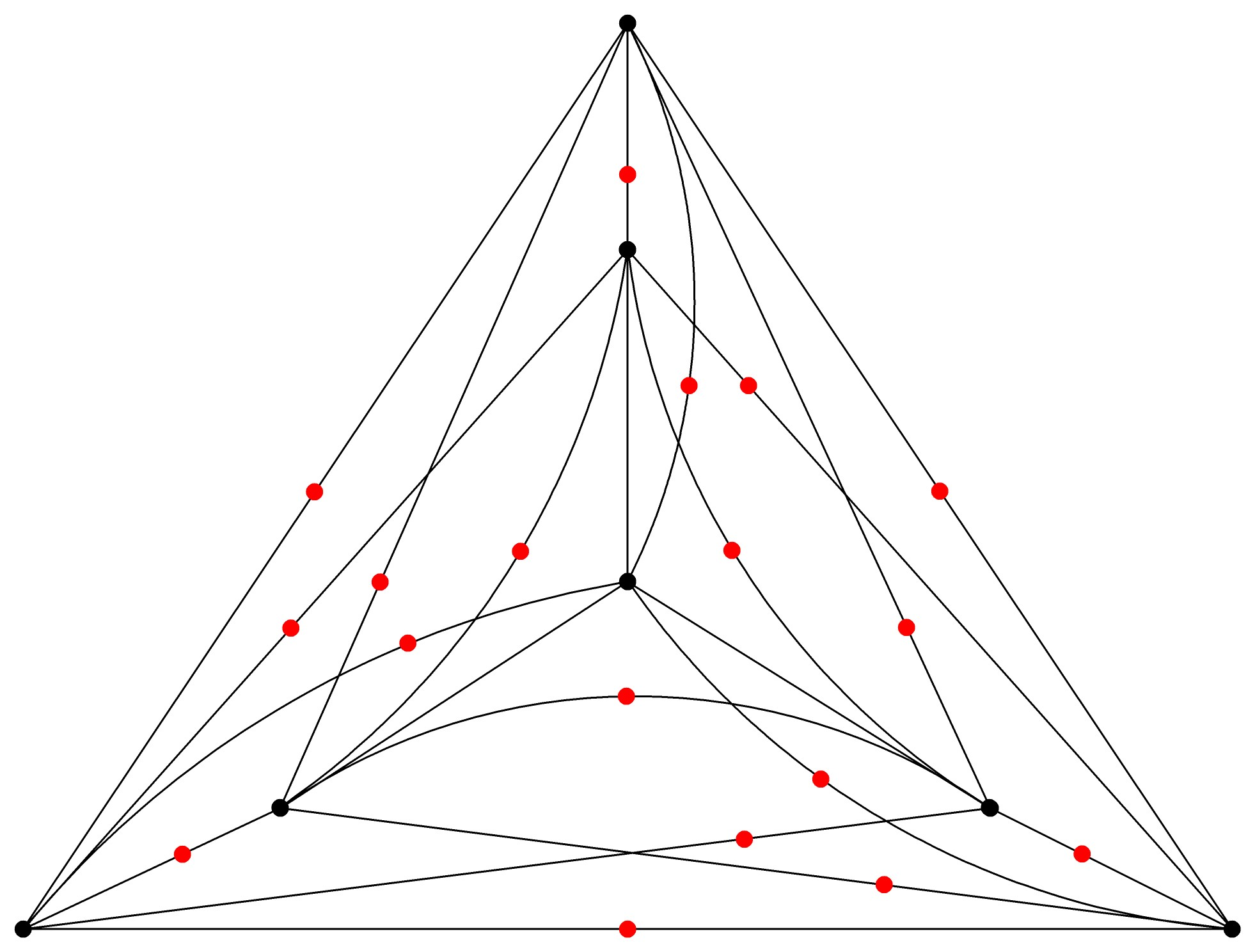
A graph is $k$-planar if it can be drawn in the plane so that each edge is crossed at most $k$ times. Typically, the class of 1-planar graphs is among the most investigated graph families within the so-called "beyond planar graphs". A dynamic $\ell$-list coloring of a graph is a proper coloring so that each vertex receives a color from a list of $\ell$ distinct candidate colors assigned to it, and meanwhile, there are at least two colors appearing in the neighborhood of every vertex of degree at least two. In this paper, we prove that each 1-planar graph has a dynamic $11$-list coloring. Moreover, we show a relationship between the dynamic coloring of 1-planar graphs and the proper coloring of 2-planar graphs, which states that the dynamic (list) chromatic number of the class of 1-planar graphs is at least the (list) chromatic number of the class of 2-planar graphs.
翻译:如果能够在平面上绘制,那么每个边缘的跨度至少为千美元。 通常, 1平面图是所谓的“ 超出平面图案” 中调查最多的图表家庭之一。 图表的动态 $ ell $ 列表颜色是一种适当的颜色, 使每个顶点从指定给它的不同候选人颜色列表中获取颜色, 同时, 每个顶点的边缘至少有两个颜色出现在每个顶点的周围。 在本文中, 我们证明每个 1 平面图有动态的 $ 11 美元 列表颜色。 此外, 我们显示了 1 平面图的动态颜色和 2 平面图的恰当颜色之间的关系, 这表明 1 平面图 类的动态( 列表) 色数至少是 2 平面图类 的( 列表) 色数 。