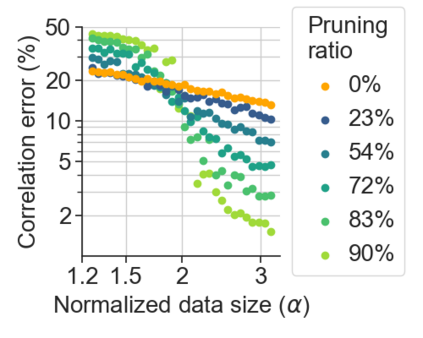
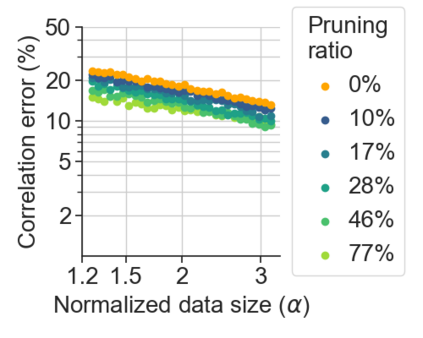
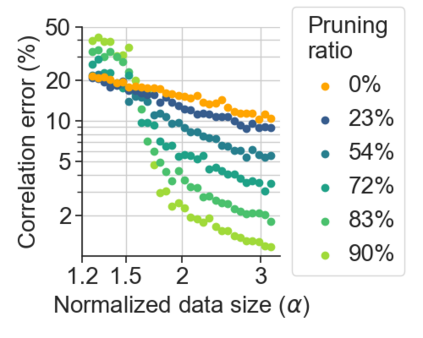
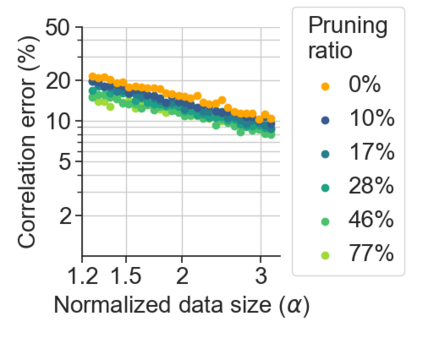
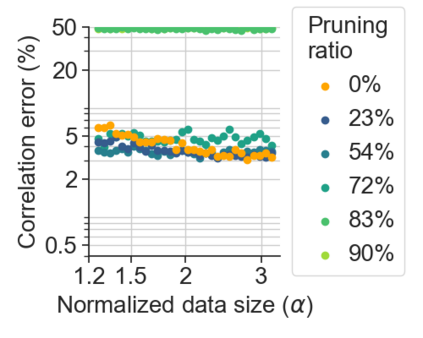
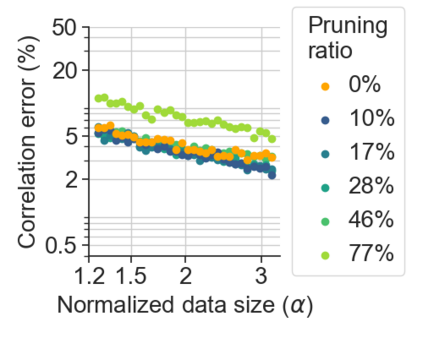
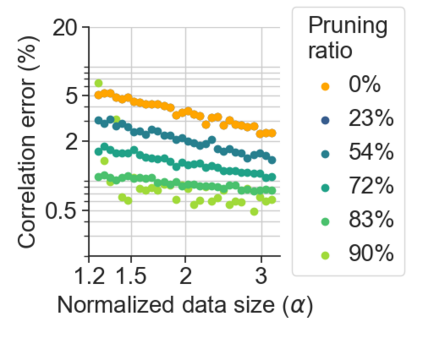
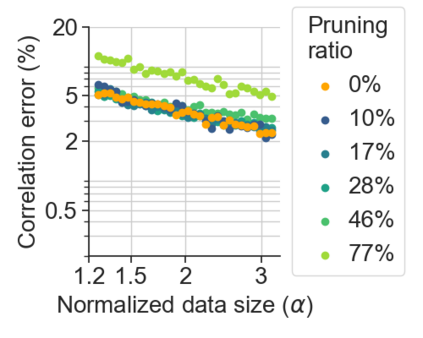
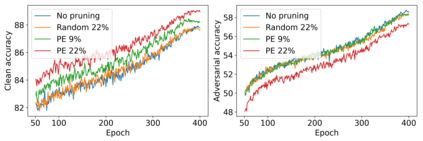
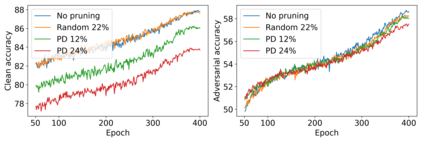
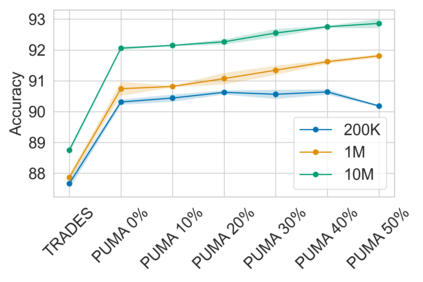
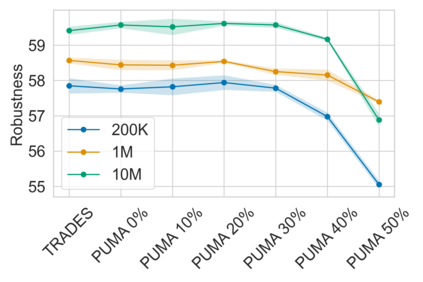
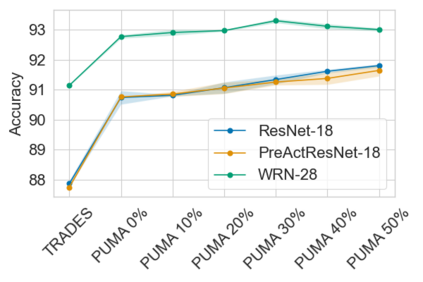
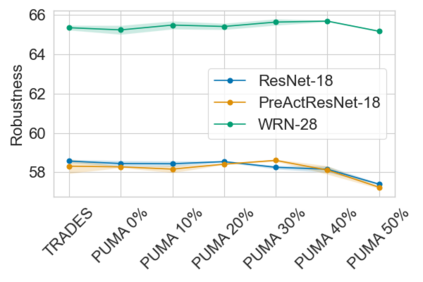
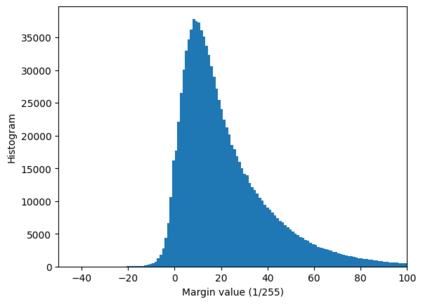
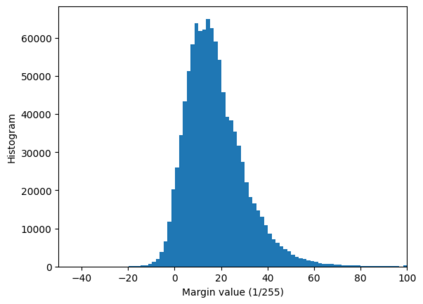
Deep learning has been able to outperform humans in terms of classification accuracy in many tasks. However, to achieve robustness to adversarial perturbations, the best methodologies require to perform adversarial training on a much larger training set that has been typically augmented using generative models (e.g., diffusion models). Our main objective in this work, is to reduce these data requirements while achieving the same or better accuracy-robustness trade-offs. We focus on data pruning, where some training samples are removed based on the distance to the model classification boundary (i.e., margin). We find that the existing approaches that prune samples with low margin fails to increase robustness when we add a lot of synthetic data, and explain this situation with a perceptron learning task. Moreover, we find that pruning high margin samples for better accuracy increases the harmful impact of mislabeled perturbed data in adversarial training, hurting both robustness and accuracy. We thus propose PUMA, a new data pruning strategy that computes the margin using DeepFool, and prunes the training samples of highest margin without hurting performance by jointly adjusting the training attack norm on the samples of lowest margin. We show that PUMA can be used on top of the current state-of-the-art methodology in robustness, and it is able to significantly improve the model performance unlike the existing data pruning strategies. Not only PUMA achieves similar robustness with less data, but it also significantly increases the model accuracy, improving the performance trade-off.
翻译:暂无翻译