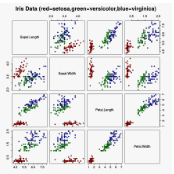
We propose a statistical framework to investigate whether a given subpopulation lies between two other subpopulations in a multivariate feature space. This methodology is motivated by a biological question from a collaborator: Is a newly discovered cell type between two known types in several given features? We propose two in-betweenness indices (IBI) to quantify the in-betweenness exhibited by a random triangle formed by the summary statistics of the three subpopulations. Statistical inference methods are provided for triangle shape and IBI metrics. The application of our methods is demonstrated in three examples: the classic Iris data set, a study of risk of relapse across three breast cancer subtypes, and the motivating neuronal cell data with measured electrophysiological features.
翻译:我们提出一个统计框架,以调查某一亚人口是否介于多变特征空间中另外两个亚人口群体之间。这个方法的动机是来自一个合作者的一个生物问题:新发现的细胞类型介于两种已知类型之间,具有几个特定特征?我们建议两个介质指数(IBI),以量化由三个亚人口组的汇总统计构成的随机三角显示的介质。提供了三角形和IBI测量尺度的统计推论方法。我们方法的应用有三个例子:典型的Iris数据集、三种乳腺癌亚型复发风险研究以及具有测测电生理特征的激励神经细胞数据。