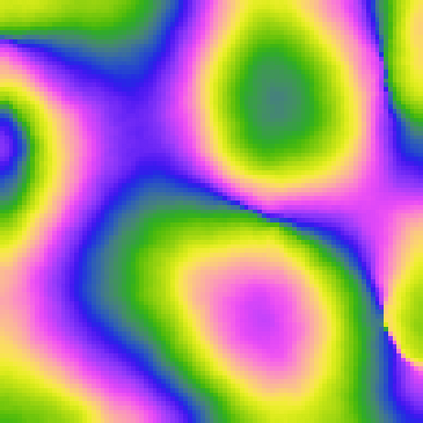
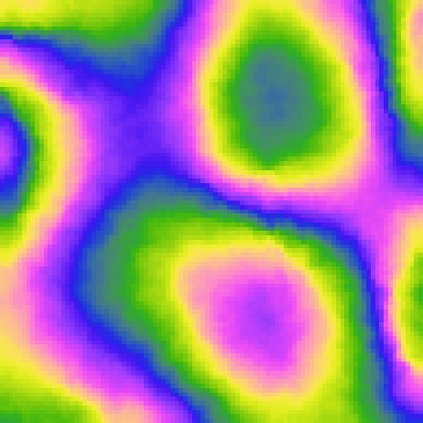
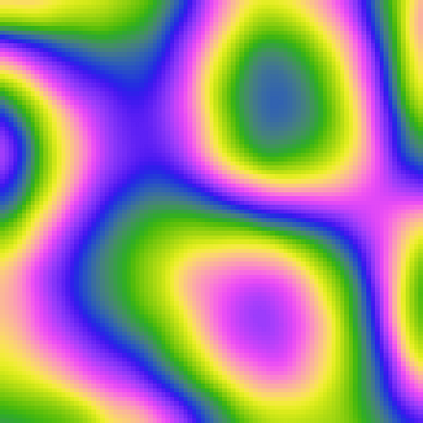
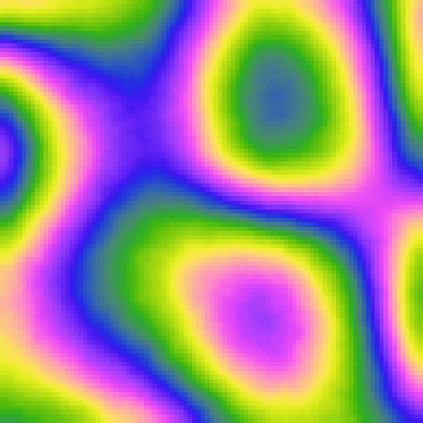
It is common to have to process signals or images whose values are cyclic and can be represented as points on the complex circle, like wrapped phases, angles, orientations, or color hues. We consider a Tikhonov-type regularization model to smoothen or interpolate circle-valued signals defined on arbitrary graphs. We propose a convex relaxation of this nonconvex problem as a semidefinite program, and an efficient algorithm to solve it.
翻译：通常需要处理具有周期性的信号或图像,这些信号或图像可以作为复杂圆圈上的点,如包绕的阶段、角度、方向或颜色。 我们认为Tikhonov型的正规化模式可以平滑或内插任意图形上定义的圆形价值信号。 我们提议将非二次曲线问题作为半无限期程序,并作为一种有效的算法来解决。
相关内容
专知会员服务
10+阅读 · 2022年3月12日
Arxiv
0+阅读 · 2022年6月10日
Arxiv
0+阅读 · 2022年6月10日
Arxiv
0+阅读 · 2022年6月9日
Arxiv
0+阅读 · 2022年6月9日
Arxiv
0+阅读 · 2022年6月8日
相关VIP内容
专知会员服务
10+阅读 · 2022年3月12日
相关资讯
相关论文
Arxiv
0+阅读 · 2022年6月10日
Arxiv
0+阅读 · 2022年6月10日
Arxiv
0+阅读 · 2022年6月9日
Arxiv
0+阅读 · 2022年6月9日
Arxiv
0+阅读 · 2022年6月8日
微信扫码咨询专知VIP会员