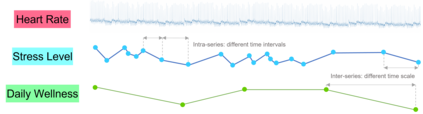
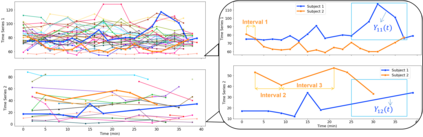
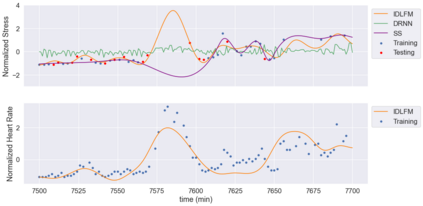
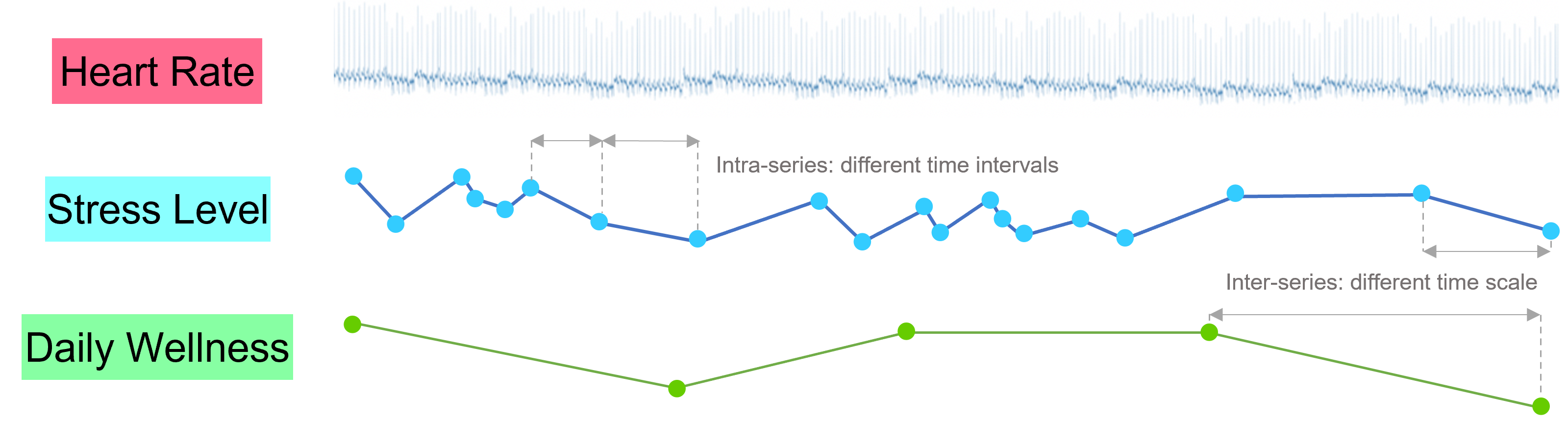
Mobile health has emerged as a major success in tracking individual health status, due to the popularity and power of smartphones and wearable devices. This has also brought great challenges in handling heterogeneous, multi-resolution data which arise ubiquitously in mobile health due to irregular multivariate measurements collected from individuals. In this paper, we propose an individualized dynamic latent factor model for irregular multi-resolution time series data to interpolate unsampled measurements of time series with low resolution. One major advantage of the proposed method is the capability to integrate multiple irregular time series and multiple subjects by mapping the multi-resolution data to the latent space. In addition, the proposed individualized dynamic latent factor model is applicable to capturing heterogeneous longitudinal information through individualized dynamic latent factors. In theory, we provide the integrated interpolation error bound of the proposed estimator and derive the convergence rate with B-spline approximation methods. Both the simulation studies and the application to smartwatch data demonstrate the superior performance of the proposed method compared to existing methods.
翻译:暂无翻译