On data-driven chance constraint learning for mixed-integer optimization problems

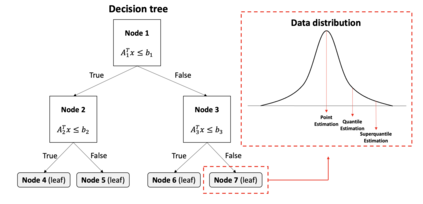
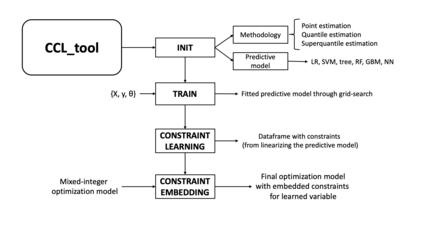
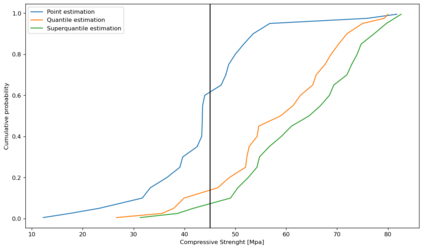
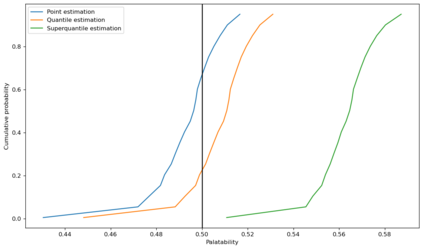
When dealing with real-world optimization problems, decision-makers usually face high levels of uncertainty associated with partial information, unknown parameters, or complex relationships between these and the problem decision variables. In this work, we develop a novel Chance Constraint Learning (CCL) methodology with a focus on mixed-integer linear optimization problems which combines ideas from the chance constraint and constraint learning literature. Chance constraints set a probabilistic confidence level for a single or a set of constraints to be fulfilled, whereas the constraint learning methodology aims to model the functional relationship between the problem variables through predictive models. One of the main issues when establishing a learned constraint arises when we need to set further bounds for its response variable: the fulfillment of these is directly related to the accuracy of the predictive model and its probabilistic behaviour. In this sense, CCL makes use of linearizable machine learning models to estimate conditional quantiles of the learned variables, providing a data-driven solution for chance constraints. An open-access software has been developed to be used by practitioners. Furthermore, benefits from CCL have been tested in two real-world case studies, proving how robustness is added to optimal solutions when probabilistic bounds are set for learned constraints.