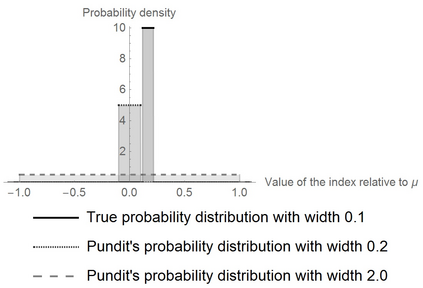
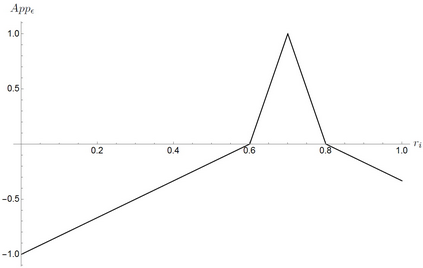
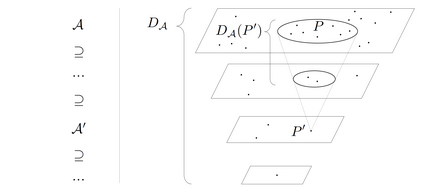
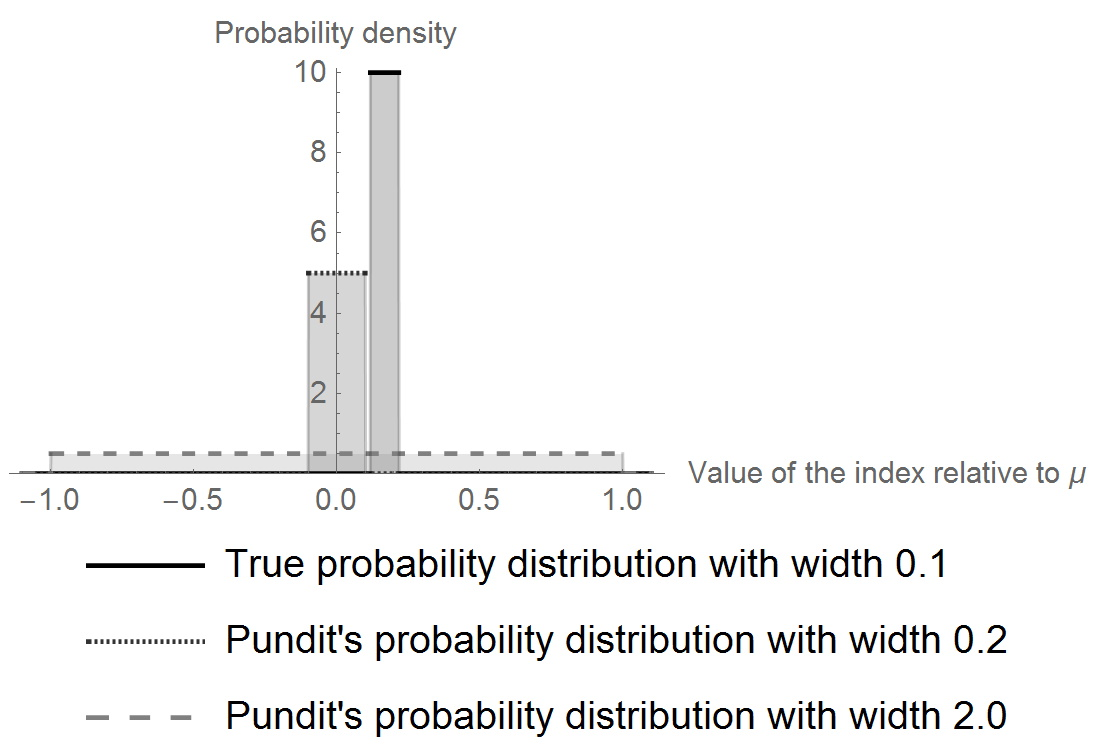
In this paper, we take a fresh look at three Popperian concepts: riskiness, falsifiability, and truthlikeness (or verisimilitude) of scientific hypotheses or theories. First, we make explicit the dimensions that underlie the notion of riskiness. Secondly, we examine if and how degrees of falsifiability can be defined, and how they are related to various dimensions of the concept of riskiness as well as the experimental context. Thirdly, we consider the relation of riskiness to (expected degrees of) truthlikeness. Throughout, we pay special attention to probabilistic theories and we offer a tentative, quantitative account of verisimilitude for probabilistic theories.
翻译:在本文中,我们重新审视了三个流行概念:科学假设或理论的风险性、可变性和真实性(或可变性),首先,我们明确了风险概念背后的维度。第二,我们审视了可变性是否和如何定义,以及这些概念与风险概念的各个方面以及实验背景的关系。第三,我们考虑了风险与(预期的)真实性之间的关系。我们始终特别关注概率理论,我们提供了概率理论的试想性、定量描述。