Commentary: analyzing binary data using MCPMod when zero counts are expected

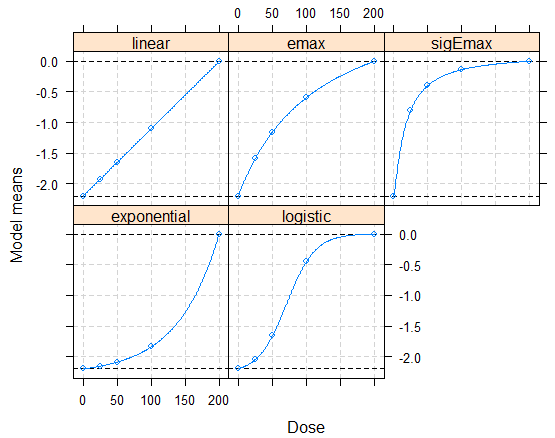
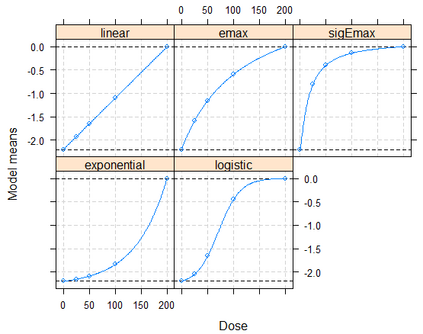
Bretz et al (2005) proposed multiple Comparison Procedure and Modeling (MCPMod) method to design and analyze dose-finding study. Pinheiro (2014) then generalized it to various types of endpoint, including but not limited to binary endpoint, survival endpoint, count data, and longitudinal data. Pinheiro (2013) recommended to use the estimated covariance matrix from the observed data to recalculate the optimal contrast and the critical value of the test For many phase II studies it is common to have small sample sizes per arm with low placebo response rates jointly. Under such circumstances, it cannot be excluded to have a zero count observed. For example, when the placebo response rate is 10%, there is about 4% chance to observe zero responders in the placebo group, or other dose group(s), which has a similar response rate as placebo. In this manuscript, we would like to illustrate the potential problem of Pinheiro (2013) using a case study and simulations. An alternative method using Firth's logistic regression was evaluated to get a stable estimate of response for each dose group. In addition, we evaluated two options to address the issue with problematic contrast coefficients.